How To Find Final Kinetic Energy
Kalali
Mar 26, 2025 · 6 min read
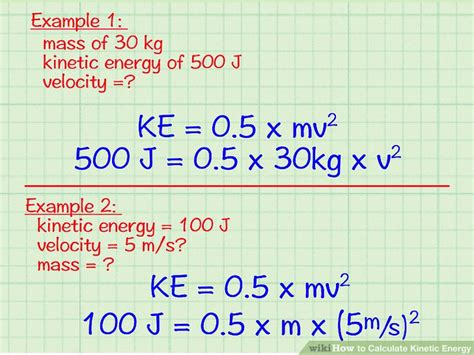
Table of Contents
How to Find Final Kinetic Energy: A Comprehensive Guide
Determining final kinetic energy is a fundamental concept in physics, particularly within the realm of mechanics and energy conservation. Understanding how to calculate it is crucial for solving numerous problems related to motion, collisions, and energy transfer. This comprehensive guide will delve into the various methods and scenarios involved in finding the final kinetic energy of an object or system.
Understanding Kinetic Energy
Before we explore how to find the final kinetic energy, let's revisit the definition of kinetic energy itself. Kinetic energy is the energy an object possesses due to its motion. It's directly proportional to the mass and the square of the velocity of the object. Mathematically, it's represented as:
KE = 1/2 * m * v²
Where:
- KE represents kinetic energy (usually measured in Joules)
- m represents the mass of the object (usually measured in kilograms)
- v represents the velocity of the object (usually measured in meters per second)
This simple formula forms the foundation for all our calculations. The key to finding the final kinetic energy is to understand the situation and identify the final velocity of the object.
Methods for Finding Final Kinetic Energy
The approach to finding final kinetic energy depends heavily on the context of the problem. Here are some common scenarios and the methods used to solve them:
1. Using Constant Acceleration
In situations involving constant acceleration, we can utilize kinematic equations to determine the final velocity, and subsequently, the final kinetic energy. Let's assume an object starts with an initial velocity (vᵢ) and undergoes constant acceleration (a) over a displacement (d). We can use the following kinematic equation:
vƒ² = vᵢ² + 2ad
Where:
- vƒ represents the final velocity
Once we've calculated the final velocity (vƒ) using this equation, we can plug it into the kinetic energy formula (KE = 1/2 * m * vƒ²) to find the final kinetic energy.
Example: A 2kg object starts from rest (vᵢ = 0 m/s) and accelerates at 5 m/s² over a distance of 10 meters. Find its final kinetic energy.
- Find the final velocity: vƒ² = 0² + 2 * 5 m/s² * 10 m = 100 m²/s² => vƒ = 10 m/s
- Calculate the final kinetic energy: KE = 1/2 * 2 kg * (10 m/s)² = 100 J
Therefore, the final kinetic energy of the object is 100 Joules.
2. Using Conservation of Energy
The principle of conservation of energy states that energy cannot be created or destroyed, only transformed from one form to another. This principle is particularly useful when dealing with systems where energy changes between potential energy (PE) and kinetic energy (KE). The total mechanical energy (TME) of a system remains constant, assuming no energy loss due to friction or other non-conservative forces.
TME = KE + PE
If we know the initial kinetic energy and potential energy, and the final potential energy, we can find the final kinetic energy:
KEƒ = TME - PEƒ = (KEᵢ + PEᵢ) - PEƒ
Where:
- KEᵢ and PEᵢ represent initial kinetic and potential energy respectively
- KEƒ and PEƒ represent final kinetic and potential energy respectively
Example: A 1 kg ball is dropped from a height of 5 meters. Find its final kinetic energy just before it hits the ground (ignoring air resistance).
- Initial energy: The ball starts with no kinetic energy (KEᵢ = 0 J) and potential energy PEᵢ = mgh = 1 kg * 9.8 m/s² * 5 m = 49 J
- Final potential energy: Just before impact, the potential energy is 0 J (PEƒ = 0 J).
- Final kinetic energy: KEƒ = (0 J + 49 J) - 0 J = 49 J
Therefore, the ball's final kinetic energy is 49 Joules.
3. Analyzing Collisions
Collisions present more complex scenarios. The final kinetic energy depends on the type of collision:
-
Elastic Collisions: In perfectly elastic collisions, both momentum and kinetic energy are conserved. This means the total kinetic energy before the collision equals the total kinetic energy after the collision. Solving for final kinetic energy requires using conservation of momentum equations alongside the conservation of kinetic energy.
-
Inelastic Collisions: In inelastic collisions, momentum is conserved, but kinetic energy is not. Some kinetic energy is lost as heat, sound, or deformation. The final kinetic energy will be less than the initial kinetic energy. You still use conservation of momentum to find final velocities, but you cannot use conservation of kinetic energy directly.
Example (Inelastic Collision): Two objects of equal mass (m) collide inelastically. Object 1 has an initial velocity of 10 m/s, and object 2 is stationary. After the collision, they stick together. Find their final kinetic energy.
- Conservation of momentum: m * 10 m/s + m * 0 m/s = 2m * vƒ => vƒ = 5 m/s
- Final kinetic energy: KEƒ = 1/2 * (2m) * (5 m/s)² = 25m J
Note that we cannot determine the exact final kinetic energy without knowing the mass (m). However, we have expressed it in terms of the mass.
4. Using Work-Energy Theorem
The work-energy theorem states that the net work done on an object is equal to the change in its kinetic energy:
Wnet = ΔKE = KEƒ - KEᵢ
This is particularly useful when forces act on an object over a distance. If we know the net work done, and the initial kinetic energy, we can calculate the final kinetic energy:
KEƒ = Wnet + KEᵢ
Example: A 5 kg object is pushed with a constant force of 20 N over a distance of 4 meters. If it starts from rest, what is its final kinetic energy?
- Net work done: Wnet = Fd = 20 N * 4 m = 80 J
- Initial kinetic energy: KEᵢ = 0 J (starts from rest)
- Final kinetic energy: KEƒ = 80 J + 0 J = 80 J
Therefore, the final kinetic energy is 80 Joules.
Advanced Scenarios and Considerations
Several factors can complicate finding final kinetic energy:
-
Friction: Friction is a non-conservative force that converts kinetic energy into heat. In real-world scenarios, friction must be accounted for, usually by including work done by friction in the work-energy theorem or by considering energy loss.
-
Rotating Objects: For rotating objects, we must consider rotational kinetic energy in addition to translational kinetic energy. The total kinetic energy is the sum of both.
-
Multiple Objects and Systems: Systems involving multiple objects require applying the principles of conservation of momentum and energy for each object and then considering the combined system.
Conclusion
Finding the final kinetic energy involves a nuanced approach depending on the specific problem. Understanding the fundamental principles of kinetic energy, conservation of energy, the work-energy theorem, and the nuances of different types of collisions is essential. By carefully considering the given information and selecting the appropriate method, you can accurately determine the final kinetic energy of an object or system in various physical situations. Remember to always pay close attention to units and to properly account for any external forces, especially friction, that may influence the energy transfer. Mastering these concepts provides a solid foundation for tackling more advanced problems in physics and engineering.
Latest Posts
Latest Posts
-
1500 Ml Is How Many Ounces
Mar 29, 2025
-
What Is 27 Degrees Centigrade In Fahrenheit
Mar 29, 2025
-
How Many Ounces Is One Cup Of Butter
Mar 29, 2025
-
How Many Feet In 42 Inches
Mar 29, 2025
-
Is H20 On The Periodic Table
Mar 29, 2025
Related Post
Thank you for visiting our website which covers about How To Find Final Kinetic Energy . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
