Multiplication And Division Of Complex Numbers
Kalali
Apr 03, 2025 · 5 min read
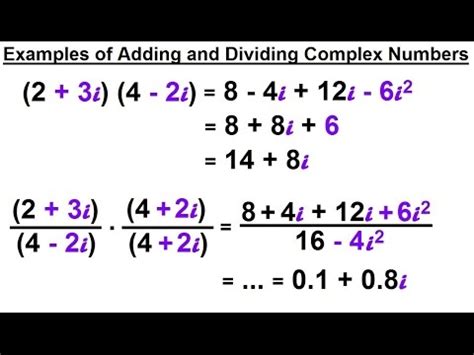
Table of Contents
Multiplication and Division of Complex Numbers: A Comprehensive Guide
Complex numbers, an extension of real numbers incorporating the imaginary unit i (where i² = -1), open up a fascinating world of mathematical possibilities. While addition and subtraction are relatively straightforward, multiplication and division of complex numbers require a deeper understanding of their properties. This comprehensive guide will delve into the mechanics, applications, and underlying principles of these operations, equipping you with a solid grasp of this crucial aspect of complex number theory.
Understanding Complex Numbers
Before diving into the operations, let's establish a firm foundation. A complex number z is generally represented as:
z = a + bi
Where:
- a is the real part (Re(z))
- b is the imaginary part (Im(z))
- i is the imaginary unit (√-1)
These numbers can be visualized on a complex plane (Argand diagram), where the real part is plotted on the x-axis and the imaginary part on the y-axis. This geometric representation offers valuable insights into the operations we'll explore.
Multiplication of Complex Numbers
Multiplying complex numbers involves applying the distributive property (FOIL method) and remembering that i² = -1. Let's multiply two complex numbers, z₁ = a + bi and z₂ = c + di:
z₁ * z₂ = (a + bi)(c + di)
Applying the distributive property:
= a(c + di) + bi(c + di)
= ac + adi + bci + bdi²
Since i² = -1:
= ac + adi + bci - bd
Rearranging to separate the real and imaginary parts:
= (ac - bd) + (ad + bc)i
Therefore, the product of two complex numbers is another complex number with a real part (ac - bd) and an imaginary part (ad + bc).
Examples of Complex Number Multiplication
Let's illustrate with some examples:
Example 1: (2 + 3i)(4 - i)
Following the formula:
- a = 2, b = 3
- c = 4, d = -1
(2 + 3i)(4 - i) = (24 - 3-1) + (2*-1 + 3*4)i = (8 + 3) + (-2 + 12)i = 11 + 10i
Example 2: (-1 + 2i)(3 + 2i)
- a = -1, b = 2
- c = 3, d = 2
(-1 + 2i)(3 + 2i) = (-13 - 22) + (-12 + 23)i = (-3 - 4) + (-2 + 6)i = -7 + 4i
Example 3: (5i)(2 + 3i)
Here, the real part of the first complex number is 0.
- a = 0, b = 5
- c = 2, d = 3
(5i)(2 + 3i) = (02 - 53) + (03 + 52)i = -15 + 10i
These examples demonstrate the systematic application of the distributive property and the substitution of i² with -1.
Division of Complex Numbers
Dividing complex numbers is slightly more involved. We achieve this by multiplying both the numerator and denominator by the complex conjugate of the denominator. The complex conjugate of a complex number a + bi is a - bi. Multiplying a complex number by its conjugate results in a real number.
Let's divide z₁ = a + bi by z₂ = c + di:
(a + bi) / (c + di)
Multiplying the numerator and denominator by the conjugate of the denominator (c - di):
= (a + bi)(c - di) / (c + di)(c - di)
Expanding the numerator and denominator:
= (ac + bd) + (bc - ad)i / (c² + d²)
Separating into real and imaginary parts:
= (ac + bd)/(c² + d²) + (bc - ad)/(c² + d²)i
The result is again a complex number.
Examples of Complex Number Division
Example 1: (3 + 2i) / (1 + i)
The conjugate of the denominator (1 + i) is (1 - i).
(3 + 2i) / (1 + i) * (1 - i) / (1 - i) = (3 - 3i + 2i - 2i²) / (1 - i² ) = (3 - i + 2) / (1 + 1) = (5 - i) / 2 = 5/2 - i/2 or 2.5 - 0.5i
Example 2: (4 - 3i) / (2 - 2i)
The conjugate of (2 - 2i) is (2 + 2i).
(4 - 3i) / (2 - 2i) * (2 + 2i) / (2 + 2i) = (8 + 8i - 6i - 6i²) / (4 - 4i²) = (8 + 2i + 6) / (4 + 4) = (14 + 2i) / 8 = 7/4 + i/4 or 1.75 + 0.25i
Example 3: (5i) / (3 - i)
The conjugate of (3 - i) is (3 + i).
(5i) / (3 - i) * (3 + i) / (3 + i) = (15i + 5i²) / (9 - i²) = (-5 + 15i) / 10 = -1/2 + 3i/2 or -0.5 + 1.5i
These examples illustrate the process of rationalizing the denominator using the complex conjugate to obtain a final answer in the standard a + bi format.
Geometric Interpretation of Multiplication and Division
The complex plane provides a powerful visual aid. Multiplication by a complex number can be interpreted as a rotation and scaling. The magnitude (or modulus) of the complex number determines the scaling factor, and the argument (or angle) determines the rotation. Division is essentially the reverse process – a scaling and rotation in the opposite direction.
Applications of Complex Number Arithmetic
The multiplication and division of complex numbers are not merely theoretical exercises; they find practical applications in various fields:
-
Electrical Engineering: Analyzing alternating current circuits, impedance calculations, and signal processing heavily rely on complex number arithmetic.
-
Quantum Mechanics: Complex numbers are fundamental in describing quantum states and wave functions.
-
Signal Processing: Manipulating signals using Fourier transforms involves extensive use of complex number operations.
-
Fractals: The generation of intricate fractal patterns, such as the Mandelbrot set, depends on iterative complex number calculations.
-
Fluid Dynamics: Complex analysis techniques are used to solve certain fluid flow problems.
Conclusion
Mastering multiplication and division of complex numbers is crucial for anyone working with advanced mathematics, engineering, or physics. Understanding the underlying principles, practicing the techniques, and appreciating the geometric interpretation will unlock a deeper understanding of these fascinating numbers and their wide-ranging applications. The examples provided offer a strong foundation for further exploration and application in various fields. Consistent practice and a focus on understanding the fundamental concepts are key to achieving proficiency in this area.
Latest Posts
Latest Posts
-
How Many Phosphate Groups Does Atp Have
Apr 03, 2025
-
What Is 300 F In C
Apr 03, 2025
-
Convert From Rectangular To Polar Equation
Apr 03, 2025
-
Which Of The Following Figures Has Reflectional Symmetry
Apr 03, 2025
-
Zeke Is Racing His Little Brother
Apr 03, 2025
Related Post
Thank you for visiting our website which covers about Multiplication And Division Of Complex Numbers . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
