Convert From Rectangular To Polar Equation
Kalali
Apr 03, 2025 · 5 min read
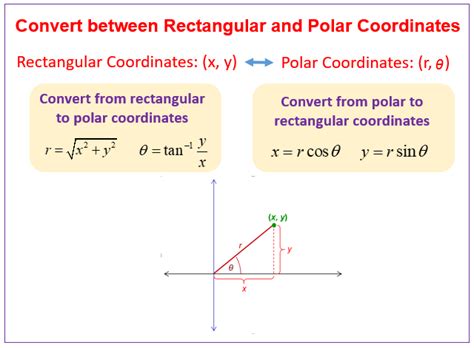
Table of Contents
Converting Rectangular to Polar Equations: A Comprehensive Guide
Converting equations between rectangular (Cartesian) and polar coordinate systems is a fundamental concept in mathematics, particularly useful in calculus, physics, and engineering. Understanding this conversion allows for a more efficient representation and solution of certain problems. This comprehensive guide will delve into the intricacies of converting rectangular equations to their polar counterparts, providing a step-by-step process, examples, and helpful tips to master this crucial skill.
Understanding Coordinate Systems
Before we dive into the conversion process, let's refresh our understanding of both coordinate systems:
Rectangular Coordinates (Cartesian Coordinates)
Rectangular coordinates represent a point in a plane using its horizontal (x) and vertical (y) distances from the origin (0, 0). These distances are perpendicular to each other.
Polar Coordinates
Polar coordinates represent a point in a plane using its distance (r) from the origin and the angle (θ) it makes with the positive x-axis, measured counterclockwise.
The relationship between these two systems is the key to conversion.
The Conversion Formulas: The Bridge Between Systems
The core of converting between rectangular and polar equations lies in the following fundamental relationships:
- x = r cos θ
- y = r sin θ
- r² = x² + y²
- tan θ = y/x (Note: This formula has limitations; we'll discuss this later)
These formulas are derived directly from trigonometry. Imagine a right-angled triangle formed by the point (x, y), the origin (0, 0), and the projection of the point onto the x-axis. The hypotenuse of this triangle is 'r', the adjacent side is 'x', and the opposite side is 'y'. Applying basic trigonometric functions (sine, cosine, and tangent) gives us the above relationships.
Step-by-Step Guide to Conversion
Converting a rectangular equation to its polar equivalent involves substituting the rectangular coordinates (x and y) with their polar counterparts (r and θ) using the formulas above. The process isn't always straightforward and often requires manipulation and simplification. Let's break down the process into manageable steps:
Step 1: Identify the Rectangular Equation:
Begin with the rectangular equation you want to convert. For example, let's consider the equation of a circle: x² + y² = a²
Step 2: Substitute Using the Conversion Formulas:
Substitute 'x' and 'y' with their polar equivalents (r cos θ and r sin θ, respectively). In our circle example:
(r cos θ)² + (r sin θ)² = a²
Step 3: Simplify and Solve for 'r' (or θ):
Simplify the equation using trigonometric identities and algebraic manipulation. In our circle example:
r²(cos²θ + sin²θ) = a²
Since cos²θ + sin²θ = 1 (a fundamental trigonometric identity), the equation simplifies to:
r² = a²
Therefore, the polar equation of a circle with radius 'a' is simply r = a. Note that this equation is independent of θ, indicating that the radius remains constant regardless of the angle.
Step 4: Consider Special Cases and Limitations:
Some equations require more complex manipulation. Also, remember the limitation of tan θ = y/x. This formula only provides the angle in the correct quadrant if both x and y are positive or negative. Otherwise, you must carefully consider the signs of x and y to determine the correct quadrant for θ.
Let's consider another example: the equation of a line y = mx + c.
Substituting the polar equivalents:
r sin θ = m(r cos θ) + c
This equation can be rearranged to solve for 'r':
r(sin θ - m cos θ) = c
r = c / (sin θ - m cos θ)
This is the polar equation of a straight line.
Advanced Examples and Techniques
Let's explore some more complex examples to demonstrate the versatility and power of the conversion process:
Example 1: Converting a Parabola
Consider the parabola: y² = 4ax. Substituting the polar equivalents:
(r sin θ)² = 4a(r cos θ)
r² sin²θ = 4ar cos θ
Assuming r ≠ 0 (excluding the origin), we can divide both sides by 'r':
r sin²θ = 4a cos θ
r = 4a cos θ / sin²θ
This equation represents the polar form of the parabola.
Example 2: Dealing with Equations Involving Both x² and y²
Consider the equation: x² - y² = 1. Substituting the polar equivalents:
(r cos θ)² - (r sin θ)² = 1
r²(cos²θ - sin²θ) = 1
r² = 1 / (cos²θ - sin²θ)
r = ± 1 / √(cos²θ - sin²θ)
This represents a hyperbola in polar coordinates. Notice the ± sign, indicating two branches of the hyperbola.
Example 3: Handling Cases with Undefined Tangent
If an equation involves a term like x = 0, which renders the tan θ = y/x formula undefined, you might need a different approach. Instead, focus on the relationship between x, y, and r:
If x = 0, the point lies on the y-axis. In polar coordinates, this means θ = π/2 or θ = 3π/2.
Tips and Tricks for Successful Conversion
- Master Trigonometric Identities: A solid grasp of trigonometric identities is crucial for simplifying the equations after substitution.
- Algebraic Manipulation: Proficiency in algebraic manipulation will help you solve for 'r' (or θ) effectively.
- Visualize the Graph: Sketching the graph of the rectangular equation can provide valuable insights into the expected shape and characteristics of the polar equation, aiding in verification and error detection.
- Check for Symmetry: Recognizing symmetry in the rectangular equation can simplify the conversion process and lead to more concise polar forms.
- Handle Special Cases Carefully: Pay close attention to cases where 'r' or 'θ' might be undefined or have limited ranges.
Conclusion
Converting rectangular equations to polar form is a valuable skill in mathematics and related fields. By understanding the fundamental conversion formulas and applying systematic steps, you can effectively transform equations between these coordinate systems. This process opens up avenues for simplifying complex equations, solving problems more efficiently, and gaining a deeper understanding of geometric shapes and their representations. Remember to practice regularly with diverse examples to solidify your understanding and master this crucial technique. The more you practice, the more intuitive and efficient the conversion process will become.
Latest Posts
Latest Posts
-
31 Out Of 35 As A Percentage
Apr 04, 2025
-
How Many Inches Is 120 Centimeters
Apr 04, 2025
-
What Percent Of 40 Is 24
Apr 04, 2025
-
What Percent Is 2 Of 15
Apr 04, 2025
-
How Many Cups Are In 64 Fluid Ounces
Apr 04, 2025
Related Post
Thank you for visiting our website which covers about Convert From Rectangular To Polar Equation . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
