Percentage Of 2 Out Of 6
Kalali
Apr 09, 2025 · 5 min read
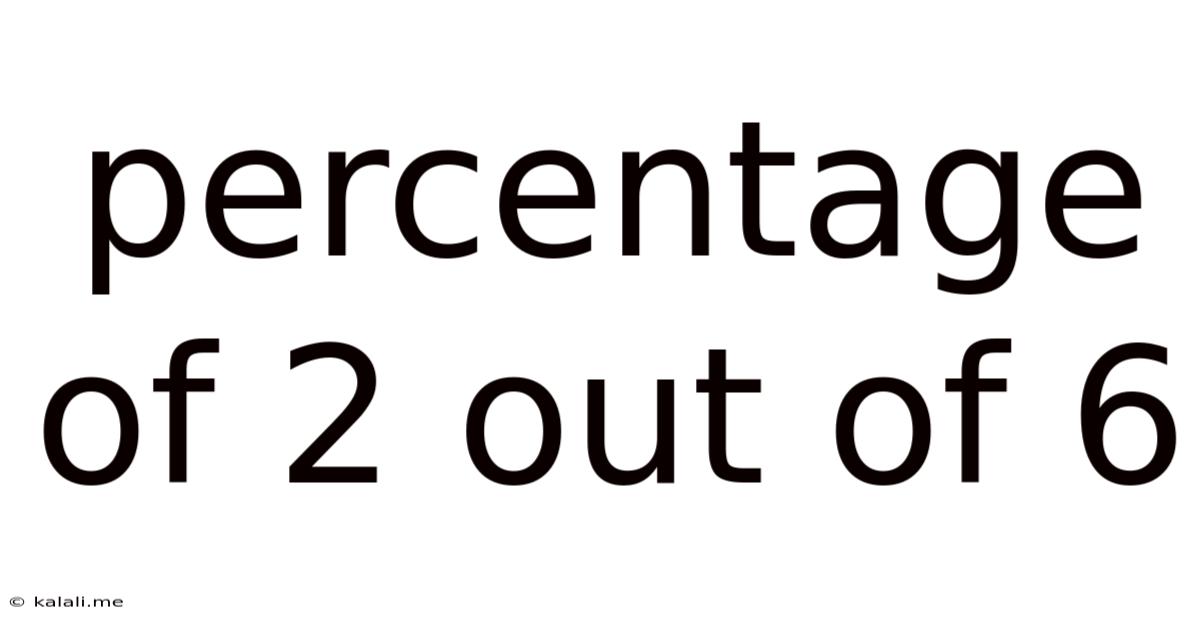
Table of Contents
Understanding Percentages: A Deep Dive into "2 out of 6"
Calculating percentages is a fundamental skill applicable across numerous fields, from everyday budgeting and shopping to complex statistical analysis and scientific research. This article delves into the seemingly simple question: "What percentage is 2 out of 6?" We'll not only solve this specific problem but explore the underlying concepts, offer multiple calculation methods, and provide practical applications to enhance your understanding of percentages. This comprehensive guide will empower you to tackle similar percentage calculations with confidence.
Meta Description: Learn how to calculate percentages effectively. This detailed guide explains how to determine the percentage represented by 2 out of 6, exploring various methods and real-world applications. Master percentage calculations and boost your math skills!
What does "2 out of 6" represent?
Before diving into calculations, let's clarify what "2 out of 6" signifies. It represents a ratio or a fraction. It tells us that there are 2 instances of a particular event or characteristic out of a total of 6 possible instances. This could represent anything from 2 successful attempts out of 6 tries to 2 red marbles out of a total of 6 marbles in a bag. The key is understanding the relationship between the part (2) and the whole (6).
Method 1: The Basic Formula
The most straightforward method to calculate the percentage involves using the basic percentage formula:
(Part / Whole) * 100% = Percentage
In our case:
(2 / 6) * 100% = Percentage
First, we divide the part (2) by the whole (6):
2 / 6 = 0.3333... (This is a recurring decimal)
Then, we multiply the result by 100% to express it as a percentage:
0.3333... * 100% ≈ 33.33%
Therefore, 2 out of 6 represents approximately 33.33%. The use of the approximately symbol (~) is because we are dealing with a recurring decimal, and it is often rounded to two decimal places for practical purposes.
Method 2: Simplifying the Fraction
Before applying the percentage formula, we can simplify the fraction 2/6. Both the numerator (2) and the denominator (6) are divisible by 2:
2 / 2 = 1 6 / 2 = 3
This simplifies the fraction to 1/3. Now we can apply the formula:
(1 / 3) * 100% = Percentage
1 / 3 ≈ 0.3333...
0.3333... * 100% ≈ 33.33%
This method demonstrates that simplifying the fraction before calculation can sometimes make the process easier, especially with larger numbers.
Method 3: Using Proportions
Another approach involves setting up a proportion. We can express the problem as:
2/6 = x/100
Where 'x' represents the percentage we want to find. To solve for 'x', we cross-multiply:
6x = 200
Then, divide both sides by 6:
x = 200 / 6 ≈ 33.33
Therefore, x ≈ 33.33%, confirming our previous results.
Understanding Recurring Decimals and Rounding
The result of 2/6, or 1/3, is a recurring decimal (0.333...). This means the digit 3 repeats infinitely. In practical applications, it's necessary to round the decimal to a certain number of decimal places. Common practice is to round to two decimal places (33.33%), although the context might dictate rounding to a different degree of accuracy. For example, in financial calculations, rounding to the nearest cent might be necessary.
Real-World Applications of Percentage Calculations
The ability to calculate percentages is essential in a wide range of scenarios. Here are a few examples:
-
Sales and Discounts: Calculating discounts, sales tax, or profit margins. For example, if an item is discounted by 33.33%, understanding this calculation helps you determine the final price.
-
Grades and Scores: Converting raw scores (like the number of correct answers on a test) into percentages. For instance, if you answered 2 questions correctly out of 6, your score is 33.33%.
-
Data Analysis and Statistics: Representing data proportions as percentages for easier interpretation and comparison. In surveys or polls, percentages are used extensively to summarize findings.
-
Finance: Calculating interest rates, returns on investments, or budgeting proportions. Knowing percentage calculations is crucial for managing personal finances effectively.
-
Science and Engineering: Expressing experimental results, error margins, or efficiencies as percentages.
-
Cooking and Baking: Scaling recipes up or down based on percentages. If a recipe calls for 2 out of 6 cups of flour and you want to double the recipe, you'd need to calculate the percentage increase for other ingredients as well.
Beyond the Basics: More Complex Percentage Problems
While "2 out of 6" is a relatively simple calculation, the principles can be extended to more complex scenarios. Consider these examples:
-
Finding the original amount: If an item is discounted by 33.33% and the final price is $10, what was the original price?
-
Calculating percentage change: If the number of sales increased from 2 to 6, what is the percentage increase?
-
Calculating percentage points: Differentiating between percentage change and percentage points is crucial for accurate interpretation of data.
-
Compound interest: Calculating the total amount after interest is compounded over several periods.
Mastering Percentage Calculations: Tips and Tricks
-
Practice Regularly: The best way to master percentage calculations is through consistent practice. Try solving various problems of increasing complexity.
-
Understand the Concepts: Focus on understanding the underlying concepts of ratios, fractions, and proportions.
-
Utilize Different Methods: Become familiar with various calculation methods, choosing the one that suits the problem best.
-
Use Technology Wisely: Calculators and spreadsheets can be helpful tools for more complex calculations. However, remember to understand the underlying process even when using technology.
-
Check Your Answers: Always double-check your answers to ensure accuracy.
In conclusion, understanding how to calculate percentages, even for seemingly simple problems like "2 out of 6," is a valuable skill with wide-ranging applications. By mastering these fundamental concepts and employing various calculation methods, you'll be well-equipped to tackle more complex percentage problems and confidently apply this knowledge in various real-world situations. Remember the key formula: (Part/Whole) * 100% = Percentage, and practice regularly to build your confidence and proficiency.
Latest Posts
Latest Posts
-
What Is 17 Degrees In Celsius
Apr 17, 2025
-
What Percentage Of 45 Is 3
Apr 17, 2025
-
What Is 16 Out Of 20 As A Percentage
Apr 17, 2025
-
How Many Cups Equal 24 Ounces
Apr 17, 2025
-
What Is The Most Reactive Nonmetal
Apr 17, 2025
Related Post
Thank you for visiting our website which covers about Percentage Of 2 Out Of 6 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.