Range Of Stem And Leaf Plot
Kalali
Apr 04, 2025 · 6 min read
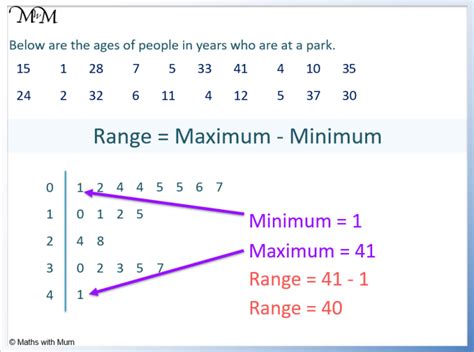
Table of Contents
Understanding the Range in Stem and Leaf Plots: A Comprehensive Guide
Stem and leaf plots, also known as stem-and-leaf diagrams, are a valuable tool in descriptive statistics. They provide a simple yet effective way to display data, revealing its distribution and key characteristics, including the range. This comprehensive guide will delve deep into the concept of range within the context of stem and leaf plots, explaining its calculation, interpretation, and significance in data analysis.
What is a Stem and Leaf Plot?
Before discussing the range, let's briefly revisit the basics of stem and leaf plots. A stem and leaf plot is a visual representation of data that organizes numerical values into "stems" and "leaves." The stem represents the leading digit(s) of the data points, while the leaf represents the trailing digit(s). This arrangement allows for a quick understanding of data distribution, including identifying clusters, outliers, and the overall spread of the data.
Example:
Consider the following dataset representing the scores of 15 students on a test:
78, 82, 85, 91, 92, 95, 75, 88, 89, 93, 72, 80, 90, 87, 94
A stem and leaf plot for this data would look like this:
Stem | Leaf
-------
7 | 2 5 8
8 | 0 2 5 7 8 9
9 | 0 1 2 3 4 5
Here, the tens digit forms the stem, and the units digit forms the leaf. This visualization immediately shows the distribution of scores, with a concentration in the 80s and 90s.
What is the Range?
The range is a fundamental measure of dispersion in statistics. It represents the difference between the highest and lowest values in a dataset. A larger range indicates greater variability or spread within the data, while a smaller range suggests that the data points are more closely clustered together. Understanding the range provides crucial insights into the overall variability of a dataset.
Formula:
The range is calculated using the simple formula:
Range = Maximum Value - Minimum Value
Calculating the Range from a Stem and Leaf Plot
Determining the range from a stem and leaf plot is straightforward. Simply identify the highest and lowest values directly from the plot and apply the range formula.
Example (continuing from the previous example):
Looking at our stem and leaf plot:
Stem | Leaf
-------
7 | 2 5 8
8 | 0 2 5 7 8 9
9 | 0 1 2 3 4 5
The minimum value is 72 (stem 7, leaf 2), and the maximum value is 95 (stem 9, leaf 5).
Therefore, the range is:
Range = 95 - 72 = 23
This indicates that the scores span a range of 23 points.
Interpreting the Range in the Context of a Stem and Leaf Plot
The range, when considered alongside the stem and leaf plot itself, provides a more comprehensive understanding of the data distribution. A large range might suggest the presence of outliers or a highly variable dataset, while a small range could indicate a relatively homogeneous dataset.
Example: Consider two datasets with the same mean but different ranges. One dataset might have a small range, indicating that the data points are tightly clustered around the mean. Conversely, another dataset with a large range would show a wider spread of data points, possibly with some outliers significantly deviating from the mean. Visualizing both datasets using stem and leaf plots would clearly highlight these differences in data distribution.
Limitations of the Range
While the range is easy to calculate and understand, it has some limitations:
- Sensitivity to Outliers: The range is highly sensitive to outliers. A single extreme value can significantly inflate the range, making it an unreliable measure of dispersion in datasets with outliers.
- Ignoring Data Distribution: The range only considers the extreme values and doesn't provide information about the distribution of data points between these extremes. A dataset with a large range might have most of its data points clustered near the mean, with a few outliers at the extremes.
- Not Suitable for All Data Types: The range is primarily suitable for numerical data. It cannot be directly applied to categorical or qualitative data.
Range and Other Measures of Dispersion
The range is a simple measure of dispersion, but it's often used in conjunction with other, more robust measures, such as:
- Interquartile Range (IQR): The IQR is the difference between the third quartile (Q3) and the first quartile (Q1) of a dataset. It's less sensitive to outliers than the range.
- Variance and Standard Deviation: These measures quantify the average squared deviation of data points from the mean, providing a more comprehensive picture of data dispersion.
Using Range for Data Analysis and Interpretation
The range, when used effectively within the context of a stem and leaf plot, can assist in several aspects of data analysis:
- Identifying Outliers: A stem and leaf plot visually highlights outliers, which can then be further investigated using the range to quantify their impact.
- Comparing Datasets: The range allows for easy comparison of data spread across multiple datasets. Comparing the ranges of different datasets displayed using stem and leaf plots can highlight which dataset exhibits greater variability.
- Preliminary Data Exploration: The range provides a quick and easy initial assessment of data variability, paving the way for more in-depth analysis.
Advanced Applications and Considerations
The interpretation of the range can be enhanced by considering:
- Data Context: The meaning of the range depends heavily on the context of the data. A range of 23 test scores might be significant, but a range of 23 years in a dataset of ages might be less significant.
- Data Scaling: The units of measurement affect the interpretation of the range. Transforming data to a different scale might be necessary before calculating the range and interpreting its significance.
- Combined with Other Statistics: The range should not be considered in isolation. Analyzing it alongside other descriptive statistics such as the mean, median, mode, and quartiles provides a more complete understanding of the data distribution.
Conclusion
The range, as a descriptive statistic, provides valuable insights into the variability of data when used in conjunction with a stem and leaf plot. While it has its limitations, primarily its sensitivity to outliers, its simplicity and ease of calculation make it a useful tool for initial data exploration and comparative analysis. By understanding its strengths and limitations, and by using it in conjunction with other statistical measures, researchers and analysts can leverage the range to effectively interpret data and draw meaningful conclusions. Remembering its context within the visual representation of a stem and leaf plot enhances its utility significantly. Therefore, mastering the concept of range and its relationship to stem and leaf plots is crucial for anyone working with statistical data.
Latest Posts
Latest Posts
-
How Many Inches In 13 Cm
Apr 04, 2025
-
How Many Cups Are In 125 Ml
Apr 04, 2025
-
What Percent Of 120 I S6
Apr 04, 2025
-
Explain Why The Composition Of An Element Is Fixed
Apr 04, 2025
-
58 Inches Is How Many Feet
Apr 04, 2025
Related Post
Thank you for visiting our website which covers about Range Of Stem And Leaf Plot . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
