What Is 18 Out Of 30
Kalali
Apr 23, 2025 · 5 min read
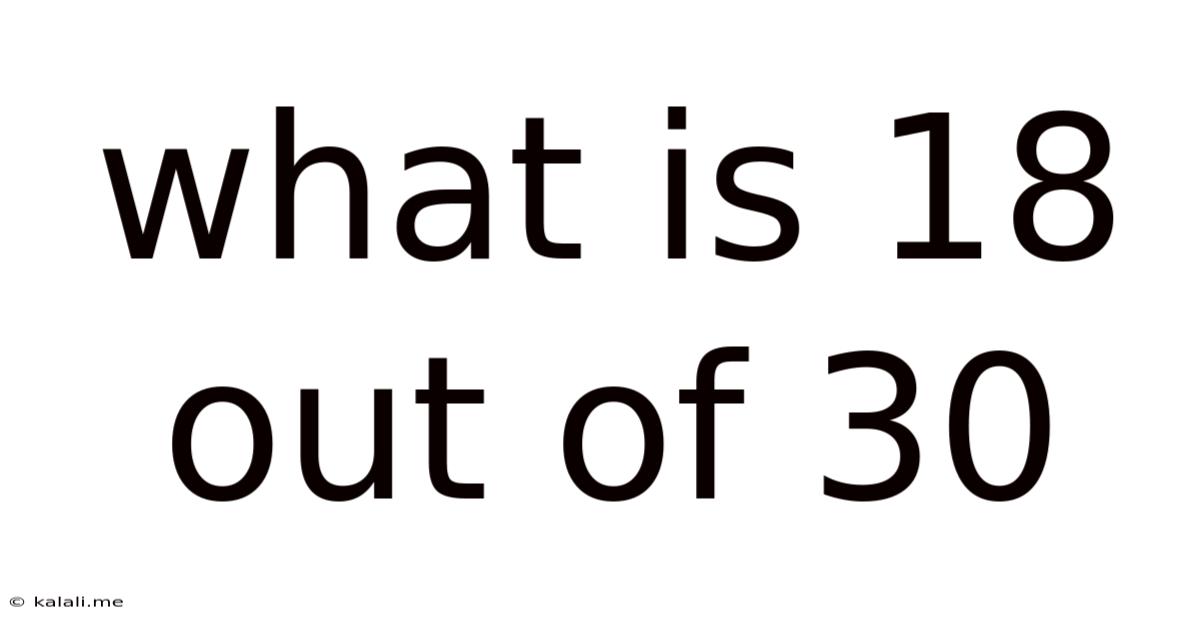
Table of Contents
What is 18 out of 30? Understanding Fractions, Percentages, and Ratios
What does "18 out of 30" mean? At first glance, it seems simple. But this seemingly straightforward question opens the door to a deeper understanding of fundamental mathematical concepts like fractions, percentages, and ratios. This comprehensive guide will explore "18 out of 30" in detail, demonstrating how to represent it in different ways and showcasing its practical applications. We'll also delve into related concepts and explore how this simple calculation can be used to solve more complex problems. Understanding these core concepts will improve your math skills and help you navigate various real-world scenarios.
Understanding the Basic Representation: Fractions
The phrase "18 out of 30" directly translates to a fraction: 18/30. This fraction represents 18 parts out of a total of 30 parts. The number 18 is the numerator (the top number), representing the part we're interested in, while 30 is the denominator (the bottom number), representing the whole. This is the most fundamental way to represent this mathematical relationship.
Simplifying the Fraction
The fraction 18/30 can be simplified. Simplification means reducing the fraction to its lowest terms by dividing both the numerator and the denominator by their greatest common divisor (GCD). The GCD of 18 and 30 is 6. Dividing both numbers by 6, we get:
18 ÷ 6 = 3 30 ÷ 6 = 5
Therefore, the simplified fraction is 3/5. This means that 18 out of 30 is equivalent to 3 out of 5. Simplifying fractions is important because it makes them easier to understand and compare.
Converting to a Percentage
Fractions can be easily converted into percentages. Percentages are a way of expressing a fraction as a proportion of 100. To convert 18/30 (or its simplified form, 3/5) to a percentage, we perform the following calculation:
(18/30) * 100% = 60%
Alternatively, using the simplified fraction:
(3/5) * 100% = 60%
Therefore, 18 out of 30 is equivalent to 60%. This percentage representation is particularly useful for comparing proportions and understanding relative quantities.
Representing the Data as a Ratio
Another way to represent "18 out of 30" is as a ratio. A ratio compares two quantities. In this case, the ratio is 18:30, which can be simplified to 3:5 by dividing both numbers by their GCD (6). This ratio signifies that for every 3 parts of one quantity, there are 5 parts of the total quantity. Ratios are commonly used in various fields, including recipes, scaling, and comparing different quantities.
Practical Applications and Real-World Examples
Understanding "18 out of 30" and its different representations has numerous real-world applications. Consider these examples:
1. Test Scores: If a student answered 18 questions correctly out of 30 on a test, their score would be 60%. This allows for easy comparison with other students' scores.
2. Sales Performance: A salesperson made 18 sales out of 30 potential clients. This translates to a 60% success rate, which is a valuable metric for assessing performance and identifying areas for improvement.
3. Survey Results: In a survey of 30 people, 18 responded positively to a particular question. This represents 60% positive responses, providing insightful data for market research or opinion polls.
4. Production Efficiency: A factory produced 18 acceptable items out of 30 produced. This 60% yield rate is crucial for assessing production efficiency and identifying potential bottlenecks.
5. Project Completion: A project consisting of 30 tasks is 18 tasks complete. This means 60% of the project is finished.
6. Statistical Analysis: In statistical analysis, "18 out of 30" forms the basis for calculating probabilities and making inferences about populations.
Expanding the Concept: Proportion and Proportionality
The concept of "18 out of 30" extends to the broader mathematical concepts of proportion and proportionality. A proportion is a statement that two ratios are equal. For instance, the proportion 18/30 = 3/5 demonstrates that these two ratios are equivalent. Proportionality refers to the relationship between two quantities where their ratio remains constant.
Understanding proportions and proportionality allows us to solve for unknown quantities in various scenarios. For example, if we know that 3 out of 5 apples are red, and we have a total of 15 apples, we can use proportions to find out how many red apples there are:
3/5 = x/15
Solving for x (the number of red apples):
x = (3 * 15) / 5 = 9
Therefore, there are 9 red apples.
Dealing with More Complex Scenarios: Improper Fractions and Mixed Numbers
While 18/30 is a proper fraction (numerator is less than the denominator), let's consider a scenario where we have more successes than the total possible. For example, imagine scoring 36 points out of 30. This results in an improper fraction (36/30), where the numerator is greater than the denominator. Improper fractions can be converted into mixed numbers, which consist of a whole number and a proper fraction.
36/30 simplifies to 6/5. As a mixed number this is 1 1/5. This means a score exceeding the total possible score; 1 and 1/5 times the total score.
Beyond the Basics: Applying Advanced Mathematical Techniques
The simple concept of "18 out of 30" can be further explored using more advanced mathematical techniques. For instance, we can utilize statistical methods to analyze the significance of this proportion, assess the margin of error, and draw inferences about the population from which the sample was drawn.
Furthermore, understanding "18 out of 30" is fundamental to grasping more advanced concepts like probability theory, where this ratio would represent the probability of a specific event occurring.
Conclusion:
The seemingly simple question, "What is 18 out of 30?" unveils a wealth of mathematical concepts – fractions, percentages, ratios, proportions, and their practical applications. By understanding these concepts and their interrelationships, we can effectively represent data, solve problems, and gain insights in various real-world situations. This understanding serves as a crucial foundation for further exploration into more advanced mathematical and statistical fields. The ability to interpret and manipulate these concepts confidently contributes significantly to enhanced problem-solving skills and a deeper understanding of quantitative information.
Latest Posts
Latest Posts
-
What Percent Of 60 Is 21
Apr 23, 2025
-
151 Cm Is How Many Inches
Apr 23, 2025
-
Cuantos Galones Hay En Un Litro
Apr 23, 2025
-
How Many Cups Is 30 Fl Oz
Apr 23, 2025
-
Why Is Pulmonary Circulation Reduced In The Unborn Fetus
Apr 23, 2025
Related Post
Thank you for visiting our website which covers about What Is 18 Out Of 30 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.