What Percent Of 60 Is 21
Kalali
Apr 23, 2025 · 5 min read
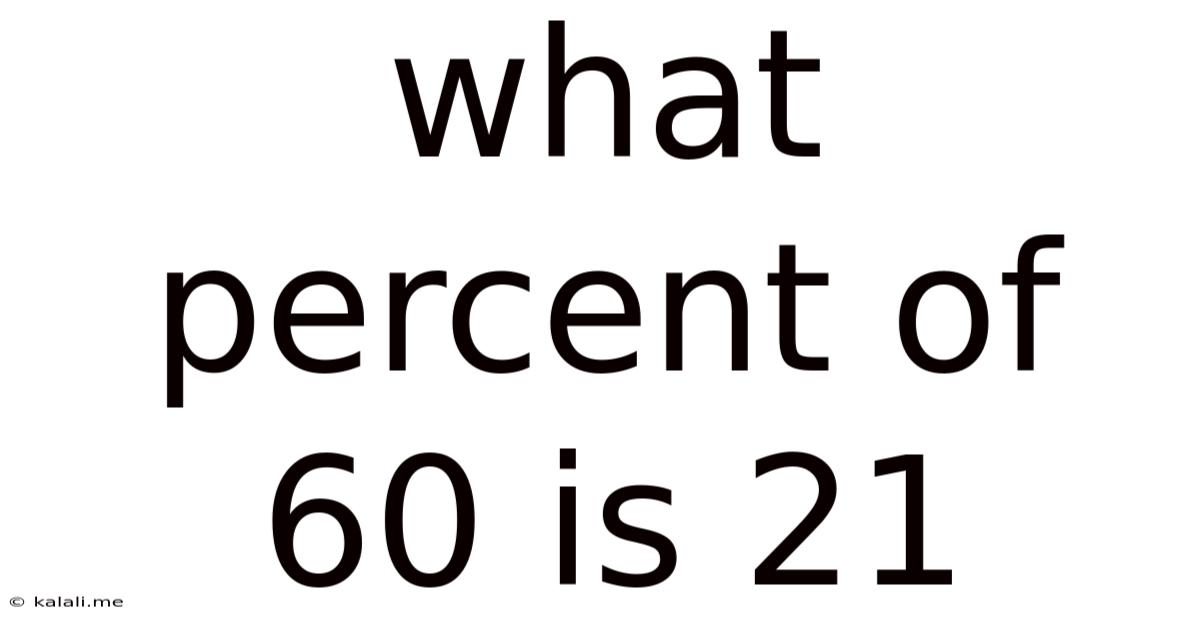
Table of Contents
What Percent of 60 is 21? A Deep Dive into Percentage Calculations and Their Applications
Finding what percent of 60 is 21 might seem like a simple arithmetic problem, but understanding the underlying principles unlocks a world of applications across various fields. This article delves deep into this seemingly basic calculation, exploring different methods for solving it, explaining the underlying concepts, and highlighting its relevance in everyday life, business, and beyond. We'll go beyond the simple answer and equip you with the knowledge to tackle similar percentage problems with confidence.
Understanding Percentages: The Foundation
Before we tackle the specific problem, let's solidify our understanding of percentages. A percentage is simply a fraction expressed as a number out of 100. The percentage sign (%) represents this division by 100. For instance, 50% is equivalent to 50/100, which simplifies to 1/2 or 0.5. Understanding this fundamental relationship is crucial for all percentage calculations.
Method 1: The Proportion Method
The most straightforward approach to solving "What percent of 60 is 21?" involves setting up a proportion. A proportion is an equation stating that two ratios are equal. We can express the problem as:
- x/100 = 21/60
Here, 'x' represents the unknown percentage we're trying to find. To solve for 'x', we cross-multiply:
- 60x = 2100
Then, divide both sides by 60:
- x = 2100/60 = 35
Therefore, 21 is 35% of 60.
Method 2: The Decimal Method
Another effective method involves converting the percentage to a decimal. We can express the problem as an equation:
- x * 60 = 21
To isolate 'x', we divide both sides by 60:
- x = 21/60
Now, we simplify the fraction:
- x = 0.35
Finally, we convert the decimal to a percentage by multiplying by 100:
- x = 0.35 * 100 = 35%
This method provides the same result, confirming that 21 is indeed 35% of 60.
Method 3: Using a Calculator
Modern calculators simplify percentage calculations significantly. Most calculators have a percentage function. You can simply input "21 ÷ 60 =" and then multiply the result by 100 to get the percentage. This provides a quick and efficient way to solve the problem, especially for more complex scenarios.
Real-World Applications of Percentage Calculations
Understanding percentage calculations is essential in numerous real-world situations:
-
Business and Finance: Calculating profit margins, discounts, interest rates, tax rates, and investment returns all rely heavily on percentage calculations. For example, understanding how much a 10% increase in sales impacts profit requires precise percentage calculations. Analyzing financial statements and making informed business decisions relies on this skill.
-
Sales and Marketing: Offering discounts, understanding conversion rates, and analyzing customer demographics often involve percentage analysis. Calculating the percentage of customers who complete a purchase after clicking an advertisement is crucial for evaluating marketing campaign effectiveness. Setting sales targets and tracking progress also requires an understanding of percentages.
-
Science and Research: In scientific research, percentages are used to express data proportions, statistical significance, and experimental error. Reporting experimental results and drawing conclusions often requires the precise representation of data as percentages. For example, reporting the percentage of subjects showing a particular response in a clinical trial is crucial for interpreting research findings.
-
Everyday Life: Calculating tips in restaurants, understanding sale prices in stores, and determining the amount of a discount are commonplace scenarios where knowledge of percentages is valuable. Even calculating the percentage of your monthly budget allocated to different expenses requires this understanding.
-
Education and Testing: Calculating grades, understanding scores on standardized tests, and evaluating performance on assessments often involve percentages. Converting raw scores to percentages provides a standardized measure of achievement.
-
Health and Fitness: Monitoring body fat percentage, calculating nutrient intake as a percentage of daily recommendations, and tracking progress towards fitness goals require an understanding of percentages. Many health and fitness tracking apps and devices rely heavily on percentages to present data to the user.
Expanding on Percentage Problems: More Complex Scenarios
While the problem "What percent of 60 is 21?" is relatively straightforward, the principles can be extended to more complex scenarios. Consider these examples:
-
Finding a Percentage of a Number: If you need to find 15% of 250, you would multiply 250 by 0.15 (15/100). This results in 37.5.
-
Finding the Original Number: If 20% of a number is 30, you can set up an equation: 0.20x = 30. Solving for x (by dividing both sides by 0.20), you find the original number is 150.
-
Comparing Percentages: You might need to compare the growth rate of two different businesses over a certain period. This involves calculating the percentage change for each business and then comparing the results.
-
Percentage Increase/Decrease: Calculating a percentage increase or decrease is crucial in many applications. For instance, calculating the percentage increase in sales from one year to the next or the percentage decrease in unemployment.
Mastering Percentages: Tips and Techniques
Mastering percentage calculations involves consistent practice and a solid understanding of the underlying concepts. Here are some tips:
-
Practice Regularly: The more you practice, the more comfortable you'll become with these calculations. Try solving various percentage problems to build your skills.
-
Use Different Methods: Familiarize yourself with different methods for solving percentage problems. This will help you choose the most efficient approach based on the specific problem.
-
Break Down Complex Problems: If you encounter a complex percentage problem, break it down into smaller, more manageable steps.
-
Utilize Online Resources: Many online resources offer practice problems and explanations of percentage calculations.
Conclusion:
The seemingly simple question, "What percent of 60 is 21?" serves as a gateway to understanding the broader world of percentage calculations. From the basics of proportions and decimal conversions to the real-world applications across diverse fields, this article has provided a comprehensive overview. By mastering these techniques and understanding their relevance, you'll be well-equipped to tackle various percentage problems with confidence and effectively apply this fundamental mathematical concept in your personal and professional life. Remember, consistent practice and a firm grasp of the underlying principles are key to achieving proficiency in percentage calculations.
Latest Posts
Latest Posts
-
Definition For Same Side Interior Angles
Apr 23, 2025
-
How Many Milliliters Is 12 Ounces
Apr 23, 2025
-
2 1 4 As An Improper Fraction
Apr 23, 2025
-
What Percent Of 60 Is 150
Apr 23, 2025
-
How Many Feet Are In 86 Inches
Apr 23, 2025
Related Post
Thank you for visiting our website which covers about What Percent Of 60 Is 21 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.