What Is 2.6 As A Fraction
Kalali
Apr 12, 2025 · 5 min read
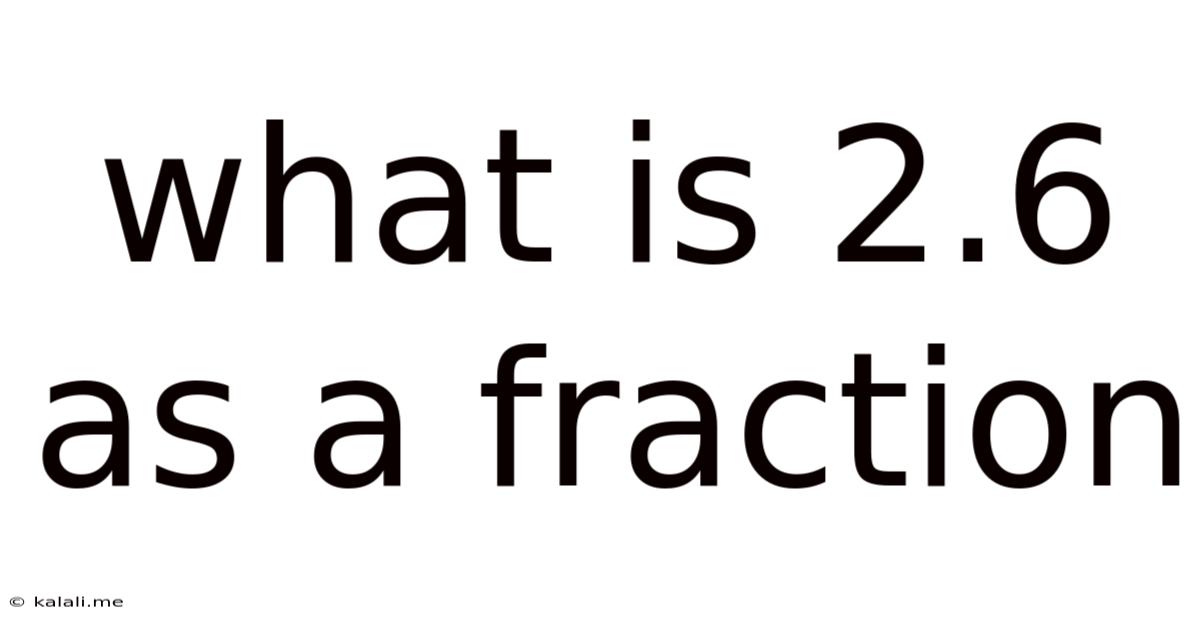
Table of Contents
What is 2.6 as a Fraction? A Comprehensive Guide
The seemingly simple question, "What is 2.6 as a fraction?", opens the door to a deeper understanding of decimal-to-fraction conversion and the underlying principles of representing numbers. This comprehensive guide will not only answer this question definitively but also explore the broader mathematical concepts involved, offering various methods and practical applications. This will ensure you not only know the answer but also understand the "why" behind the process, equipping you with the knowledge to tackle similar conversions confidently.
Meta Description: Learn how to convert the decimal 2.6 into a fraction. This guide provides a step-by-step explanation, explores different methods, and delves into the broader mathematical concepts involved, offering a comprehensive understanding of decimal-to-fraction conversion.
Understanding Decimals and Fractions
Before diving into the conversion, let's briefly review the fundamental concepts of decimals and fractions. Decimals represent numbers as a sum of powers of ten, using a decimal point to separate the whole number part from the fractional part. For instance, 2.6 represents 2 whole units and 6 tenths.
Fractions, on the other hand, express a number as a ratio of two integers – a numerator (the top number) and a denominator (the bottom number). The denominator indicates the number of equal parts a whole is divided into, while the numerator shows how many of those parts are being considered.
The core of converting a decimal to a fraction lies in understanding that decimals and fractions represent the same value, just expressed differently. The conversion process aims to find the equivalent fractional representation of a given decimal.
Method 1: The Direct Conversion Method
This is the most straightforward approach for converting a decimal like 2.6 to a fraction.
-
Identify the whole number and the decimal part: In 2.6, the whole number is 2, and the decimal part is 0.6.
-
Convert the decimal part to a fraction: The decimal 0.6 represents six-tenths, which can be written as the fraction 6/10.
-
Combine the whole number and the fraction: We now have 2 and 6/10. To express this as a single improper fraction, we multiply the whole number by the denominator and add the numerator: (2 * 10) + 6 = 26. This becomes the new numerator, while the denominator remains the same.
-
The final fraction: Therefore, 2.6 as a fraction is 26/10.
-
Simplification: This fraction can be simplified by finding the greatest common divisor (GCD) of the numerator and denominator. The GCD of 26 and 10 is 2. Dividing both the numerator and denominator by 2, we get the simplified fraction 13/5.
Method 2: Using Place Value
This method emphasizes the place value of each digit in the decimal number.
-
Identify the place value of the last digit: In 2.6, the last digit (6) is in the tenths place.
-
Write the decimal as a fraction based on place value: Since 6 is in the tenths place, the fraction representation is 6/10.
-
Add the whole number: Combine the whole number (2) with the fraction 6/10, resulting in 2 and 6/10.
-
Convert to an improper fraction: Following the same steps as Method 1, this becomes 26/10.
-
Simplify: Simplify the fraction to its lowest terms, which is 13/5.
Method 3: Using Powers of 10
This method leverages the concept of expressing decimals as fractions with powers of 10 as denominators.
-
Write the decimal without the decimal point: Write 2.6 as 26.
-
Determine the denominator: Count the number of digits after the decimal point in the original decimal (one digit). This indicates the power of 10 to use as the denominator: 10¹.
-
Form the fraction: The fraction becomes 26/10.
-
Simplify: Simplify the fraction to its lowest terms, resulting in 13/5.
Mixed Numbers vs. Improper Fractions
In the previous methods, we arrived at the improper fraction 13/5. This means the numerator (13) is greater than the denominator (5). It's also possible to express this as a mixed number, which combines a whole number and a proper fraction.
To convert 13/5 to a mixed number, divide the numerator (13) by the denominator (5):
13 ÷ 5 = 2 with a remainder of 3.
The quotient (2) becomes the whole number, and the remainder (3) becomes the numerator of the fraction, with the denominator remaining the same (5). Therefore, 13/5 as a mixed number is 2 3/5. Both 13/5 and 2 3/5 represent the same value as the decimal 2.6.
Practical Applications and Further Exploration
Understanding decimal-to-fraction conversion has numerous practical applications in various fields:
-
Cooking and Baking: Many recipes use fractions for ingredient measurements. Converting decimal measurements from a digital scale to fractions ensures accuracy.
-
Construction and Engineering: Precision is paramount in construction and engineering. Converting decimal measurements to fractions helps in accurate calculations and blueprint interpretations.
-
Finance: Working with percentages and interest rates often involves converting decimals to fractions for precise calculations.
-
Data Analysis and Statistics: Representing data in fractional form can be crucial for understanding proportions and ratios in statistical analysis.
-
Advanced Mathematics: Decimal-to-fraction conversion forms a foundational skill in more advanced mathematical concepts like algebra and calculus.
Converting More Complex Decimals
The methods outlined above can be adapted to convert more complex decimals to fractions. For example, to convert 3.125 to a fraction:
-
Separate the whole number and decimal part: Whole number = 3, decimal part = 0.125.
-
Convert the decimal part: 0.125 represents 125 thousandths, which is 125/1000.
-
Combine and simplify: 3 + 125/1000 = (3000 + 125)/1000 = 3125/1000. Simplifying this fraction by dividing both numerator and denominator by their GCD (125) results in 25/8.
Conclusion
Converting a decimal like 2.6 to a fraction is a fundamental mathematical skill with widespread applications. While the answer is definitively 13/5 (or its equivalent mixed number, 2 3/5), understanding the underlying principles and different conversion methods is crucial. This deeper understanding empowers you to tackle more complex conversions confidently and apply this knowledge across various practical scenarios. By mastering this seemingly simple task, you build a strong foundation for more advanced mathematical explorations. Remember to always simplify your fractions to their lowest terms for the most concise representation.
Latest Posts
Latest Posts
-
Cuantos Grados Centigrados Son 105 Fahrenheit
Apr 13, 2025
-
Ten To The Power Of 100
Apr 13, 2025
-
What Is The Percent For 1 20
Apr 13, 2025
-
154 Centimeters In Feet And Inches
Apr 13, 2025
-
How Much Is A 1 2 Pint
Apr 13, 2025
Related Post
Thank you for visiting our website which covers about What Is 2.6 As A Fraction . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.