What Is 20 Off Of 45
Kalali
Apr 12, 2025 · 5 min read
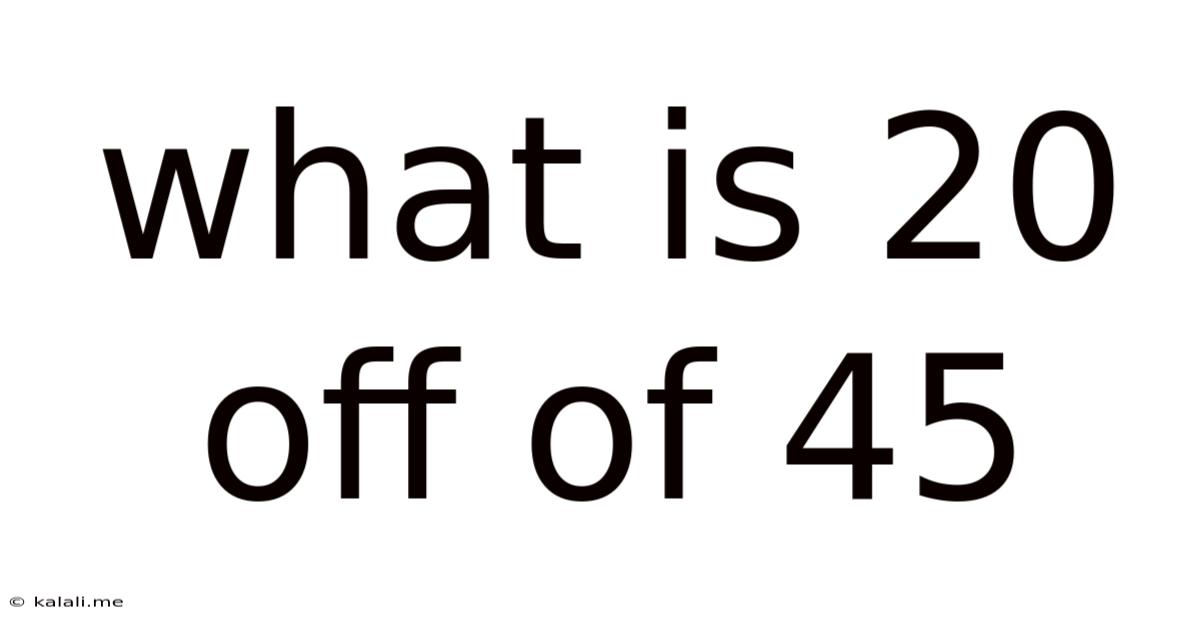
Table of Contents
What is 20% Off of 45? A Deep Dive into Percentage Calculations and Their Applications
This seemingly simple question, "What is 20% off of 45?", opens the door to a broader understanding of percentage calculations, their real-world applications, and even some surprisingly relevant mathematical concepts. This article will not only answer the initial question but also explore the underlying principles, provide multiple methods for solving similar problems, and demonstrate the practical uses of percentage calculations in various scenarios.
Meta Description: Learn how to calculate 20% off of 45 and master percentage calculations. This comprehensive guide explores different methods, real-world applications, and advanced concepts related to percentage discounts.
The straightforward answer is $36. But let's delve deeper into how we arrive at this answer and explore the various ways to approach this type of problem.
Method 1: Calculating the Discount Amount Directly
The most intuitive method involves calculating the discount amount first and then subtracting it from the original price.
-
Convert the percentage to a decimal: 20% is equivalent to 0.20 (divide 20 by 100).
-
Multiply the original price by the decimal: 45 * 0.20 = 9. This represents the discount amount.
-
Subtract the discount from the original price: 45 - 9 = 36. Therefore, 20% off of 45 is $36.
Method 2: Calculating the Remaining Percentage
This method focuses on finding the percentage of the original price that remains after the discount.
-
Find the remaining percentage: If 20% is discounted, then 100% - 20% = 80% of the original price remains.
-
Convert the remaining percentage to a decimal: 80% is equivalent to 0.80.
-
Multiply the original price by the remaining decimal: 45 * 0.80 = 36. This directly gives you the final price after the discount.
Method 3: Using Proportions
This method leverages the concept of ratios and proportions, a fundamental aspect of algebra.
We can set up a proportion:
- Let 'x' be the discounted price.
- We know that 20% of 45 is the discount, so (20/100) = (x/45)
Solving for 'x':
- Cross-multiply: 20 * 45 = 100 * x
- Simplify: 900 = 100x
- Solve for x: x = 900 / 100 = 9 (this is the discount amount)
- Subtract the discount from the original price: 45 - 9 = 36
Beyond the Basics: Expanding on Percentage Calculations
The simple calculation above is a springboard to understanding more complex percentage problems. Let's explore some related concepts:
Calculating Percentage Increase/Decrease
Percentage calculations aren't limited to discounts. They're crucial for understanding changes in various quantities, such as population growth, economic fluctuations, or even the appreciation/depreciation of assets.
For example, if a quantity increases from 45 to 54, the percentage increase can be calculated as follows:
- Find the difference: 54 - 45 = 9
- Divide the difference by the original value: 9 / 45 = 0.2
- Multiply by 100 to express as a percentage: 0.2 * 100 = 20%
Therefore, there's a 20% increase from 45 to 54. Similarly, you can calculate percentage decreases using the same principle.
Compounding Percentages
When dealing with multiple consecutive percentage changes, the concept of compounding comes into play. This is particularly relevant in finance, where interest rates are often compounded annually or even more frequently.
Imagine a 20% discount followed by a further 10% discount. It's crucial to understand that these discounts don't simply add up to 30%. Instead, each discount is calculated on the remaining value.
- First discount: 45 * 0.80 = 36
- Second discount: 36 * 0.90 = 32.40
The final price after both discounts is $32.40, not $31.50 (45 * 0.70).
Applications in Real-World Scenarios
Percentage calculations are pervasive in everyday life. Here are some examples:
- Sales and discounts: Retail stores frequently offer percentage discounts to attract customers.
- Taxes and tips: Calculating sales tax or adding a service tip involves percentage calculations.
- Finance and investments: Interest rates, returns on investments, and loan payments all involve percentages.
- Statistics and data analysis: Percentages are widely used to represent proportions and trends in data.
- Science and Engineering: Percentage error, efficiency calculations, and concentration levels often utilize percentage calculations.
Advanced Concepts and Considerations
While the basic calculations are relatively straightforward, understanding the nuances of percentage calculations can be more challenging. Here are some advanced concepts to consider:
-
Weighted Averages: When calculating the average of multiple percentages, you might need to consider their weights. For instance, if one item contributes 60% and another contributes 40%, their weighted average will reflect their respective contributions.
-
Percentage Points vs. Percentages: It's crucial to distinguish between a percentage point change and a percentage change. For example, an increase from 20% to 25% is a 5 percentage point increase but a 25% increase relative to the original value.
-
Dealing with Complex Scenarios: Real-world scenarios often involve multiple discounts, taxes, or other factors that require a layered approach to percentage calculations. This might involve using multiple steps or formulating algebraic equations to accurately determine the final result.
Conclusion: Mastering Percentage Calculations
This article has moved beyond the simple question of "What is 20% off of 45?" to explore the broader world of percentage calculations. We've demonstrated several methods for solving basic percentage problems and expanded into more advanced concepts and their practical applications. Mastering these calculations is essential for anyone navigating the complexities of everyday life, finance, and numerous other fields. Remember, understanding the underlying principles, not just the formulas, allows for flexible problem-solving and a deeper comprehension of this fundamental mathematical tool. The ability to quickly and accurately calculate percentages provides a significant advantage in various aspects of life, from personal finance to professional settings.
Latest Posts
Latest Posts
-
Cuanto Tiene Un Metro En Pies
Apr 13, 2025
-
Common Multiple Of 5 And 9
Apr 13, 2025
-
40 Is What Percent Of 24
Apr 13, 2025
-
How Many Oz Is 500ml Of Water
Apr 13, 2025
-
1 9 M In Inches And Feet
Apr 13, 2025
Related Post
Thank you for visiting our website which covers about What Is 20 Off Of 45 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.