What Is 25 Off Of 30
Kalali
Apr 17, 2025 · 4 min read
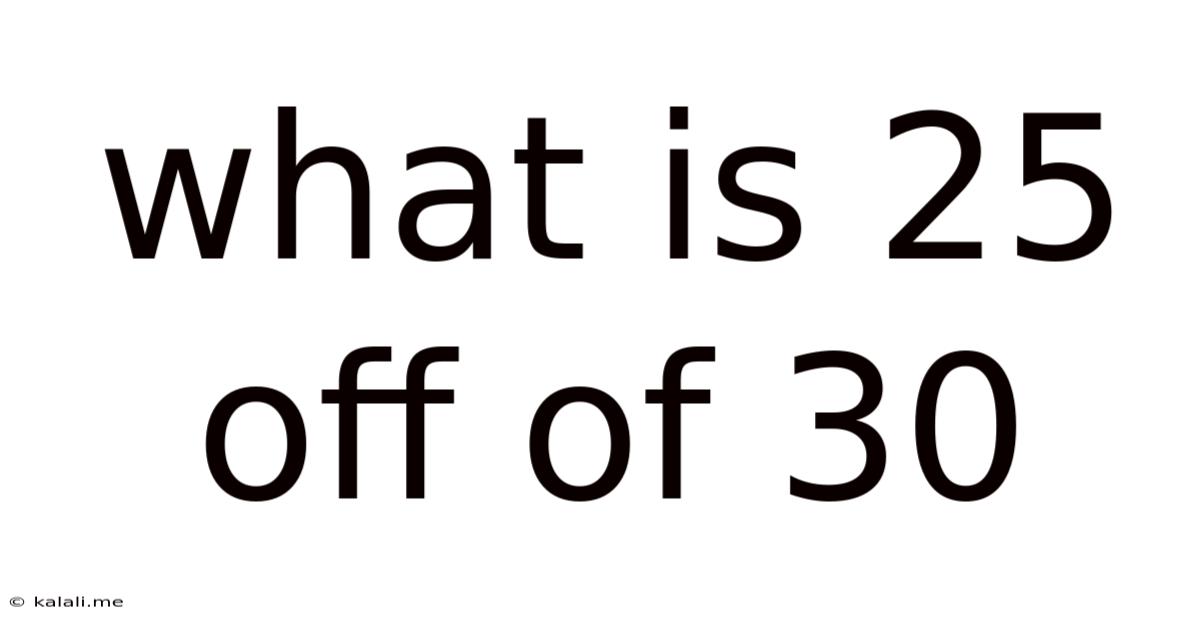
Table of Contents
What is 25% Off of 30? A Comprehensive Guide to Percentage Calculations
This article delves into the seemingly simple question: "What is 25% off of 30?" While the answer might seem immediately obvious to some, understanding the underlying principles of percentage calculations is crucial for various applications in everyday life, from shopping and budgeting to more complex financial calculations and data analysis. This guide will not only provide the answer but also equip you with the knowledge and methods to solve similar percentage problems efficiently and accurately. We'll explore different approaches, discuss common pitfalls, and offer practical examples to solidify your understanding.
Understanding Percentages
A percentage is a fraction or ratio expressed as a number out of 100. The symbol "%" represents "per cent," meaning "out of one hundred." Understanding this fundamental concept is key to mastering percentage calculations. For instance, 25% means 25 out of 100, which can be written as the fraction 25/100 or the decimal 0.25.
Method 1: Using Decimal Conversion
The most straightforward approach to calculating 25% off of 30 is to convert the percentage to a decimal and then multiply it by the original amount.
-
Convert the percentage to a decimal: 25% is equivalent to 0.25 (divide 25 by 100).
-
Multiply the decimal by the original amount: 0.25 * 30 = 7.5
-
This result (7.5) represents the discount amount.
-
Subtract the discount from the original amount to find the final price: 30 - 7.5 = 22.5
Therefore, 25% off of 30 is $22.5.
Method 2: Using Fractions
Percentages can also be expressed as fractions. This method can be particularly helpful when dealing with simpler percentages.
-
Convert the percentage to a fraction: 25% can be written as 25/100, which simplifies to 1/4.
-
Multiply the fraction by the original amount: (1/4) * 30 = 7.5
-
This result (7.5) is the discount amount.
-
Subtract the discount from the original amount: 30 - 7.5 = 22.5
Again, the final price after a 25% discount on 30 is $22.5.
Method 3: Finding the Remaining Percentage
Instead of calculating the discount and subtracting it, we can directly calculate the remaining percentage and multiply it by the original amount.
-
Determine the remaining percentage: If we're taking 25% off, then 100% - 25% = 75% remains.
-
Convert the remaining percentage to a decimal: 75% is equivalent to 0.75.
-
Multiply the decimal by the original amount: 0.75 * 30 = 22.5
This method directly gives us the final price after the discount: $22.5.
Practical Applications and Real-World Examples
Understanding percentage calculations is essential in various everyday situations:
-
Shopping: Calculating discounts on clothing, electronics, or groceries. Imagine a $50 shirt with a 15% discount; you can quickly determine the final price using the methods described above.
-
Sales Tax: Determining the final price of an item after adding sales tax. If the sales tax is 6%, you'll add 6% of the item's price to the original price.
-
Tips: Calculating appropriate tips in restaurants. A 20% tip on a $75 meal is easily calculated using the percentage methods.
-
Finance: Calculating interest earned on savings accounts or interest paid on loans. Compound interest calculations, while more complex, also rely on the fundamental principles of percentages.
-
Data Analysis: Percentages are vital for interpreting data and making comparisons in various fields like market research, healthcare, and economics.
Common Mistakes to Avoid
-
Incorrect Decimal Conversion: Ensure you accurately convert percentages to decimals before performing calculations. A simple mistake here can lead to significant errors in the final result.
-
Confusing Percentage Increase and Decrease: Remember to subtract the discount from the original amount when dealing with percentage discounts and add the increase when dealing with percentage increases.
-
Rounding Errors: Be mindful of rounding errors, especially when dealing with multiple calculations or complex scenarios. It's best to keep the numbers in decimal form until the final answer to maintain accuracy.
-
Misunderstanding the Base: Always identify the base number (the number you're taking the percentage of). In this case, 30 is the base number.
Advanced Percentage Calculations
While this article focuses on a simple percentage calculation, the underlying principles can be extended to more complex scenarios:
-
Successive Discounts: Calculating the final price after applying multiple discounts consecutively. For example, a 20% discount followed by a 10% discount is not simply a 30% discount; the second discount is applied to the already reduced price.
-
Percentage Changes: Determining the percentage increase or decrease between two numbers. This involves finding the difference between the two numbers, dividing it by the original number, and then multiplying by 100 to express the change as a percentage.
-
Compound Interest: Calculating the total amount after adding interest to the principal amount, where the interest earned is also added to the principal for subsequent interest calculations.
Conclusion:
Calculating 25% off of 30 is a simple yet fundamental percentage calculation. Mastering this and similar calculations equips you with essential skills for navigating various aspects of daily life and more complex financial and analytical tasks. By understanding the different methods – decimal conversion, fraction method, and remaining percentage approach – you can choose the most efficient and accurate strategy depending on the specific problem. Remember to avoid common mistakes and pay attention to detail to ensure accuracy in your calculations. The methods discussed in this guide provide a solid foundation for tackling a wide range of percentage-related problems.
Latest Posts
Latest Posts
-
What Percentage Is 18 Of 24
Apr 19, 2025
-
How Hot Is 90 Degrees Celsius
Apr 19, 2025
-
Cuanto Es 18 Libras En Kilos
Apr 19, 2025
-
24 Oz Is How Many Milliliters
Apr 19, 2025
-
How Many Protons Are In F
Apr 19, 2025
Related Post
Thank you for visiting our website which covers about What Is 25 Off Of 30 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.