What Is 25 Percent Of 1000
Kalali
Apr 27, 2025 · 6 min read
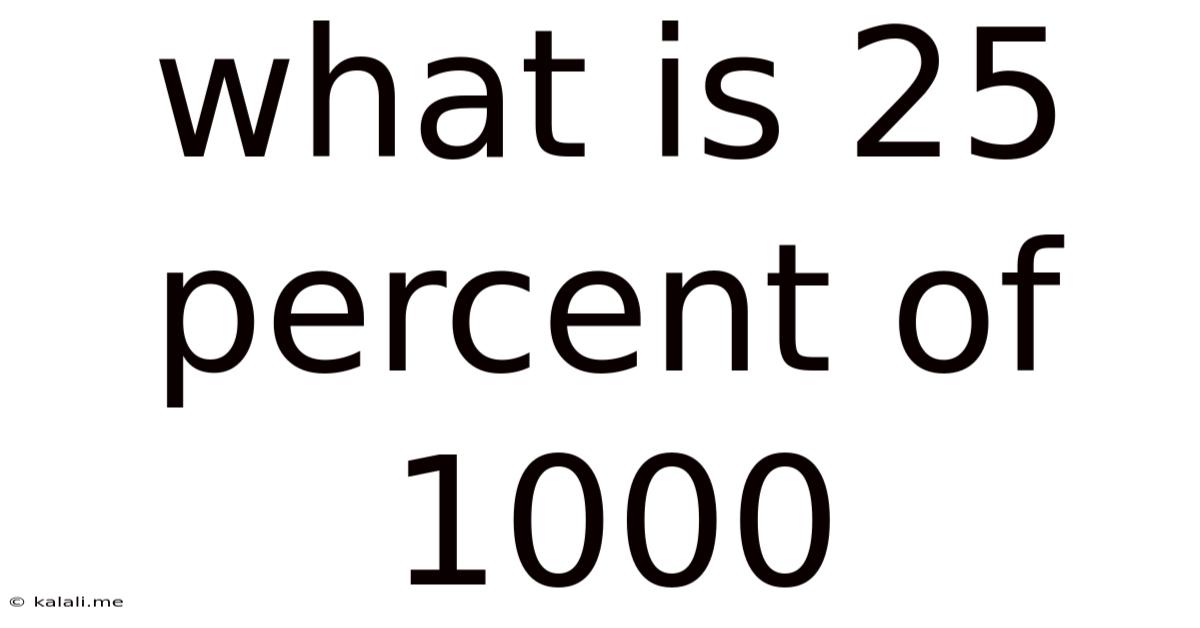
Table of Contents
What is 25 Percent of 1000? A Deep Dive into Percentages and Their Applications
Finding 25 percent of 1000 might seem like a simple calculation, but understanding the underlying principles of percentages opens the door to a vast world of applications in various fields. This article will not only answer the question directly but also explore the broader context of percentages, their calculations, and practical examples in everyday life and professional settings.
Meta Description: Discover how to calculate 25% of 1000 and delve into the world of percentages, exploring their applications in various fields, from finance to statistics. This comprehensive guide provides practical examples and tips for mastering percentage calculations.
What is 25 Percent of 1000? The Basic Calculation
The most straightforward way to find 25% of 1000 is to convert the percentage to a decimal and then multiply:
25% is equivalent to 0.25 (divide 25 by 100).
Therefore, 25% of 1000 = 0.25 * 1000 = 250
This simple calculation forms the foundation for understanding more complex percentage problems.
Understanding Percentages: A Fundamental Concept
Percentages are a way of expressing a number as a fraction of 100. The word "percent" literally means "out of one hundred" (per centum in Latin). They provide a standardized way to compare proportions and make calculations easier to understand, especially when dealing with ratios and proportions. Percentages are ubiquitous in various aspects of life, from calculating discounts and taxes to understanding statistical data and financial reports.
Beyond the Basics: Different Methods for Calculating Percentages
While the direct multiplication method is efficient, other methods can be equally helpful, especially when dealing with more complex scenarios or when mental calculations are preferred.
-
Fraction Method: 25% can be expressed as the fraction ¼ (25/100 simplifies to 1/4). Therefore, finding 25% of 1000 is the same as finding ¼ of 1000, which is 1000 ÷ 4 = 250. This method is particularly useful when dealing with common percentage values like 25%, 50%, and 75%.
-
Using Proportions: You can set up a proportion to solve for the unknown value. Let 'x' represent 25% of 1000. The proportion would be:
25/100 = x/1000
Cross-multiplying and solving for 'x' gives:
100x = 25000 x = 25000/100 = 250
This method is valuable for understanding the underlying relationship between percentages and proportions, making it a good approach for more complex problems.
Practical Applications of Percentage Calculations
The ability to calculate percentages is crucial in numerous fields. Here are some examples:
1. Finance and Business:
- Calculating discounts: Stores often offer discounts expressed as percentages. Understanding percentage calculations allows consumers to quickly determine the actual price after a discount. For example, a 15% discount on a $500 item would be 0.15 * $500 = $75, resulting in a final price of $425.
- Interest calculations: Interest rates on loans, savings accounts, and investments are expressed as percentages. Knowing how to calculate interest is essential for financial planning and managing debt. For instance, a 5% annual interest on a $10,000 loan would be $500 per year.
- Profit margins: Businesses use percentages to calculate profit margins, which represent the percentage of revenue that remains as profit after deducting costs.
- Tax calculations: Sales tax, income tax, and other taxes are often calculated as a percentage of the taxable amount.
2. Statistics and Data Analysis:
- Representing data: Percentages are used extensively in data representation to show proportions and trends in data sets. Charts and graphs often use percentages to visually communicate information.
- Probability and risk assessment: Probabilities and risks are often expressed as percentages, such as the probability of a certain event occurring or the risk of an investment failing.
3. Everyday Life:
- Tip calculations: Calculating tips at restaurants involves determining a percentage of the bill. A 15% tip on a $50 meal would be 0.15 * $50 = $7.50.
- Sales tax calculations: Sales tax is added to the price of goods and services, and understanding percentage calculations is crucial for determining the final price.
- Grade calculations: In many educational systems, grades are often expressed as percentages, representing the student's performance in relation to the total possible points.
Advanced Percentage Calculations and Problem Solving
While calculating 25% of 1000 is relatively straightforward, more complex scenarios may require a multi-step approach. Consider these examples:
-
Finding the original value: If you know a percentage and the resulting value, you can work backward to find the original value. For example, if 30% of a number is 60, you can set up the equation: 0.3x = 60. Solving for x gives x = 60/0.3 = 200. The original number was 200.
-
Percentage increase or decrease: Calculating percentage changes is essential for understanding growth or decline. The formula for percentage change is: [(New Value - Old Value) / Old Value] * 100. For example, if a stock price increases from $100 to $120, the percentage increase is [(120 - 100) / 100] * 100 = 20%.
-
Compound interest: Compound interest involves calculating interest on both the principal amount and accumulated interest. The formula for compound interest is: A = P (1 + r/n)^(nt), where A is the final amount, P is the principal amount, r is the annual interest rate, n is the number of times interest is compounded per year, and t is the number of years. Compound interest calculations are crucial for understanding long-term investment growth.
-
Percentage points: It's important to differentiate between percentage change and percentage points. A change from 10% to 15% is a 5 percentage point increase, but a 50% increase in the percentage.
Tips for Mastering Percentage Calculations
- Practice regularly: The best way to become proficient in percentage calculations is to practice regularly. Solve various problems involving different scenarios and complexities.
- Understand the concepts: Focusing solely on memorizing formulas without understanding the underlying concepts will hinder your ability to solve more complex problems.
- Use a calculator: For more complex calculations, especially those involving compound interest, use a calculator to save time and ensure accuracy.
- Break down complex problems: Divide complex problems into smaller, manageable steps to make the calculations less daunting.
- Check your work: Always check your work to ensure accuracy. Using different methods to solve the same problem can help confirm your answer.
Conclusion: The Power of Percentages
Understanding percentages is a fundamental skill applicable across various disciplines. From calculating simple discounts to analyzing complex financial models, the ability to work confidently with percentages is a valuable asset in both personal and professional life. While the answer to "What is 25 percent of 1000?" is a simple 250, the journey of understanding the broader context of percentages offers significant benefits and opens doors to more advanced mathematical concepts and applications. By mastering the fundamentals and exploring various methods of calculation, you can confidently navigate the world of percentages and apply your knowledge effectively.
Latest Posts
Latest Posts
-
27 4 As A Mixed Number
Apr 27, 2025
-
How Tall Is 1 63m In Feet
Apr 27, 2025
-
What Is 157 Cm In Inches
Apr 27, 2025
-
Inputs And Outputs Of The Citric Acid Cycle
Apr 27, 2025
-
Cuanto Son 24 Oz En Litros
Apr 27, 2025
Related Post
Thank you for visiting our website which covers about What Is 25 Percent Of 1000 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.