What Is 32 Out Of 50 As A Percentage
Kalali
Apr 06, 2025 · 5 min read
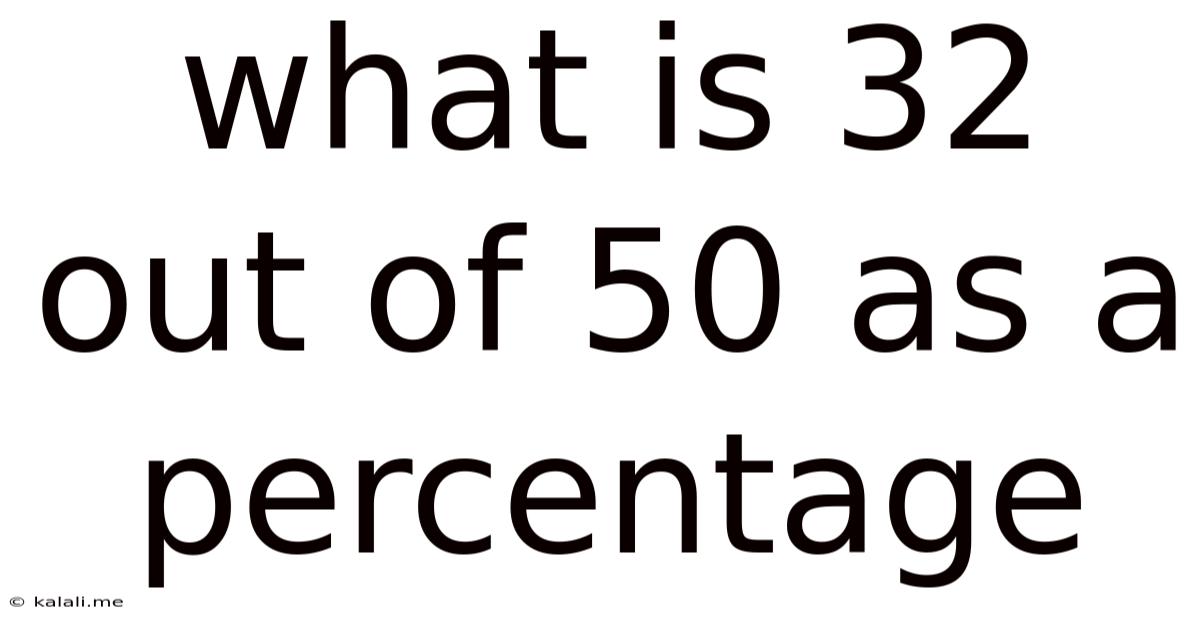
Table of Contents
What is 32 out of 50 as a Percentage? A Comprehensive Guide
Calculating percentages is a fundamental skill with wide-ranging applications in various aspects of life, from academic pursuits to professional settings. Understanding how to express fractions as percentages is crucial for interpreting data, making informed decisions, and communicating effectively. This article will delve into the process of determining what 32 out of 50 represents as a percentage, and further explore the broader concept of percentage calculations.
Understanding Percentages
A percentage is a way of expressing a number as a fraction of 100. The term "percent" literally means "out of one hundred" ( per centum in Latin). Percentages are used to represent proportions or ratios, making it easier to compare and understand different quantities relative to a whole. They are ubiquitous in everyday life, appearing in contexts like:
- Sales and discounts: "20% off" signifies a reduction of 20 units for every 100 units.
- Financial statements: Company performance is often expressed using percentages (profit margins, growth rates).
- Statistics and data analysis: Percentages are crucial for representing data sets and highlighting trends.
- Academic grades: Test scores and overall grades are commonly expressed as percentages.
Calculating 32 out of 50 as a Percentage
To find out what 32 out of 50 represents as a percentage, we follow these steps:
Step 1: Express the ratio as a fraction.
32 out of 50 can be written as the fraction 32/50.
Step 2: Convert the fraction to a decimal.
Divide the numerator (32) by the denominator (50): 32 ÷ 50 = 0.64
Step 3: Convert the decimal to a percentage.
Multiply the decimal by 100 and add the "%" symbol: 0.64 x 100 = 64%
Therefore, 32 out of 50 is 64%.
Alternative Methods for Percentage Calculation
While the above method is straightforward, several alternative approaches can be used to calculate percentages, particularly when dealing with more complex scenarios:
Using Proportions
This method involves setting up a proportion to solve for the unknown percentage. We can set up the proportion:
32/50 = x/100
Where 'x' represents the percentage we are trying to find. Solving for 'x' involves cross-multiplication:
50x = 3200
x = 3200/50
x = 64
Therefore, x = 64%.
Using the Percentage Formula
The basic percentage formula is:
Percentage = (Part / Whole) x 100
In our case:
Percentage = (32 / 50) x 100 = 64%
Practical Applications of Percentage Calculations
The ability to calculate percentages has far-reaching applications across many disciplines:
1. Finance and Budgeting:
- Calculating interest: Determining the interest earned on savings accounts or the interest paid on loans requires percentage calculations.
- Analyzing investment returns: Understanding the rate of return on investments relies heavily on percentage calculations.
- Managing personal finances: Budgeting involves tracking expenses as a percentage of income to maintain financial stability.
2. Sales and Marketing:
- Calculating discounts: Determining the final price after applying a discount percentage is a common application.
- Analyzing sales data: Tracking sales growth, customer acquisition costs, and conversion rates often involves percentage changes.
- Setting pricing strategies: Businesses use percentages to determine markup on products and services.
3. Science and Research:
- Representing experimental data: Scientific data is frequently represented using percentages to demonstrate proportions and trends.
- Calculating error margins: Statistical analysis often involves expressing the error margin in percentage terms.
- Expressing concentrations: Concentrations of solutions are commonly expressed as percentages.
4. Education and Assessments:
- Calculating grades: Students' performance is often assessed and reported using percentages.
- Analyzing test scores: Understanding the distribution of scores and identifying areas for improvement often requires percentage analysis.
- Evaluating learning outcomes: Tracking the progress of students requires the use of percentages to gauge the effectiveness of teaching methods.
Beyond the Basics: More Complex Percentage Problems
While calculating 32 out of 50 as a percentage is a relatively simple task, other percentage problems can be more complex. Here are a few examples:
Finding the Percentage Increase or Decrease
Suppose a value increases from 50 to 64. To calculate the percentage increase:
- Find the difference: 64 - 50 = 14
- Divide the difference by the original value: 14 / 50 = 0.28
- Multiply by 100 to express as a percentage: 0.28 x 100 = 28%
Therefore, there is a 28% increase from 50 to 64.
Finding a Percentage of a Number
To find, say, 64% of 50:
- Convert the percentage to a decimal: 64% = 0.64
- Multiply the decimal by the number: 0.64 x 50 = 32
Therefore, 64% of 50 is 32.
Working Backwards from a Percentage
Suppose a value has been reduced by 36% to 32. To find the original value:
- Let 'x' represent the original value.
- The reduced value is represented by x - 0.36x = 0.64x
- We know 0.64x = 32
- Solve for x: x = 32 / 0.64 = 50
Therefore, the original value was 50.
Mastering Percentage Calculations: Tips and Tricks
- Practice regularly: The more you practice, the more comfortable you'll become with percentage calculations.
- Use a calculator: For more complex calculations, using a calculator can save time and reduce errors.
- Understand the concepts: A strong understanding of the underlying concepts will make it easier to tackle more challenging problems.
- Break down complex problems: Divide complex problems into smaller, manageable steps.
- Check your work: Always double-check your answers to ensure accuracy.
Conclusion
Calculating percentages is a valuable skill with applications across numerous fields. Understanding how to convert fractions and decimals to percentages, as demonstrated with the example of 32 out of 50 being 64%, is essential for interpreting data, making informed decisions, and effectively communicating information. By mastering various percentage calculation techniques and practicing regularly, you can build confidence and proficiency in this crucial area of mathematics. The ability to confidently and accurately work with percentages will enhance your analytical skills and broaden your problem-solving capabilities, leading to a greater understanding of the world around you.
Latest Posts
Latest Posts
-
Cuanto Es 1 M En Pulgadas
Apr 07, 2025
-
How Many Inches In 84 Cm
Apr 07, 2025
-
18 Is What Percent Of 60
Apr 07, 2025
-
How Many Feet Are 25 Meters
Apr 07, 2025
-
28 Oz Is How Many Cups
Apr 07, 2025
Related Post
Thank you for visiting our website which covers about What Is 32 Out Of 50 As A Percentage . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.