What Is 5 To The Power Of 2
Kalali
Mar 28, 2025 · 5 min read
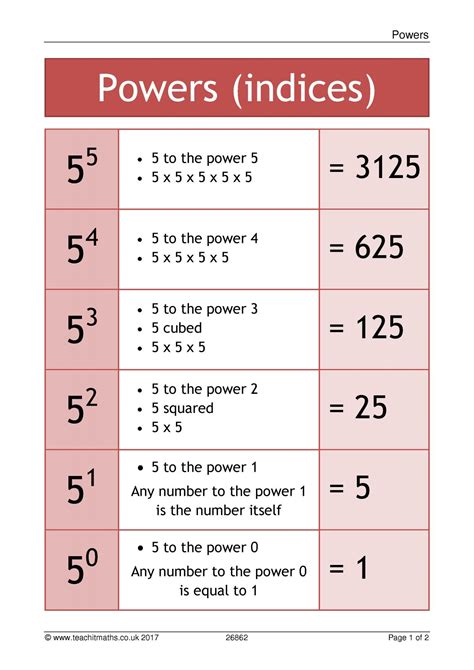
Table of Contents
What is 5 to the Power of 2? A Deep Dive into Exponents and Their Applications
The seemingly simple question, "What is 5 to the power of 2?" opens a door to a vast and fascinating world of mathematics – the realm of exponents. While the answer itself is straightforward (25), understanding the underlying concepts provides a strong foundation for more complex mathematical operations and their applications in various fields. This article will delve deep into the meaning of exponents, explore different ways to calculate 5², and discuss its significance in various contexts.
Understanding Exponents: The Basics
Exponents, also known as powers or indices, represent repeated multiplication. The expression 5² (pronounced "5 to the power of 2," "5 squared," or "5 raised to the power of 2") signifies 5 multiplied by itself two times. In general, the expression a<sup>b</sup> represents the base (a) multiplied by itself b times. Thus, 5² = 5 × 5 = 25.
Key Terminology:
- Base: The number being multiplied (in this case, 5).
- Exponent (or Power or Index): The number indicating how many times the base is multiplied by itself (in this case, 2).
- Result: The product obtained after performing the repeated multiplication (in this case, 25).
Calculating 5²: Different Approaches
While the calculation of 5² is simple, understanding different approaches can solidify the concept and prepare you for more challenging exponent problems.
1. Direct Multiplication:
This is the most straightforward method. Simply multiply the base (5) by itself the number of times indicated by the exponent (2): 5 × 5 = 25.
2. Using a Calculator:
Most calculators have an exponent function (usually denoted by a "^" symbol or a button labeled "x<sup>y</sup>"). Simply input 5, press the exponent function, enter 2, and press the equals sign to get the answer: 25.
3. Visual Representation:
Imagine a square with sides of length 5 units. The area of this square is calculated by multiplying the length of one side by itself: 5 × 5 = 25 square units. This visual representation demonstrates the geometric interpretation of squaring a number.
Beyond 5²: Exploring Higher Powers and Negative Exponents
Understanding 5² is crucial for grasping the broader concept of exponents. Let's explore further:
1. Higher Powers of 5:
- 5³ (5 to the power of 3 or 5 cubed) = 5 × 5 × 5 = 125
- 5⁴ (5 to the power of 4) = 5 × 5 × 5 × 5 = 625
- 5⁵ (5 to the power of 5) = 5 × 5 × 5 × 5 × 5 = 3125
Notice the rapid growth as the exponent increases. This illustrates the exponential nature of these calculations, which has significant implications in areas like compound interest and population growth.
2. Negative Exponents:
Negative exponents represent the reciprocal of the positive exponent. For instance:
- 5⁻¹ = 1/5 = 0.2
- 5⁻² = 1/5² = 1/25 = 0.04
- 5⁻³ = 1/5³ = 1/125 = 0.008
The value decreases as the absolute value of the negative exponent increases. Understanding negative exponents is critical in scientific notation and various mathematical applications.
3. Zero Exponent:
Any number (except 0) raised to the power of 0 equals 1. Therefore, 5⁰ = 1. This might seem counterintuitive but is a fundamental rule of exponents.
Applications of Exponents: Real-World Examples
The concept of "5 to the power of 2," and exponents in general, is far from an abstract mathematical exercise. It has widespread applications in numerous fields:
1. Geometry and Area Calculations:
As shown earlier, calculating the area of a square utilizes exponents. Similarly, the volume of a cube is found by cubing the length of its side (side³). Exponents are fundamental to geometric calculations involving shapes with similar dimensions.
2. Compound Interest:
Compound interest calculations heavily rely on exponents. The formula for compound interest involves raising the principal amount plus the interest rate to the power of the number of compounding periods. Understanding exponents is crucial for anyone managing investments or loans.
3. Scientific Notation:
Exponents are essential in scientific notation, which is used to represent extremely large or small numbers concisely. For example, the speed of light can be expressed as 3 x 10⁸ meters per second, where 10⁸ represents 10 raised to the power of 8.
4. Computer Science and Data Storage:
Computer data storage and memory are often expressed using powers of 2 (kilobytes, megabytes, gigabytes, etc.). Understanding binary numbers and their relation to powers of 2 is crucial in computer science.
5. Population Growth Models:
Exponential growth models, often expressed using exponential functions (functions involving exponents), are used to predict population growth. These models use exponents to represent the rapid increase in a population over time.
6. Physics and Engineering:
Many physical phenomena, such as radioactive decay and wave behavior, are described by exponential functions. Exponents are fundamental to many physics and engineering calculations.
Conclusion: The Significance of Understanding 5² and Exponents
While the answer to "What is 5 to the power of 2?" is simply 25, the underlying concepts and applications of exponents are vast and far-reaching. Mastering exponents forms a cornerstone for higher-level mathematics, scientific understanding, and practical applications across various disciplines. From calculating areas and volumes to understanding compound interest and population growth, the significance of exponents cannot be overstated. This deep dive into the seemingly simple calculation of 5² showcases the power and versatility of this fundamental mathematical concept. A thorough understanding of exponents is crucial for anyone seeking to enhance their mathematical skills and comprehension of the world around them. By grasping the concepts presented here, you'll be well-equipped to tackle more complex mathematical problems and appreciate the elegance and practicality of exponents in numerous contexts.
Latest Posts
Latest Posts
-
How Many Inches Is 30 Ft
Mar 31, 2025
-
What Is The Boiling Point Of Saltwater
Mar 31, 2025
-
What Are The 2 Parts Of A Solution
Mar 31, 2025
-
What Are The Chemical Equations Of Photosynthesis And Cellular Respiration
Mar 31, 2025
-
What Is 57 Celsius In Fahrenheit
Mar 31, 2025
Related Post
Thank you for visiting our website which covers about What Is 5 To The Power Of 2 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
