What Is 6 Out Of 7 As A Percentage
Kalali
Apr 17, 2025 · 5 min read
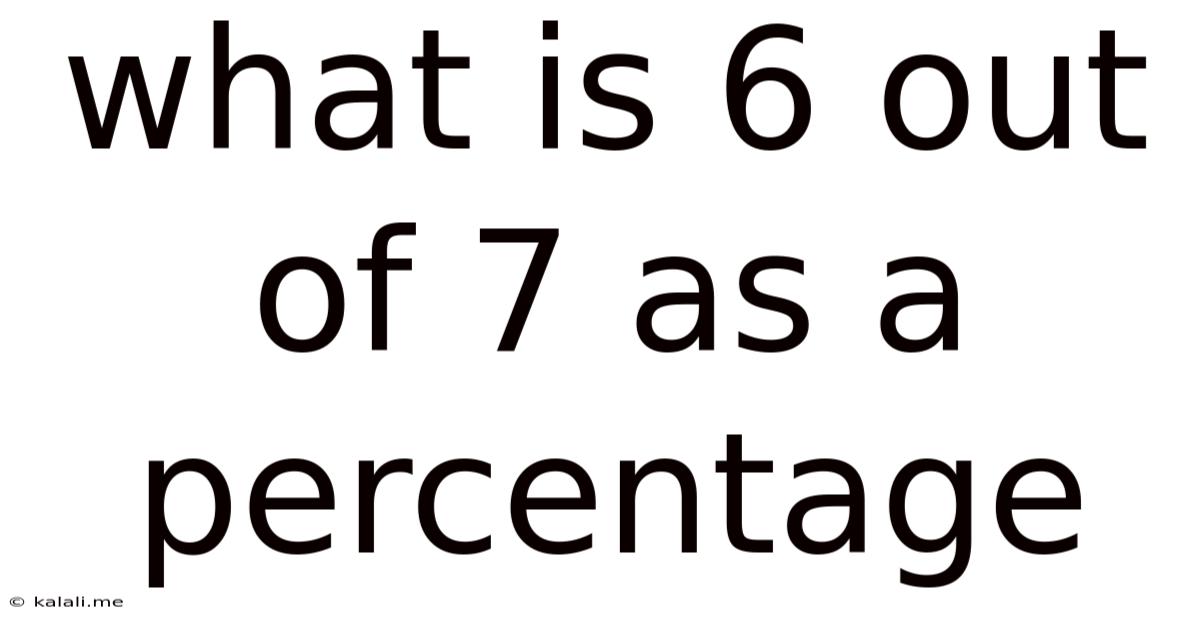
Table of Contents
What is 6 out of 7 as a Percentage? A Comprehensive Guide to Percentage Calculations
Understanding percentages is a fundamental skill in many aspects of life, from calculating discounts and taxes to comprehending statistical data and analyzing financial reports. This comprehensive guide will delve into the calculation of "6 out of 7 as a percentage," providing not only the answer but also a thorough explanation of the underlying methodology. We'll explore different methods for calculating percentages, address common misconceptions, and offer practical applications to solidify your understanding.
Meta Description: Learn how to calculate 6 out of 7 as a percentage. This detailed guide explains various methods, tackles common mistakes, and provides practical examples for mastering percentage calculations.
Understanding the Basics of Percentages
A percentage is a way of expressing a number as a fraction of 100. The word "percent" itself comes from the Latin "per centum," meaning "out of a hundred." Therefore, any percentage can be represented as a fraction with a denominator of 100. For example, 50% is equivalent to 50/100, which simplifies to 1/2.
This understanding is crucial for converting fractions into percentages. The core concept revolves around finding the equivalent fraction with a denominator of 100.
Method 1: Using the Fraction Method to Calculate 6 out of 7 as a Percentage
The simplest way to calculate 6 out of 7 as a percentage is to treat it as a fraction: 6/7. To convert this fraction into a percentage, we need to find an equivalent fraction with a denominator of 100. We can achieve this by following these steps:
-
Set up the equation: We start with the fraction 6/7. We want to find the percentage, which we'll represent as 'x%'. This can be written as:
6/7 = x/100
-
Solve for x: To solve for 'x', we cross-multiply:
6 * 100 = 7 * x 600 = 7x
-
Isolate x: Divide both sides by 7:
x = 600/7
-
Calculate the decimal: Performing the division, we get:
x ≈ 85.7142857
-
Convert to percentage: Finally, we express this decimal as a percentage by multiplying by 100:
x ≈ 85.71%
Therefore, 6 out of 7 is approximately 85.71%. The slight rounding is due to the fact that 6/7 is a recurring decimal.
Method 2: Using Decimal Conversion to Calculate 6 out of 7 as a Percentage
Another approach involves converting the fraction to a decimal first, then multiplying by 100 to express it as a percentage.
-
Convert the fraction to a decimal: Divide 6 by 7:
6 ÷ 7 ≈ 0.857142857
-
Multiply by 100: Multiply the decimal by 100 to convert it to a percentage:
0.857142857 * 100 ≈ 85.71%
This method yields the same result as the fraction method: approximately 85.71%.
Method 3: Using a Calculator for Percentage Calculation
Most calculators have a percentage function that simplifies the process significantly. Simply enter "6 ÷ 7 * 100" and the calculator will directly provide the percentage value of approximately 85.71%. This method is efficient and minimizes the risk of manual calculation errors.
Understanding Rounding and Significant Figures
As we've seen, the result of 6 out of 7 as a percentage is a recurring decimal. In practical applications, you'll need to round the result to a suitable number of decimal places. The level of precision needed depends on the context.
- Two decimal places: 85.71% is commonly used for general purposes.
- One decimal place: 85.7% provides a slightly less precise but still accurate representation.
- Three decimal places or more: Higher precision may be necessary for scientific or financial calculations where accuracy is paramount.
Common Mistakes in Percentage Calculations
Several common errors can occur when calculating percentages:
- Incorrect order of operations: Remember to follow the order of operations (PEMDAS/BODMAS). Division should be performed before multiplication when calculating percentages.
- Incorrect decimal placement: Ensure correct placement of the decimal point when converting decimals to percentages and vice-versa.
- Rounding errors: While rounding is necessary, be mindful of the potential for accumulated errors, especially in complex calculations.
Practical Applications of Percentage Calculations
Understanding percentage calculations is vital in various real-world situations:
- Calculating discounts: If an item is discounted by 20%, you can easily determine the final price.
- Determining tax amounts: Calculating sales tax or income tax involves percentage calculations.
- Analyzing financial statements: Financial reports often use percentages to represent ratios and trends.
- Evaluating performance metrics: Percentages are frequently employed to measure performance in areas like sports, academics, and business.
- Understanding statistical data: Many statistical analyses rely on percentages to represent proportions and probabilities.
Beyond the Basics: Advanced Percentage Calculations
While the calculation of 6 out of 7 as a percentage is relatively straightforward, more complex percentage problems may involve:
- Finding the original value: If you know the percentage and the resulting value, you can calculate the original value.
- Calculating percentage increase or decrease: This involves determining the percentage change between two values.
- Working with compound percentages: Compound percentages involve applying a percentage to a value multiple times, such as calculating compound interest.
Conclusion: Mastering Percentage Calculations for Everyday Use
Calculating percentages is an essential skill applicable across many disciplines. This guide has provided a detailed explanation of how to calculate 6 out of 7 as a percentage (approximately 85.71%), outlining different methods, addressing common errors, and illustrating practical applications. By mastering these fundamental concepts, you'll be better equipped to tackle various percentage-related challenges in your personal and professional life. Remember that practice is key to solidifying your understanding and increasing your calculation speed and accuracy. Continue practicing with different examples to reinforce your knowledge and build confidence in your ability to handle percentage problems effectively. The more you practice, the more intuitive percentage calculations will become.
Latest Posts
Latest Posts
-
9 As A Percentage Of 25
Apr 19, 2025
-
What Is 1 12 In A Decimal
Apr 19, 2025
-
Cuanto Es 39 Fahrenheit En Celsius
Apr 19, 2025
-
3 6 Amino Acids Per One Alpha Helix Turn
Apr 19, 2025
-
Is The Sun Biotic Or Abiotic
Apr 19, 2025
Related Post
Thank you for visiting our website which covers about What Is 6 Out Of 7 As A Percentage . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.