What Is 7 To The Power Of 2
Kalali
Apr 06, 2025 · 5 min read
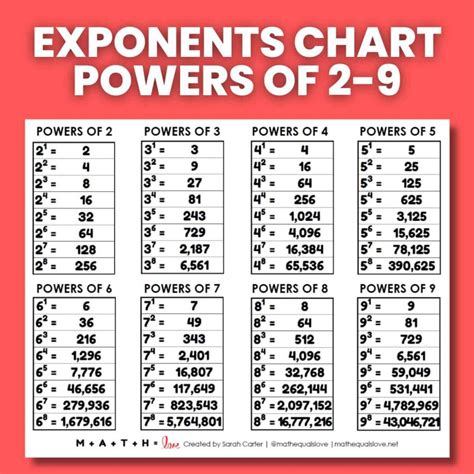
Table of Contents
What is 7 to the Power of 2? A Deep Dive into Exponents and Their Applications
The seemingly simple question, "What is 7 to the power of 2?" opens a door to a vast world of mathematical concepts, their practical applications, and their importance in various fields. While the answer itself is straightforward (49), exploring the underlying principles and expanding on the concept of exponents provides a richer understanding of mathematics and its relevance in our daily lives.
Understanding Exponents: The Fundamentals
In mathematics, an exponent (also known as a power or index) represents repeated multiplication. The expression "7 to the power of 2" (written as 7², 7<sup>2</sup>, or sometimes even 7**2 in programming languages) signifies 7 multiplied by itself two times: 7 × 7 = 49.
The general form of an exponential expression is b<sup>n</sup>, where:
- b is the base – the number being multiplied.
- n is the exponent – the number of times the base is multiplied by itself.
Therefore, 7² signifies that 7 (the base) is multiplied by itself 2 (the exponent) times.
Beyond the Basics: Exploring Higher Powers of 7
While 7² is easily calculated, understanding the concept allows us to tackle higher powers with ease. Let's explore a few:
- 7³ (7 to the power of 3): 7 × 7 × 7 = 343
- 7⁴ (7 to the power of 4): 7 × 7 × 7 × 7 = 2401
- 7⁵ (7 to the power of 5): 7 × 7 × 7 × 7 × 7 = 16807
Notice the rapid increase in the result as the exponent increases. This exponential growth is a characteristic feature of exponential functions and has significant implications in various real-world scenarios.
The Power of Zero and Negative Exponents
The concept of exponents extends beyond positive integers. Let's examine two special cases:
-
7⁰ (7 to the power of 0): Any non-zero number raised to the power of 0 is always equal to 1. Therefore, 7⁰ = 1. This might seem counterintuitive, but it's a fundamental rule in algebra that ensures consistency in mathematical operations.
-
7⁻¹ (7 to the power of -1): A negative exponent indicates the reciprocal of the base raised to the positive exponent. Therefore, 7⁻¹ = 1/7 = 0.142857...
-
7⁻² (7 to the power of -2): Similarly, 7⁻² = 1/7² = 1/49 = 0.020408...
Applications of Exponents in Real Life
The concept of exponents isn't confined to abstract mathematical exercises. It finds numerous applications in diverse fields:
1. Compound Interest Calculations:
Banks and financial institutions utilize exponential functions to calculate compound interest. Compound interest means earning interest on both the principal amount and accumulated interest. The formula for compound interest involves exponents:
A = P (1 + r/n)<sup>nt</sup>
Where:
- A = the future value of the investment/loan, including interest
- P = the principal investment amount (the initial deposit or loan amount)
- r = the annual interest rate (decimal)
- n = the number of times that interest is compounded per year
- t = the number of years the money is invested or borrowed for
Understanding exponents is crucial for comprehending how investments grow over time.
2. Population Growth and Decay:
Exponential functions model population growth (e.g., bacterial growth, human population) and decay (e.g., radioactive decay). The rate of growth or decay is proportional to the current population size. These models often involve exponential equations.
3. Scientific Notation:
Scientists and engineers frequently use exponential notation (scientific notation) to express very large or very small numbers concisely. For example, the speed of light is approximately 3 x 10⁸ meters per second. This is equivalent to 300,000,000 m/s.
4. Computer Science and Data Storage:
In computer science, exponents are crucial for understanding data storage capacity (e.g., kilobytes, megabytes, gigabytes, etc., which are based on powers of 2). Binary systems, the foundation of computers, rely heavily on powers of 2.
5. Physics and Engineering:
Many physical phenomena, such as radioactive decay, the growth of populations, and the propagation of waves, are described by exponential functions. Exponents are vital in understanding these processes. For example, in calculating the energy of a photon, Planck's constant and frequency use exponential relationships.
Expanding on the Concept: Exponential Functions
The expression 7² is a single point on a broader mathematical entity: the exponential function. An exponential function is a function of the form f(x) = a<sup>x</sup>, where 'a' is a positive constant (the base), and 'x' is the exponent (a variable). When 'a' is greater than 1, the function shows exponential growth. When 'a' is between 0 and 1, it shows exponential decay. Graphing these functions reveals their characteristic curves.
Beyond Base 7: Exploring Other Bases
While we've focused on base 7, the concept of exponents applies to any base. Let's consider some examples:
- 2² (2 to the power of 2): 2 × 2 = 4
- 3² (3 to the power of 2): 3 × 3 = 9
- 10² (10 to the power of 2): 10 × 10 = 100
- e² (e to the power of 2): Where 'e' is Euler's number (approximately 2.71828), e² ≈ 7.389
Euler's number (e) is a particularly important base in calculus and many other areas of mathematics and science. The exponential function with base e (e<sup>x</sup>) is often denoted as exp(x) and has unique mathematical properties.
Logarithms: The Inverse of Exponents
Logarithms are the inverse operation of exponentiation. If 7² = 49, then the logarithm base 7 of 49 is 2 (written as log₇49 = 2). Logarithms are used extensively in various fields to solve equations involving exponents, analyze data with exponential trends, and simplify complex calculations. They are particularly useful when dealing with large or small numbers.
Conclusion: The Significance of 7² and Beyond
While the answer to "What is 7 to the power of 2?" is simply 49, delving into the broader context of exponents reveals a powerful mathematical tool with vast applications in various fields. Understanding exponents is not just about performing calculations; it's about understanding fundamental principles that govern growth, decay, and many other phenomena in our world. From financial calculations to scientific models, exponents provide a crucial framework for comprehending and analyzing the world around us. The seemingly simple 7² serves as a gateway to a much deeper and more significant understanding of mathematics and its role in our lives.
Latest Posts
Latest Posts
-
What Is 4 Celsius In Fahrenheit
Apr 06, 2025
-
2 Out Of 30 Is What Percent
Apr 06, 2025
-
Como Se Escribe El 0 En Numeros Romanos
Apr 06, 2025
-
1 Out Of 40 As A Percentage
Apr 06, 2025
-
What Is Ten Percent Of 50000
Apr 06, 2025
Related Post
Thank you for visiting our website which covers about What Is 7 To The Power Of 2 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.