What Is Ten Percent Of 50000
Kalali
Apr 06, 2025 · 5 min read
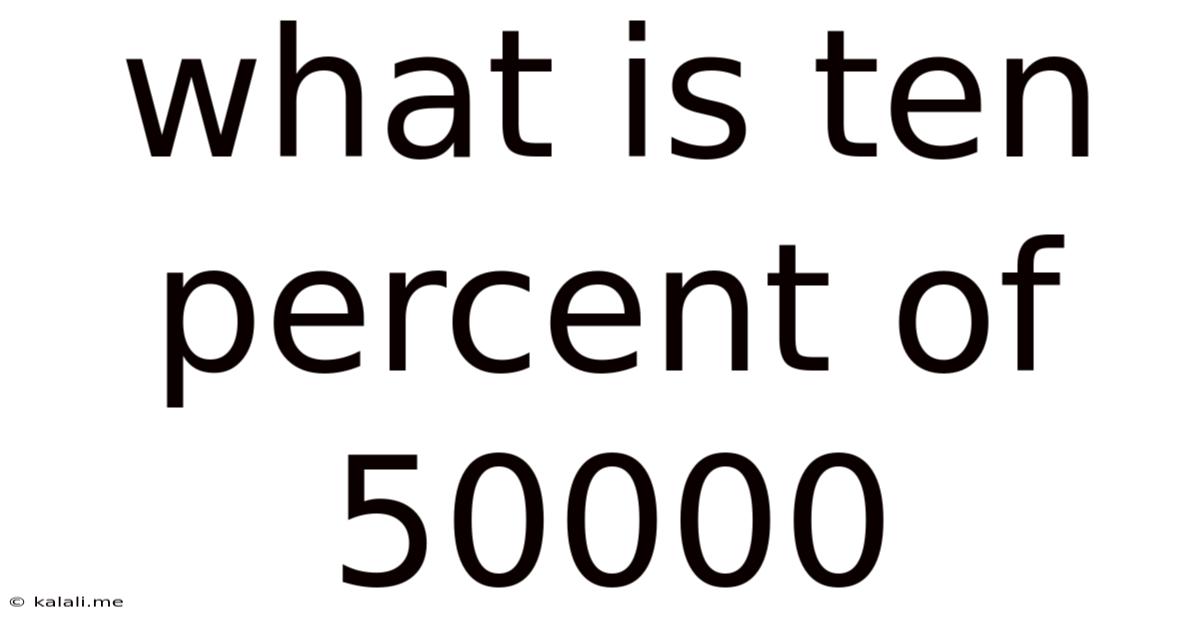
Table of Contents
What is Ten Percent of 50,000? A Comprehensive Guide to Percentages
Calculating percentages is a fundamental skill applicable across numerous areas of life, from financial planning and budgeting to understanding sales discounts and analyzing statistical data. This comprehensive guide will not only answer the question, "What is ten percent of 50,000?" but will also equip you with the knowledge and tools to confidently tackle any percentage calculation.
Understanding Percentages
Before diving into the specific calculation, let's establish a solid understanding of percentages. A percentage is simply a fraction expressed as a portion of 100. The symbol "%" represents "per hundred" or "out of 100." For example, 50% means 50 out of 100, or 50/100, which simplifies to 1/2 or 0.5.
Understanding this fundamental concept allows us to easily translate percentages into decimals or fractions, and vice versa, which is crucial for calculating percentages of numbers.
Method 1: The Decimal Method
This is arguably the most straightforward method for calculating percentages. It involves converting the percentage to a decimal and then multiplying it by the number.
Step 1: Convert the percentage to a decimal.
To convert a percentage to a decimal, simply divide the percentage by 100. In our case:
10% / 100 = 0.10
Step 2: Multiply the decimal by the number.
Now, multiply the decimal (0.10) by the number you're finding the percentage of (50,000):
0.10 * 50,000 = 5,000
Therefore, 10% of 50,000 is 5,000.
This method is efficient and easily adaptable to calculating any percentage of any number.
Method 2: The Fraction Method
This method utilizes the fractional representation of percentages.
Step 1: Express the percentage as a fraction.
10% can be expressed as the fraction 10/100. This fraction can be simplified to 1/10.
Step 2: Multiply the fraction by the number.
Now, multiply the simplified fraction (1/10) by the number (50,000):
(1/10) * 50,000 = 50,000 / 10 = 5,000
Therefore, 10% of 50,000 is 5,000. This method reinforces the understanding of percentages as fractions.
Method 3: Using Proportions
This method uses the concept of ratios and proportions. It's a more versatile method useful for solving more complex percentage problems.
Step 1: Set up a proportion.
We can set up a proportion as follows:
x / 50,000 = 10 / 100
Where 'x' represents the unknown value (10% of 50,000).
Step 2: Solve for x.
To solve for x, we cross-multiply:
100x = 10 * 50,000
100x = 500,000
x = 500,000 / 100
x = 5,000
Therefore, 10% of 50,000 is 5,000. This method provides a structured approach to solving percentage problems, especially those involving unknowns.
Real-World Applications: Why Understanding Percentages Matters
The ability to calculate percentages is crucial in many aspects of daily life. Consider these examples:
-
Financial Planning: Calculating interest on loans or savings accounts, determining the percentage of income allocated to savings, or understanding tax rates all involve percentage calculations. Knowing that 10% of your $50,000 savings is $5,000 can significantly impact your budgeting and financial decisions.
-
Sales and Discounts: Retailers frequently offer discounts expressed as percentages. Understanding how to calculate these discounts allows you to determine the actual price after the discount. For instance, a 10% discount on a $50,000 car would be $5,000, leaving the final price at $45,000.
-
Statistics and Data Analysis: Percentages are used extensively in data analysis to represent proportions and trends. Understanding percentages allows you to interpret data effectively and draw meaningful conclusions. For example, if 10% of 50,000 survey respondents answered "yes" to a particular question, it means 5,000 respondents answered affirmatively.
-
Tip Calculation: Calculating a tip in a restaurant is a common application of percentages. If you want to leave a 10% tip on a $50,000 bill (perhaps a very lavish dinner!), the tip would be $5,000.
-
Commission Calculation: Salespeople often earn a commission based on a percentage of their sales. If a salesperson earns a 10% commission on $50,000 worth of sales, they would earn $5,000.
These examples highlight the practical relevance of mastering percentage calculations.
Beyond the Basics: Calculating Other Percentages of 50,000
While we've focused on 10%, the methods outlined can be applied to calculate any percentage of 50,000. Let's look at a few examples:
- 25% of 50,000: Using the decimal method: 0.25 * 50,000 = 12,500
- 5% of 50,000: Using the fraction method: (5/100) * 50,000 = 2,500
- 75% of 50,000: Using the proportion method: x / 50,000 = 75 / 100; x = 37,500
Troubleshooting Common Percentage Calculation Mistakes
Even simple percentage calculations can sometimes lead to errors. Here are some common mistakes to avoid:
-
Incorrect Decimal Conversion: Ensure you correctly convert the percentage to a decimal before multiplying. A common mistake is forgetting to divide by 100.
-
Incorrect Multiplication/Division: Double-check your multiplication and division calculations to avoid simple arithmetic errors.
-
Confusing Percentage and Decimal: Remember the difference between a percentage and a decimal. 10% is not equal to 10; it's equal to 0.10.
Conclusion: Mastering Percentage Calculations for Success
Understanding how to calculate percentages is a valuable skill that extends far beyond the classroom. From managing your personal finances to analyzing data in your professional life, the ability to quickly and accurately calculate percentages can significantly enhance your decision-making abilities and problem-solving skills. By mastering the different methods presented in this guide, you'll be well-equipped to confidently tackle any percentage calculation, no matter the context. Remember the fundamental principle: a percentage is simply a fraction out of 100, and armed with that understanding, you can tackle any percentage problem with ease. Therefore, to reiterate the answer to the initial question: 10% of 50,000 is 5,000.
Latest Posts
Latest Posts
-
What Are Producers In An Ecosystem
Apr 09, 2025
-
How Many Feet Is 166 Cm
Apr 09, 2025
-
What Is 50 Ml In Cups
Apr 09, 2025
-
Energy From Moving Air Is Produced By
Apr 09, 2025
-
10 Out Of 16 As A Percentage
Apr 09, 2025
Related Post
Thank you for visiting our website which covers about What Is Ten Percent Of 50000 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.