What Is 80 Percent Of 15
Kalali
Mar 30, 2025 · 5 min read
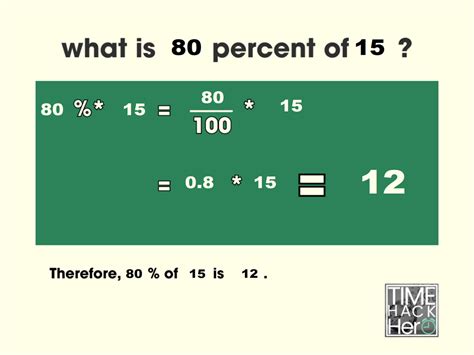
Table of Contents
What is 80 Percent of 15? A Deep Dive into Percentage Calculations
This seemingly simple question, "What is 80 percent of 15?", opens the door to a fascinating exploration of percentage calculations, their real-world applications, and the underlying mathematical principles. While the answer itself is straightforward, understanding the how and why behind the calculation is crucial for anyone seeking to master percentages and their pervasive use in various fields. This comprehensive guide will not only answer the question but also equip you with the tools and knowledge to tackle any percentage problem with confidence.
Understanding Percentages: The Foundation
Percentages are a fundamental concept in mathematics representing a fraction of 100. The term "percent" literally means "out of one hundred" ( per cent ). Therefore, 80 percent signifies 80 parts out of 100 equal parts. This fractional representation is key to understanding how percentage calculations work. We can express 80 percent as a fraction: 80/100, which simplifies to 4/5. This simplified fraction provides an alternative method for calculating 80% of 15.
Calculating 80% of 15: The Methods
There are several approaches to calculating 80% of 15. Each method offers a different perspective and can be useful depending on the context and your mathematical comfort level.
Method 1: The Direct Multiplication Method
This is perhaps the most straightforward method. We convert the percentage to a decimal by dividing it by 100. 80% becomes 0.80 (or simply 0.8). Then, we multiply this decimal by the number we're finding the percentage of:
0.8 * 15 = 12
Therefore, 80% of 15 is 12.
Method 2: The Fractional Method
As mentioned earlier, 80% can be expressed as the fraction 4/5. This allows us to solve the problem using fractions:
(4/5) * 15 = (4 * 15) / 5 = 60 / 5 = 12
This method demonstrates the equivalence between percentages and fractions, offering an alternative approach to reach the same solution: 12.
Method 3: Breaking it Down
We can break down the calculation into smaller, more manageable steps. For instance, we can find 10% of 15 first:
10% of 15 = 0.1 * 15 = 1.5
Since 80% is eight times 10%, we multiply the result by 8:
1.5 * 8 = 12
This method is helpful for mental calculations or when dealing with less convenient percentages. Again, the answer is 12.
Real-World Applications of Percentage Calculations
The ability to calculate percentages is essential in a wide variety of real-world scenarios. Here are just a few examples:
-
Sales and Discounts: Retail stores frequently offer discounts expressed as percentages. Calculating the final price after a discount involves percentage calculations. For example, a 20% discount on a $50 item is easily calculated using the methods discussed above.
-
Taxes: Sales tax, income tax, and other taxes are usually expressed as percentages of the taxable amount. Understanding percentage calculations is crucial for accurately determining the total cost of goods or services and for managing personal finances effectively.
-
Tips and Gratuities: When dining out or receiving services, it's customary to leave a tip, often expressed as a percentage of the bill. Quickly calculating a 15% or 20% tip requires proficiency in percentage calculations.
-
Interest Rates: Interest rates on loans, mortgages, and savings accounts are expressed as percentages. Understanding these percentages is vital for making informed financial decisions and managing debt.
-
Investment Returns: Investors track their returns as percentages of their initial investment. Calculating percentage returns helps assess the performance of different investment strategies.
-
Statistics and Data Analysis: Percentages are frequently used in statistics to represent proportions, probabilities, and changes in data. Understanding percentages is crucial for interpreting statistical data accurately.
-
Scientific Research: Percentage calculations are commonly employed in scientific research to represent data, proportions, and errors.
Advanced Percentage Problems and Concepts
While calculating 80% of 15 is relatively simple, percentage problems can become more complex. Let's explore some of these:
-
Finding the Percentage: Sometimes, you might know the original amount and the resulting amount after a percentage increase or decrease, and you need to find the percentage change. This requires setting up and solving an equation.
-
Percentage Increase and Decrease: These calculations involve finding the new amount after an increase or decrease by a specific percentage. Understanding the difference between these calculations is essential.
-
Compound Interest: Compound interest involves earning interest on both the principal amount and accumulated interest. Calculating compound interest requires more complex formulas but relies on the fundamental principles of percentage calculations.
-
Percentage Points: It's important to distinguish between percentage points and percentages. A change from 10% to 20% is a 10 percentage point increase, but it represents a 100% increase relative to the initial value.
Mastering Percentages: Tips and Strategies
To become proficient in percentage calculations, consider these tips:
-
Practice Regularly: The more you practice, the better you'll become. Start with simple problems and gradually move to more complex ones.
-
Understand the Concepts: Don't just memorize formulas; understand the underlying principles. This will make it easier to solve different types of percentage problems.
-
Use Multiple Methods: Experiment with different methods to find the approach that suits you best. Some methods might be more efficient for certain types of problems.
-
Utilize Online Resources: There are many online resources, including calculators and tutorials, that can help you learn and practice percentage calculations.
-
Break Down Complex Problems: Large or complex problems can be broken down into smaller, more manageable steps.
-
Check Your Work: Always check your answers to ensure accuracy. Use a different method to verify your results.
Conclusion: The Significance of Percentage Calculations
The seemingly simple calculation of 80% of 15 underscores the importance of understanding percentages in our daily lives. From managing finances and making informed consumer choices to interpreting data and succeeding in academic and professional settings, proficiency in percentage calculations is a valuable skill with far-reaching applications. By mastering the techniques and principles discussed in this guide, you will be well-equipped to tackle any percentage problem with confidence and apply this fundamental mathematical concept to a wide range of real-world situations. Remember, consistent practice and a solid understanding of the underlying principles are the keys to mastering percentages and unlocking their practical applications.
Latest Posts
Latest Posts
-
How Many Feet Are In 49 Inches
Apr 01, 2025
-
Use The Graph To Write An Equation Of The Line
Apr 01, 2025
-
8 Out Of 120 As A Percentage
Apr 01, 2025
-
What Percent Is 11 Of 15
Apr 01, 2025
-
How Many Electrons Do Chlorine Have
Apr 01, 2025
Related Post
Thank you for visiting our website which covers about What Is 80 Percent Of 15 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
