What Is 9 To The Power Of 2
Kalali
Apr 13, 2025 · 5 min read
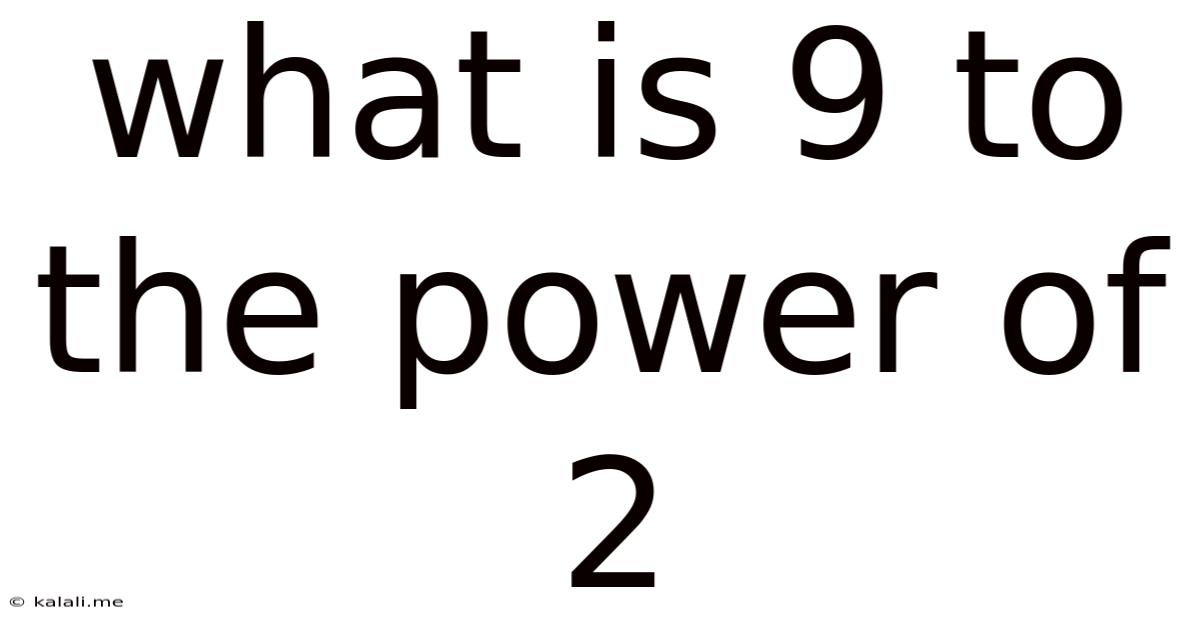
Table of Contents
What is 9 to the Power of 2? A Deep Dive into Exponents and Their Applications
What is 9 to the power of 2? The simple answer is 81. But this seemingly straightforward question opens the door to a much broader exploration of exponents, their mathematical properties, and their widespread applications across various fields. This article will delve into the meaning of exponents, explain how to calculate 9² and similar expressions, and explore the significance of this fundamental concept in mathematics and beyond.
Meta Description: Discover the answer to "What is 9 to the power of 2?" and explore the world of exponents, their mathematical properties, and their practical applications in various fields. Learn how to calculate exponential expressions and understand their significance in science, technology, and finance.
Understanding Exponents
Exponents, also known as powers or indices, represent repeated multiplication. The expression a<sup>b</sup> signifies that the base a is multiplied by itself b times. In our case, 9 to the power of 2 (written as 9²) means 9 multiplied by itself twice: 9 x 9 = 81.
The number 9 is the base, and the number 2 is the exponent or power. Understanding this fundamental definition is crucial for grasping more complex exponential operations.
Calculating 9² and Other Exponential Expressions
Calculating 9² is straightforward, as shown above. However, let's expand our understanding to encompass other exponential calculations:
-
Positive Integer Exponents: These are the most basic type of exponent, representing repeated multiplication. For example, 5³ = 5 x 5 x 5 = 125.
-
Zero Exponent: Any non-zero number raised to the power of zero equals 1. For example, 9⁰ = 1. This might seem counterintuitive, but it's a consistent rule within the mathematical framework of exponents.
-
Negative Exponents: A negative exponent indicates a reciprocal. For instance, 9⁻² = 1/9² = 1/81. This means the base is inverted and raised to the positive power.
-
Fractional Exponents: These exponents represent roots. For example, 9^(1/2) is the square root of 9, which is 3. Similarly, 8^(1/3) is the cube root of 8, which is 2. A fractional exponent like 9^(3/2) can be interpreted as (9^(1/2))³ = 3³ = 27.
-
Decimal Exponents: Decimal exponents can be handled by converting them into fractions or using a calculator. For example, 9^0.5 is the same as 9^(1/2), which is 3.
These rules provide a comprehensive approach to calculating exponential expressions, regardless of the nature of the exponent.
Applications of Exponents
The concept of exponents extends far beyond simple mathematical calculations. Its applications are pervasive across various disciplines:
1. Science and Engineering
-
Exponential Growth and Decay: Exponents are fundamental in describing phenomena exhibiting exponential growth (e.g., bacterial population growth, compound interest) or decay (e.g., radioactive decay, drug metabolism). Understanding exponential functions is crucial for modeling these processes accurately. The formula for compound interest, for example, relies heavily on exponential functions.
-
Physics: Exponents appear frequently in physics equations. For instance, Newton's Law of Universal Gravitation involves an inverse square law (an exponent of -2), describing the force between two masses. Similarly, many equations in electromagnetism and quantum mechanics utilize exponential functions.
-
Engineering: Exponential functions are used in various engineering disciplines, including electrical engineering (circuit analysis), mechanical engineering (vibration analysis), and chemical engineering (reaction kinetics).
2. Finance and Economics
-
Compound Interest: As mentioned earlier, compound interest calculations rely heavily on exponential functions. Understanding how exponents influence compound interest is critical for financial planning and investment strategies.
-
Economic Growth Models: Economic growth models often employ exponential functions to represent the growth of an economy over time.
-
Inflation Calculations: Inflation's impact on the value of money over time can be modeled using exponential functions.
3. Computer Science
-
Algorithm Analysis: Exponents are used to describe the time and space complexity of algorithms. This is crucial for understanding the efficiency and scalability of computer programs. For example, an algorithm with O(n²) time complexity indicates that the execution time grows quadratically with the input size.
-
Data Structures: The analysis of data structures, such as trees and graphs, often involves exponential concepts.
-
Cryptography: Exponential functions play a significant role in modern cryptography, particularly in public-key cryptography systems such as RSA.
4. Biology
-
Population Growth: The growth of populations (bacteria, animals, humans) can often be modeled using exponential functions, particularly during periods of rapid growth.
-
Genetics: Exponential concepts are relevant in genetic studies involving DNA replication and population genetics.
5. Everyday Life
While not always explicitly noticed, exponents are present in many aspects of everyday life. For instance:
-
Area Calculations: The area of a square is calculated by squaring the length of its side (side²).
-
Volume Calculations: The volume of a cube is calculated by cubing the length of its side (side³).
-
Digital Data Storage: Data storage capacity is often expressed in powers of 2 (kilobytes, megabytes, gigabytes, etc.), reflecting the binary nature of computer systems.
Beyond 9²: Exploring Larger Exponents
While we’ve focused primarily on 9², the principles discussed apply to calculating any base raised to any power. Consider these examples:
-
9³ (9 to the power of 3): This means 9 x 9 x 9 = 729.
-
9⁴ (9 to the power of 4): This means 9 x 9 x 9 x 9 = 6561.
-
9<sup>10</sup> (9 to the power of 10): This results in a much larger number (3486784401). Calculating such large exponents often requires the use of calculators or computer software.
Calculating larger exponents highlights the rapid growth inherent in exponential functions. This growth explains why exponential processes, whether growth or decay, can have such dramatic effects over time.
Conclusion
The answer to "What is 9 to the power of 2?" is 81. However, this simple calculation serves as a gateway to understanding the broader concept of exponents, their mathematical properties, and their wide-ranging applications across diverse fields. From scientific modeling and financial calculations to computer algorithms and everyday measurements, exponents are an essential part of our understanding of the world around us. Mastering exponential concepts is crucial for success in various academic and professional pursuits. The power of exponents goes far beyond a simple numerical answer; it empowers us to model, understand, and predict complex phenomena.
Latest Posts
Latest Posts
-
Convert 33 Degrees Farenheit To Celcius
Apr 13, 2025
-
What Percent Of 15 Is 75
Apr 13, 2025
-
230 Degrees Celsius Is What In Fahrenheit
Apr 13, 2025
-
Lowest Common Multiple Of 4 And 18
Apr 13, 2025
-
What Forces Control The Motion Of Everyday Objects
Apr 13, 2025
Related Post
Thank you for visiting our website which covers about What Is 9 To The Power Of 2 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.