What Is The Percent Of 2 7
Kalali
Apr 26, 2025 · 5 min read
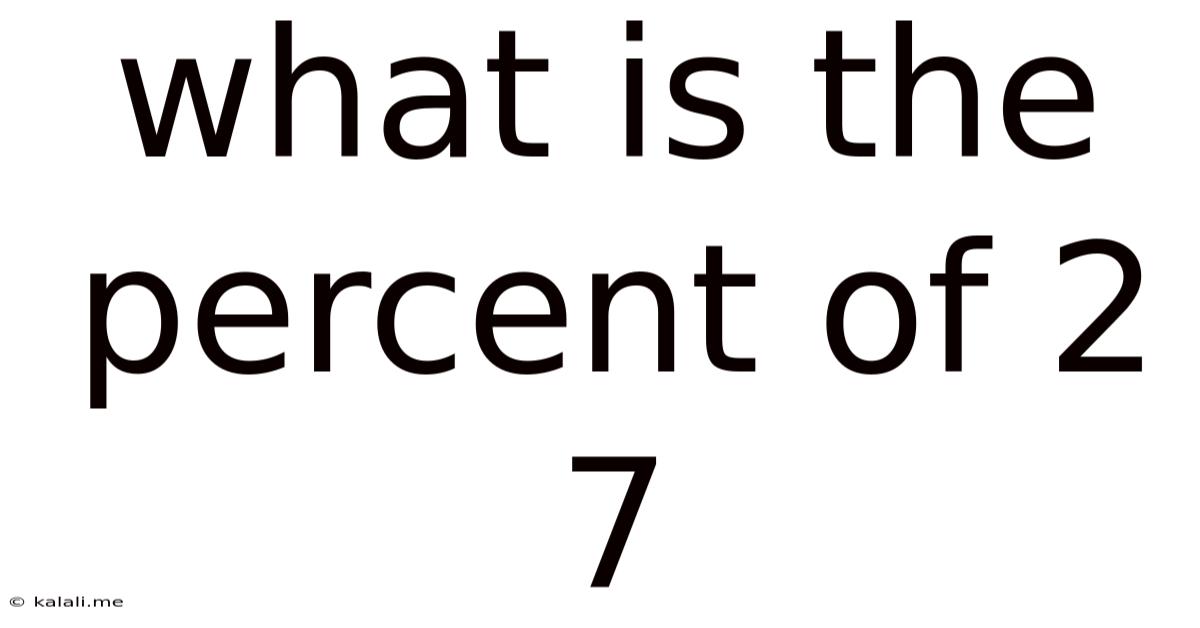
Table of Contents
What is the Percent of 2/7? A Deep Dive into Fractions, Decimals, and Percentages
This article explores the seemingly simple question: "What is the percent of 2/7?" While the calculation itself is straightforward, the process reveals fundamental concepts in mathematics crucial for understanding percentages, fractions, and decimals – concepts vital in numerous fields, from everyday budgeting to advanced scientific calculations. We'll delve into the calculation, explore various methods, and discuss the broader applications of this type of problem.
Understanding the Fundamentals: Fractions, Decimals, and Percentages
Before diving into the calculation, let's refresh our understanding of the three core mathematical concepts involved:
-
Fractions: A fraction represents a part of a whole. It's expressed as a ratio of two numbers, the numerator (top number) and the denominator (bottom number). 2/7 means 2 parts out of a total of 7 parts.
-
Decimals: Decimals are another way to represent parts of a whole. They use a base-ten system, with digits to the right of the decimal point representing tenths, hundredths, thousandths, and so on. Decimals are particularly useful for calculations and comparisons.
-
Percentages: A percentage is a fraction or decimal expressed as a portion of 100. The symbol "%" represents "per hundred." Percentages are commonly used to express proportions, rates, and changes. They are easily understood and used in everyday life.
Calculating the Percentage: From Fraction to Percent
To find the percentage equivalent of 2/7, we need to convert the fraction into a decimal and then multiply by 100. Here's the step-by-step process:
-
Divide the numerator by the denominator: This gives us the decimal representation of the fraction. 2 divided by 7 is approximately 0.2857.
-
Multiply the decimal by 100: This converts the decimal to a percentage. 0.2857 multiplied by 100 is 28.57%.
Therefore, 2/7 is approximately 28.57%.
Different Methods and Levels of Precision
While the above method is straightforward, there are other approaches to calculating the percentage, offering different levels of precision:
-
Long Division: For a more precise answer, performing long division of 2 by 7 will yield a longer decimal value. The more decimal places you calculate, the more precise your percentage will be. For example, using a calculator, we get 0.2857142857... Multiplying this by 100 gives us a more accurate percentage, but for most practical purposes, 28.57% is sufficient.
-
Using a Calculator: Calculators simplify the process significantly. Simply enter 2 ÷ 7 × 100 to get the percentage directly. Calculators offer greater precision and speed compared to manual calculations.
-
Approximation: In certain contexts, an approximate percentage might suffice. For instance, you might round 28.57% to 29%. The level of accuracy needed depends on the application.
Practical Applications and Real-World Examples
The ability to convert fractions to percentages has numerous practical applications across various fields:
-
Finance: Calculating interest rates, discounts, profit margins, and tax rates all involve working with percentages. For instance, if you receive a 2/7 discount on a product, understanding the percentage equivalent allows you to easily calculate the actual discount amount.
-
Statistics and Data Analysis: Percentages are crucial for representing proportions and probabilities in statistical analysis. They help communicate findings clearly and concisely, making data more accessible to a wider audience.
-
Science and Engineering: Percentages are used to express concentrations, efficiencies, and errors in scientific measurements and engineering designs.
-
Everyday Life: From calculating tips in restaurants to understanding sale discounts in stores, percentages are an integral part of our daily lives.
Expanding on the Concept: Working with More Complex Fractions and Percentages
The principles discussed above can be extended to more complex scenarios:
-
Improper Fractions: If the numerator is larger than the denominator (an improper fraction), the resulting percentage will be greater than 100%.
-
Mixed Numbers: A mixed number (a whole number combined with a fraction) needs to be converted to an improper fraction before calculating the percentage.
-
Percentage Increase/Decrease: Calculations often involve determining the percentage increase or decrease between two values. This requires understanding the difference between the two values and expressing it as a percentage of the original value.
-
Compounding Percentages: In some situations, percentages are compounded – meaning a percentage is calculated on a value that has already had a percentage applied to it. This is common in compound interest calculations.
Beyond the Calculation: The Importance of Understanding the Underlying Concepts
While the act of calculating the percentage equivalent of 2/7 is relatively simple, the underlying concepts of fractions, decimals, and percentages are far-reaching and important. A strong grasp of these concepts allows for:
-
Problem-Solving: The ability to confidently convert between these forms enhances problem-solving skills in various mathematical and real-world situations.
-
Data Interpretation: Understanding percentages enables effective interpretation of data presented in various forms, from charts and graphs to statistical reports.
-
Critical Thinking: The process of working with fractions, decimals, and percentages fosters critical thinking skills by requiring careful analysis and attention to detail.
Conclusion: Mastering Percentages for a Broader Mathematical Understanding
The question, "What is the percent of 2/7?", serves as a gateway to a deeper understanding of fundamental mathematical concepts. By mastering the conversion between fractions, decimals, and percentages, individuals equip themselves with essential tools for navigating the quantitative aspects of daily life and various professional fields. Beyond the specific calculation, the process reinforces the importance of precision, accuracy, and the power of understanding the underlying mathematical principles behind seemingly simple questions. Understanding these concepts is crucial for success in numerous academic and professional pursuits. The seemingly simple problem of converting 2/7 into a percentage showcases the broader importance of mathematical literacy and problem-solving skills.
Latest Posts
Latest Posts
-
8 Mm Is Equal To How Many Inches
Apr 27, 2025
-
32 Oz Equals How Many Liters
Apr 27, 2025
-
What Is 6 Out Of 8 In Percentage
Apr 27, 2025
-
98 6 F Is What In Celsius
Apr 27, 2025
-
What Is The Decimal For 12
Apr 27, 2025
Related Post
Thank you for visiting our website which covers about What Is The Percent Of 2 7 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.