What Percent Is 13 Of 15
Kalali
Apr 11, 2025 · 5 min read
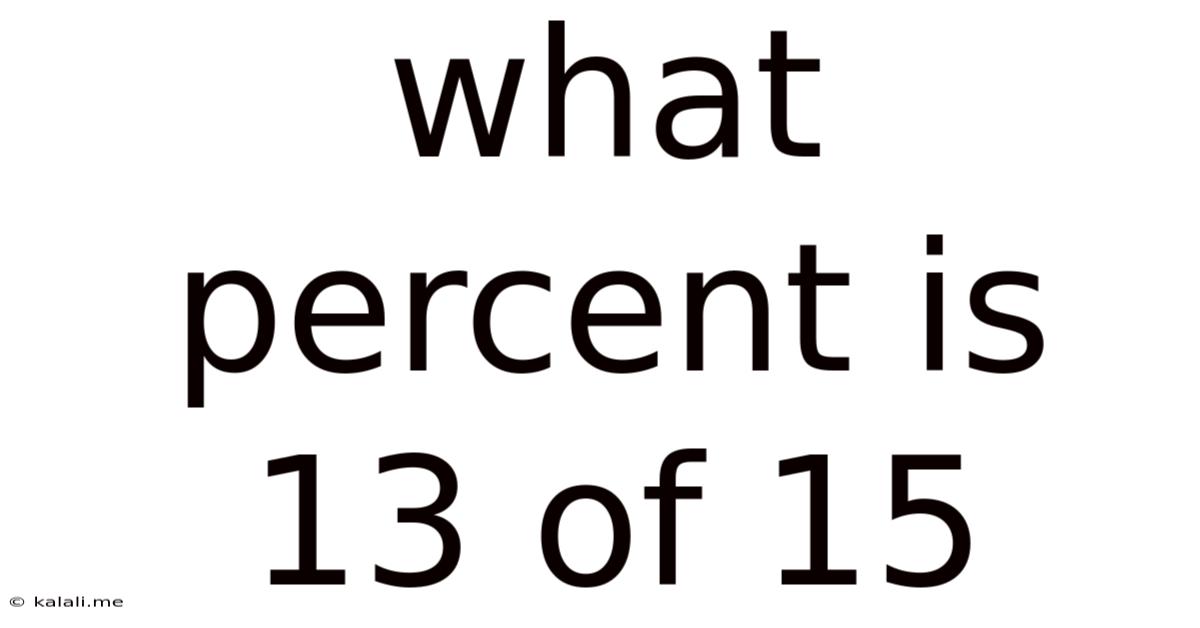
Table of Contents
What Percent is 13 of 15? A Deep Dive into Percentage Calculations and Real-World Applications
What percent is 13 of 15? This seemingly simple question opens the door to a broader understanding of percentages, their calculations, and their widespread applications in everyday life and various fields. This article will not only answer the initial question but also explore the underlying principles, offer different calculation methods, and delve into practical examples showcasing the significance of percentage calculations.
Meta Description: Learn how to calculate percentages with a detailed explanation of what percent 13 is of 15. This guide covers various methods, real-world applications, and helps you master percentage calculations.
Understanding Percentages: The Basics
Before diving into the specific calculation, let's establish a firm grasp on the fundamental concept of percentages. A percentage represents a fraction of 100. The word "percent" itself is derived from the Latin "per centum," meaning "out of one hundred." Therefore, any percentage can be expressed as a fraction with a denominator of 100 or as a decimal. For instance, 50% is equivalent to 50/100, which simplifies to 1/2, or 0.5 as a decimal.
This fundamental understanding is crucial for solving percentage problems. It forms the basis for converting between percentages, fractions, and decimals – skills essential for various calculations.
Method 1: The Proportion Method
This method uses the concept of proportions to solve the percentage problem. We set up a proportion where the part (13) is related to the whole (15) as the percentage (x) is related to 100.
The proportion can be written as:
13/15 = x/100
To solve for x, we cross-multiply:
13 * 100 = 15 * x
1300 = 15x
x = 1300 / 15
x ≈ 86.67
Therefore, 13 is approximately 86.67% of 15.
Method 2: The Decimal Method
This method involves converting the fraction 13/15 into a decimal and then multiplying by 100 to obtain the percentage.
First, we divide 13 by 15:
13 ÷ 15 ≈ 0.8667
Next, we multiply the decimal by 100 to express it as a percentage:
0.8667 * 100 ≈ 86.67%
This method provides the same result as the proportion method, confirming the accuracy of our calculations.
Method 3: Using a Calculator
Modern calculators simplify percentage calculations significantly. Most calculators have a percentage function (%) that directly computes percentages. Simply enter 13 ÷ 15 and then multiply the result by 100. The calculator will automatically handle the decimal conversion and provide the result as a percentage. This method is efficient and minimizes the risk of manual calculation errors, especially for more complex percentage problems.
Understanding the Result: 86.67%
The result, 86.67%, indicates that 13 represents approximately 86.67 out of every 100 parts of 15. This signifies a significant portion of the whole. The use of the approximation symbol (≈) highlights that the result is a rounded value. Depending on the context, you might need to round the percentage to a whole number (87%) or retain more decimal places for greater accuracy.
Real-World Applications of Percentage Calculations
Percentage calculations are not confined to mathematical exercises; they are deeply ingrained in various aspects of daily life and professional fields. Understanding percentages is essential for:
-
Financial Calculations: Interest rates, discounts, taxes, profit margins, and investment returns are all expressed as percentages. Calculating these accurately is crucial for making informed financial decisions. For instance, understanding a 15% discount on a $100 item involves calculating 15% of $100, which is $15. This knowledge helps you determine the final price.
-
Data Analysis and Statistics: Percentages are extensively used to present and interpret data. For example, surveys, polls, and market research often present findings using percentages to illustrate trends and patterns. Understanding the percentage of respondents who prefer a certain product or have a particular opinion is vital for drawing meaningful conclusions.
-
Scientific Applications: Percentages are used to represent concentrations, efficiencies, and error margins in various scientific fields, from chemistry to physics. For example, the percentage composition of a chemical compound or the efficiency of a machine are expressed as percentages.
-
Academic Performance: Grade point averages (GPAs) are often represented as percentages, reflecting a student's academic performance. Understanding GPA percentages helps students assess their progress and identify areas needing improvement.
-
Everyday Life: Tips in restaurants, sales tax, and even the nutritional content of food are often expressed as percentages. Understanding percentages in these situations enables us to make informed choices and manage our finances efficiently.
Further Exploring Percentage Calculations: Advanced Concepts
While this article primarily focuses on calculating what percent 13 is of 15, understanding percentages extends to more complex scenarios. Let's briefly touch upon some advanced concepts:
-
Percentage Increase/Decrease: Calculating the percentage change between two values – for example, the percentage increase in sales from one year to the next – involves calculating the difference between the two values, dividing it by the original value, and multiplying by 100.
-
Percentage Points: It's important to distinguish between percentage points and percentage changes. A change from 20% to 25% is a 5 percentage point increase, but a 25% increase (5/20 * 100 = 25%).
-
Compound Interest: Compound interest calculations involve applying interest not only to the principal amount but also to the accumulated interest over time. This is a more complex calculation that requires an understanding of exponential growth.
Conclusion: The Importance of Mastering Percentage Calculations
Mastering percentage calculations is a fundamental skill with wide-ranging applications. From navigating personal finances to interpreting data and making informed decisions in various fields, a strong grasp of percentages empowers you to analyze information effectively and solve practical problems accurately. The seemingly simple question of "What percent is 13 of 15?" serves as a springboard to a deeper understanding of this crucial mathematical concept and its real-world relevance. By understanding the different methods and their applications, you equip yourself with a valuable tool for success in various aspects of life. Remember to practice regularly and apply your knowledge to real-world scenarios to reinforce your understanding and build confidence in your percentage calculation skills.
Latest Posts
Latest Posts
-
59 Centimeters Is How Many Inches
Apr 18, 2025
-
How Many Liters Is 64 Oz Of Water
Apr 18, 2025
-
What Is 30 Out Of 40
Apr 18, 2025
-
What Percent Is 3 Of 7
Apr 18, 2025
-
What Energy Transformation Happens In A Motor
Apr 18, 2025
Related Post
Thank you for visiting our website which covers about What Percent Is 13 Of 15 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.