What Percent Is 25 Of 80
Kalali
Apr 15, 2025 · 5 min read
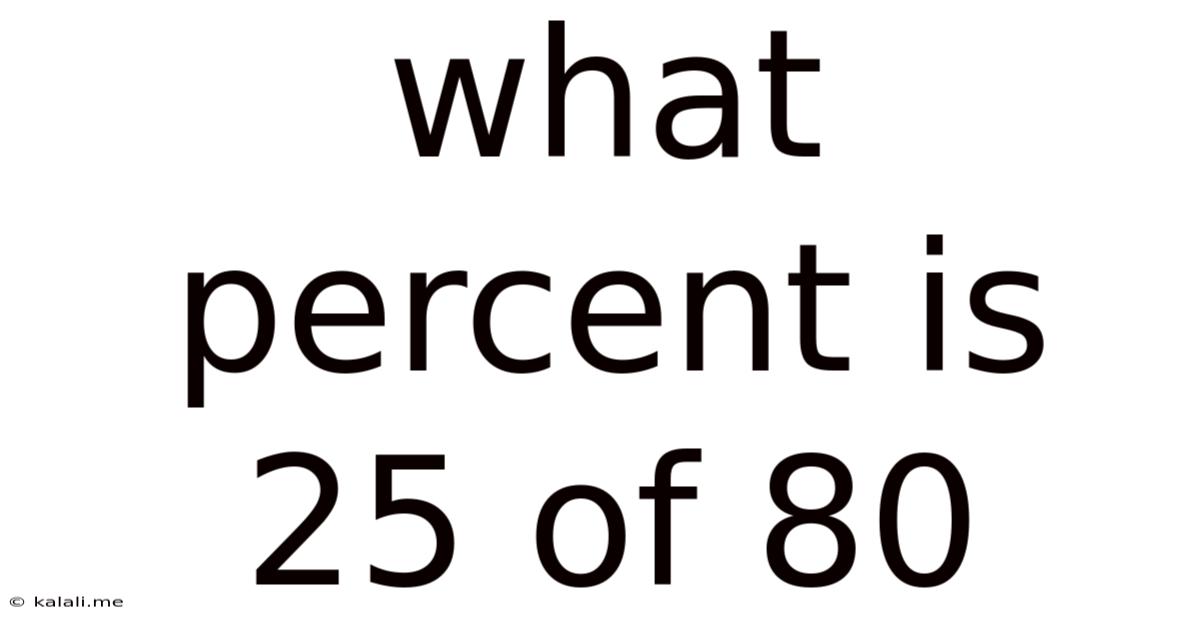
Table of Contents
What Percent is 25 of 80? A Deep Dive into Percentage Calculations and Applications
What percent is 25 of 80? This seemingly simple question opens the door to a world of percentage calculations, their practical applications, and the underlying mathematical principles. Understanding percentages is fundamental to various aspects of life, from financial planning and budgeting to interpreting data and understanding statistics. This article will not only answer the initial question but also explore the broader context of percentage calculations, providing you with a comprehensive understanding of this essential mathematical concept.
Meta Description: This article comprehensively explains how to calculate what percent 25 is of 80, delving into the underlying mathematical principles, providing multiple methods for solving percentage problems, and showcasing real-world applications of percentage calculations.
Understanding Percentages: The Basics
A percentage is a way of expressing a number as a fraction of 100. The word "percent" literally means "per hundred." When we say "x percent," we mean x out of 100, or x/100. This fractional representation is key to understanding percentage calculations. The percentage symbol (%) is simply a shorthand notation for this fraction.
For example, 50% means 50/100, which simplifies to 1/2 or 0.5. This means that 50% represents half of a whole. Similarly, 25% represents one-quarter (25/100 = 1/4 = 0.25), and 75% represents three-quarters (75/100 = 3/4 = 0.75).
Calculating "What Percent is 25 of 80?" - Method 1: The Proportion Method
The most straightforward method for solving "What percent is 25 of 80?" involves setting up a proportion. A proportion is an equation stating that two ratios are equal. We can express the problem as a proportion:
25/80 = x/100
Where 'x' represents the percentage we want to find. To solve for 'x', we can cross-multiply:
25 * 100 = 80 * x
2500 = 80x
Now, we divide both sides by 80 to isolate 'x':
x = 2500 / 80
x = 31.25
Therefore, 25 is 31.25% of 80.
Calculating "What Percent is 25 of 80?" - Method 2: The Decimal Method
Another common approach involves converting the fraction to a decimal and then multiplying by 100. First, we express the relationship as a fraction:
25/80
Next, we convert this fraction to a decimal by dividing 25 by 80:
25 ÷ 80 = 0.3125
Finally, we multiply the decimal by 100 to express it as a percentage:
0.3125 * 100 = 31.25%
This confirms that 25 is 31.25% of 80.
Calculating "What Percent is 25 of 80?" - Method 3: Using Percentage Formula
The basic percentage formula is:
(Part / Whole) * 100 = Percentage
In our problem:
- Part = 25
- Whole = 80
Substituting these values into the formula:
(25 / 80) * 100 = 31.25%
This method reinforces the previous results, demonstrating that 25 is indeed 31.25% of 80.
Real-World Applications of Percentage Calculations
The ability to calculate percentages is invaluable in numerous real-world scenarios. Here are a few examples:
-
Finance: Calculating interest rates, discounts, taxes, tips, profit margins, and investment returns all rely heavily on percentage calculations. For example, determining the interest earned on a savings account or the discount on a sale item requires a solid understanding of percentages.
-
Retail and Sales: Retail businesses use percentages extensively for pricing strategies, sales promotions, and calculating markups. Understanding percentage change allows businesses to track sales performance and adjust pricing accordingly. Calculating discounts (e.g., a 20% discount on a $100 item) is a direct application of percentage calculations.
-
Data Analysis and Statistics: Percentages are crucial for interpreting data and presenting statistical information. For example, expressing survey results as percentages makes the data easier to understand and compare. Analyzing market share, comparing growth rates, and evaluating the effectiveness of marketing campaigns all involve percentage calculations.
-
Science and Engineering: Percentages are used in various scientific and engineering applications, such as expressing concentrations of solutions, calculating efficiency of processes, and representing error margins in measurements. For example, calculating the percentage of a specific element in a compound is a common scientific task.
-
Everyday Life: From calculating the tip at a restaurant to understanding nutritional information on food labels, percentages are ubiquitous in our daily lives. Determining the percentage of fat or protein in a food product requires accurate percentage calculations.
Beyond the Basics: More Complex Percentage Problems
While the problem "What percent is 25 of 80?" is relatively straightforward, percentage calculations can become more complex. These complexities often involve:
-
Finding the whole (or base): Given a percentage and a part, finding the whole amount. For example: "30% of what number is 15?"
-
Finding the part: Given a percentage and the whole, finding the part. For example: "What is 15% of 200?"
-
Percentage increase and decrease: Calculating the percentage change between two values. For example, "If the price of an item increased from $50 to $60, what is the percentage increase?"
-
Compound percentages: Calculating percentages applied sequentially, as often seen with compound interest calculations.
Solving these more complex percentage problems requires a deeper understanding of the underlying principles and the ability to adapt the fundamental formulas. Mastering these variations equips you to tackle a wider range of percentage-related challenges.
Using Technology for Percentage Calculations
While manual calculations are valuable for developing a strong understanding of percentages, technology can significantly simplify the process, particularly for more complex problems. Spreadsheets (like Microsoft Excel or Google Sheets), calculators, and specialized software can all be used to perform percentage calculations quickly and accurately. Many online calculators are also readily available for solving various percentage-related problems.
Conclusion
The answer to "What percent is 25 of 80?" is 31.25%. However, this article extends beyond a simple numerical solution. It delves into the core principles of percentage calculations, demonstrating multiple approaches to solve the problem and highlighting their importance in various aspects of life. Understanding percentages is a critical skill, enabling you to navigate financial matters, interpret data, and engage with the world around you more effectively. By mastering these fundamental concepts and utilizing available tools, you'll be well-equipped to tackle a wide range of percentage-related problems with confidence. The ability to accurately and efficiently calculate percentages is a valuable asset in both personal and professional settings.
Latest Posts
Related Post
Thank you for visiting our website which covers about What Percent Is 25 Of 80 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.