What Percent Is 28 Of 40
Kalali
Apr 18, 2025 · 5 min read
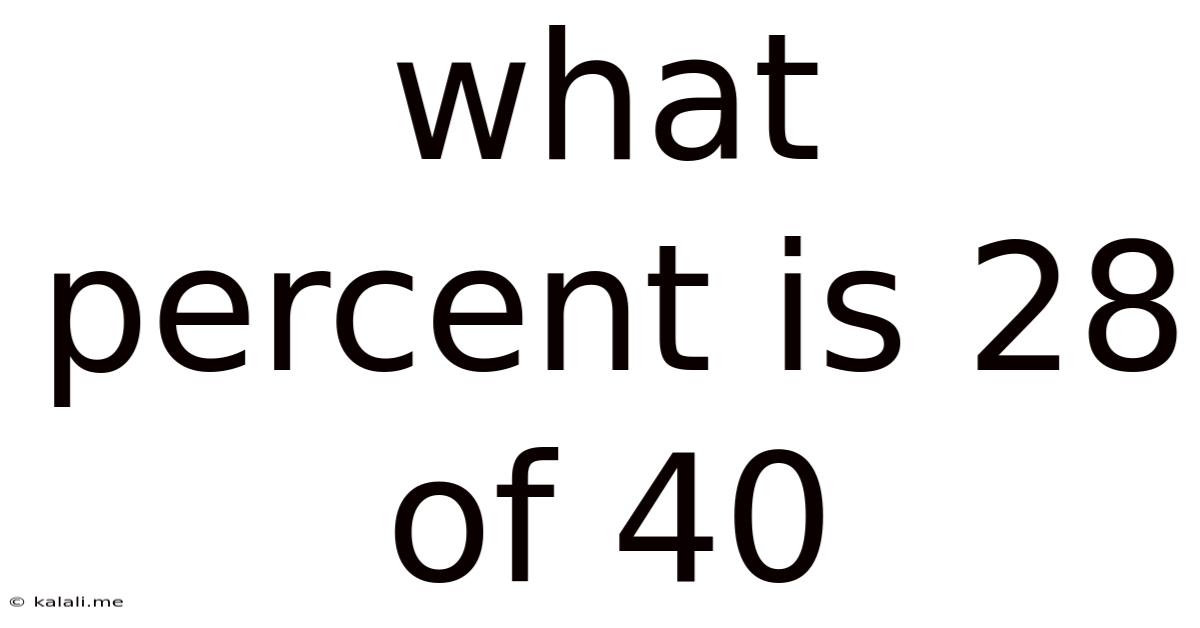
Table of Contents
What Percent is 28 of 40? A Deep Dive into Percentage Calculations and Real-World Applications
What percent is 28 of 40? This seemingly simple question opens the door to a world of percentage calculations, their practical applications, and the underlying mathematical principles. This article will not only answer this specific question but also explore the broader concept of percentages, providing you with the tools and understanding to tackle similar problems with confidence. We'll delve into different methods of calculation, explore various real-world scenarios where percentage calculations are crucial, and even touch upon advanced percentage-related concepts.
Understanding Percentages: The Foundation
Percentages are a fundamental part of mathematics and everyday life. They represent a fraction of 100, offering a standardized way to express proportions and ratios. The word "percent" itself comes from the Latin "per centum," meaning "out of one hundred." This means that 25%, for example, signifies 25 parts out of 100, or 25/100, which simplifies to the fraction 1/4.
Calculating "What Percent is 28 of 40?" – Three Methods
Now, let's tackle the core question: what percent is 28 of 40? We can solve this using three common methods:
Method 1: The Fraction Method
This method directly translates the problem into a fraction and then converts it to a percentage.
-
Express as a Fraction: The problem "what percent is 28 of 40?" can be expressed as the fraction 28/40.
-
Simplify the Fraction (Optional): We can simplify this fraction by dividing both the numerator and denominator by their greatest common divisor, which is 4. This simplifies the fraction to 7/10.
-
Convert to a Decimal: To convert the fraction to a decimal, we divide the numerator (7) by the denominator (10): 7 ÷ 10 = 0.7
-
Convert to a Percentage: To convert the decimal to a percentage, we multiply by 100: 0.7 × 100 = 70%
Therefore, 28 is 70% of 40.
Method 2: The Proportion Method
This method uses proportions to solve for the unknown percentage.
-
Set up a Proportion: We can set up a proportion as follows:
x/100 = 28/40where 'x' represents the unknown percentage. -
Cross-Multiply: Cross-multiplying gives us:
40x = 2800 -
Solve for x: Dividing both sides by 40 gives us:
x = 70
Therefore, 28 is 70% of 40.
Method 3: The Formula Method
This method uses a straightforward formula:
Percentage = (Part / Whole) × 100
-
Identify the Part and the Whole: In this problem, the part is 28, and the whole is 40.
-
Substitute into the Formula: Percentage = (28 / 40) × 100
-
Calculate: Percentage = 0.7 × 100 = 70%
Therefore, 28 is 70% of 40. All three methods yield the same result, confirming that 28 represents 70% of 40.
Real-World Applications of Percentage Calculations
Percentage calculations are ubiquitous in our daily lives. Here are some examples:
-
Sales and Discounts: Retail stores frequently advertise discounts like "30% off." Understanding percentages allows you to quickly calculate the actual price reduction. For instance, a 30% discount on a $100 item would save you $30 (30% of $100).
-
Taxes and Interest: Calculating taxes and interest involves percentages. Income tax rates, sales tax, and interest on loans or savings accounts are all expressed as percentages.
-
Financial Statements: Financial statements like balance sheets and income statements heavily utilize percentages to represent ratios and proportions of different financial aspects of a business. For example, profit margins and debt-to-equity ratios are expressed as percentages.
-
Grade Calculations: In education, your grades are often expressed as percentages. Understanding percentages helps you track your academic progress and understand your performance relative to the total possible score.
-
Statistical Analysis: Percentages are fundamental in statistics to represent proportions within data sets. For instance, survey results are often presented as percentages to show the distribution of responses.
-
Scientific Research: Scientific experiments and research often involve calculating percentages to represent changes, growth rates, or error margins.
-
Health and Nutrition: Nutritional information on food labels uses percentages to indicate the recommended daily intake of various nutrients.
-
Sports Statistics: Sports statistics heavily rely on percentages, such as batting averages in baseball, field goal percentages in basketball, and completion percentages in football.
Advanced Percentage Concepts
Beyond the basic calculations, there are more advanced concepts involving percentages:
-
Percentage Increase and Decrease: These calculations determine the percentage change between two values. For example, if a price increases from $50 to $60, the percentage increase is calculated as: [(60 - 50) / 50] × 100 = 20%. Similarly, percentage decrease is calculated analogously.
-
Compound Interest: This involves calculating interest not only on the principal amount but also on the accumulated interest. It's a key concept in finance and investments.
-
Percentage Points: It's important to differentiate between percentage points and percentages. A change from 10% to 15% is a 5 percentage point increase, but a 50% increase in the percentage itself.
-
Weighted Averages: When calculating averages, weights may be applied to different values, often expressed as percentages, to reflect their relative importance.
Conclusion
Understanding percentages is a crucial skill for navigating everyday life and various professional fields. The simple question, "What percent is 28 of 40?" serves as a springboard to explore the broader applications and complexities of percentage calculations. By mastering these concepts and employing various calculation methods, you'll be well-equipped to tackle numerous percentage-related problems with accuracy and confidence. Remember to always double-check your work and consider the context to ensure the accuracy and meaningfulness of your results. Practice is key to mastering percentage calculations, so don't hesitate to explore different problems and applications to solidify your understanding. The more you practice, the more intuitive percentage calculations will become.
Latest Posts
Latest Posts
-
What Is Equivalent To 2 Quarts
Apr 19, 2025
-
Is Eubacteria Single Celled Or Multicellular
Apr 19, 2025
-
3 5 Feet Is How Many Inches
Apr 19, 2025
-
3 Out Of 13 As A Percentage
Apr 19, 2025
-
Which Statement Describes An Atomic Nucleus
Apr 19, 2025
Related Post
Thank you for visiting our website which covers about What Percent Is 28 Of 40 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.