What Percent Is 3 Of 5
Kalali
Apr 09, 2025 · 5 min read
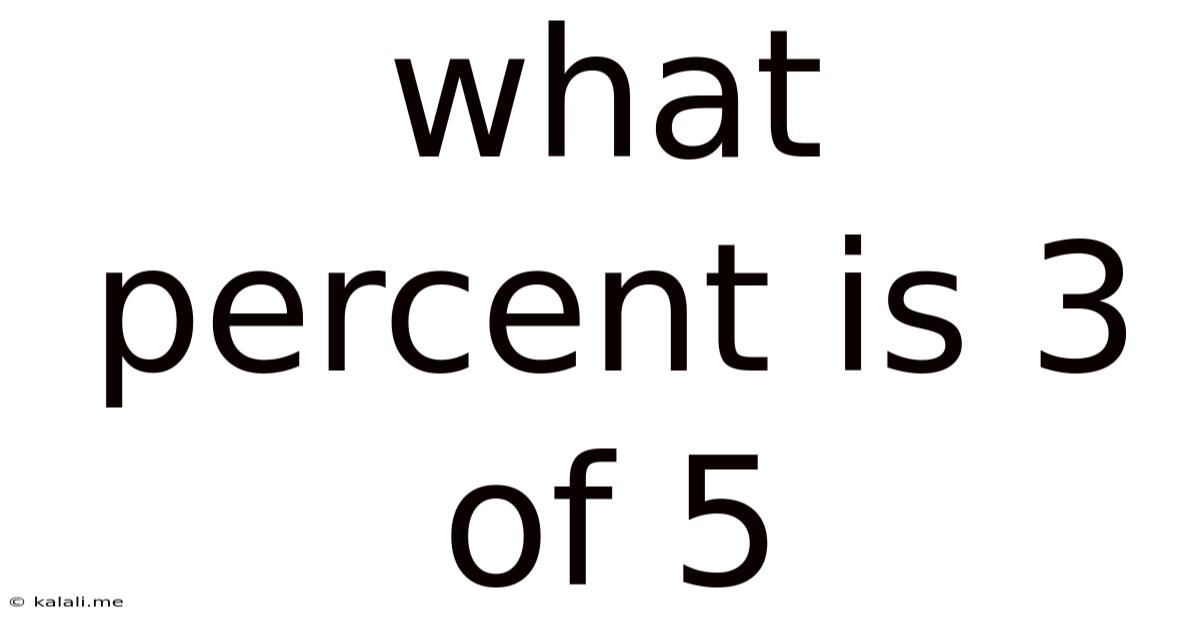
Table of Contents
What Percent is 3 of 5? A Deep Dive into Percentage Calculations and Their Applications
This seemingly simple question, "What percent is 3 of 5?", opens the door to a vast world of percentage calculations and their practical applications in various fields. While the answer itself is straightforward (60%), understanding the underlying principles and exploring diverse methods of calculation is crucial for anyone aiming to master percentages. This article will delve deep into the topic, providing multiple approaches to solving this problem and demonstrating the significance of percentage calculations in everyday life and professional contexts.
Meta Description: Learn how to calculate percentages effectively! This comprehensive guide explains multiple methods for determining what percent 3 is of 5, and explores the practical applications of percentage calculations in various fields.
Understanding the Fundamentals of Percentages
Before jumping into the calculation, let's establish a solid foundation in percentage concepts. A percentage is a fraction or ratio expressed as a number out of 100. The symbol "%" represents "per cent" or "out of 100." Therefore, 60% means 60 out of 100, or 60/100, which simplifies to 3/5.
This core understanding is critical for tackling percentage problems. We can express any fraction or ratio as a percentage using a simple formula, and vice-versa. Mastering this conversion is key to navigating various percentage-related scenarios.
Method 1: The Classic Formula Approach
The most common and straightforward method involves a simple formula:
(Part / Whole) x 100% = Percentage
In our case:
- Part: 3
- Whole: 5
Substituting these values into the formula:
(3 / 5) x 100% = 0.6 x 100% = 60%
Therefore, 3 is 60% of 5. This method is universally applicable and forms the bedrock of all percentage calculations.
Method 2: Using Proportions
Another effective method uses proportions. We can set up a proportion to solve for the unknown percentage:
3/5 = x/100
To solve for x (the percentage), we cross-multiply:
5x = 300
x = 300 / 5
x = 60
Therefore, 3 is 60% of 5. This method is particularly helpful when dealing with more complex percentage problems involving unknown values.
Method 3: Decimal Conversion
This approach converts the fraction to a decimal and then multiplies by 100%.
First, convert the fraction 3/5 to a decimal by dividing 3 by 5:
3 ÷ 5 = 0.6
Then, multiply the decimal by 100% to express it as a percentage:
0.6 x 100% = 60%
This method provides a quick and efficient way to calculate percentages, especially when using a calculator.
Practical Applications of Percentage Calculations
The ability to accurately calculate percentages is not just a mathematical skill; it's a practical life skill with numerous applications across various domains:
1. Finance and Business:
- Calculating interest rates: Understanding interest rates on loans, savings accounts, and investments heavily relies on percentage calculations. Calculating simple and compound interest involves directly applying percentage formulas.
- Profit margins: Businesses use percentages to determine profit margins—the difference between the cost of goods and the selling price, expressed as a percentage of the selling price. This metric is critical for pricing strategies and profitability analysis.
- Sales tax: Calculating sales tax on purchases involves determining a percentage of the pre-tax amount.
- Discounts and markups: Businesses frequently use percentages to advertise discounts and markups on products. Understanding these percentages is essential for consumers to make informed purchasing decisions.
- Financial statements analysis: Financial ratios, such as return on investment (ROI) and debt-to-equity ratio, are often expressed as percentages and are vital for analyzing a company’s financial health.
2. Everyday Life:
- Tip calculations: Calculating tips in restaurants usually involves determining a percentage of the total bill amount.
- Grocery shopping: Comparing prices and unit costs often requires percentage calculations to determine the best value for money.
- Sales and discounts: Consumers frequently use percentages to compare sales and discounts offered by different retailers.
- Understanding statistics: Many statistical data, such as population growth rates, unemployment rates, and inflation rates, are expressed as percentages.
3. Science and Engineering:
- Error analysis: In scientific experiments, percentages are used to express the magnitude of measurement errors or uncertainties.
- Chemical concentrations: The concentration of solutions is often expressed as a percentage by weight or volume.
- Efficiency calculations: In engineering, efficiency is often calculated as a percentage of the input to output ratio.
4. Education:
- Grading systems: Many educational institutions use percentage-based grading systems to assess student performance.
- Test scores: Test scores are often expressed as percentages to indicate the proportion of correct answers.
5. Healthcare:
- Medication dosages: Dosage calculations in healthcare frequently involve percentages to ensure accurate administration of medication.
- Disease prevalence: The prevalence of diseases is usually expressed as a percentage of the total population.
Advanced Percentage Calculations and Problem-Solving
While the "what percent is 3 of 5" problem is basic, understanding its underlying principles allows you to tackle more complex scenarios. Consider these examples:
- Finding the whole: If 20% of a number is 10, what is the number? (Solution: 50)
- Finding the part: What is 35% of 200? (Solution: 70)
- Percentage increase/decrease: A product's price increased from $50 to $60. What is the percentage increase? (Solution: 20%)
- Compound percentages: Calculating compound interest or population growth over multiple periods.
Mastering these advanced techniques requires a strong grasp of the fundamental concepts discussed earlier. Practice is key to improving proficiency in percentage calculations.
Conclusion: Beyond the Basics
The seemingly simple question, "What percent is 3 of 5?", serves as a gateway to understanding a crucial mathematical concept with far-reaching applications. By mastering the various methods of calculating percentages and appreciating their practical relevance, you equip yourself with a valuable skill applicable across numerous facets of life, from personal finance to professional endeavors. Remember that consistent practice and a firm understanding of the underlying principles are essential for confidently tackling diverse percentage-related challenges. The ability to accurately and efficiently calculate percentages is an invaluable asset in today's world.
Latest Posts
Latest Posts
-
7 Is What Percent Of 8
Apr 17, 2025
-
2 1 4 As An Improper Fraction
Apr 17, 2025
-
65 Cm Equals How Many Inches
Apr 17, 2025
-
What Is 100 In Decimal Form
Apr 17, 2025
-
What Is 60 As A Fraction
Apr 17, 2025
Related Post
Thank you for visiting our website which covers about What Percent Is 3 Of 5 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.