What Percent Is 3 Out Of 7
Kalali
Apr 12, 2025 · 5 min read
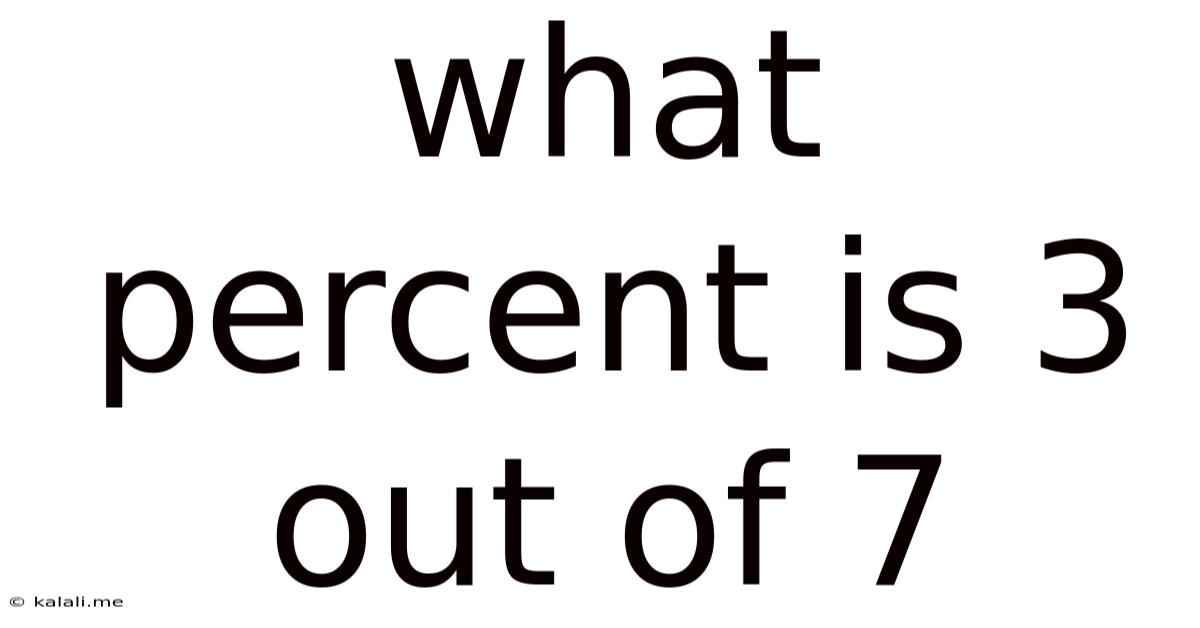
Table of Contents
What Percent is 3 out of 7? A Comprehensive Guide to Percentage Calculations
Finding the percentage that 3 represents out of 7 might seem like a simple arithmetic problem, but understanding the underlying principles is crucial for various applications, from everyday budgeting to complex statistical analysis. This comprehensive guide will not only answer the question "What percent is 3 out of 7?" but also equip you with the tools and understanding to tackle similar percentage calculations with confidence. We’ll delve into various methods, explore practical examples, and uncover the broader context of percentages in different fields.
Meta Description: Learn how to calculate percentages easily! This guide explains how to determine what percent 3 is out of 7, covering various methods and practical examples. Master percentage calculations for everyday use and beyond.
Understanding Percentages: The Foundation
Before diving into the calculation, let's establish a solid understanding of percentages. A percentage is a fraction or ratio expressed as a number out of 100. The term "percent" literally means "per hundred." Therefore, 50% means 50 out of 100, or 50/100, which simplifies to 1/2. Understanding this fundamental concept is key to mastering percentage calculations.
Method 1: The Fraction-to-Decimal-to-Percentage Approach
This method is arguably the most straightforward approach for calculating percentages. It involves three steps:
-
Express the numbers as a fraction: The problem "What percent is 3 out of 7?" translates directly to the fraction 3/7.
-
Convert the fraction to a decimal: To convert a fraction to a decimal, divide the numerator (the top number) by the denominator (the bottom number). In this case, 3 divided by 7 is approximately 0.4286.
-
Convert the decimal to a percentage: Multiply the decimal by 100 and add the percent sign (%). So, 0.4286 multiplied by 100 is 42.86%.
Therefore, 3 out of 7 is approximately 42.86%.
Method 2: Using Proportions
This method employs the concept of proportions, offering a slightly different perspective on the calculation. We set up a proportion where one ratio represents the known values (3 out of 7) and the other represents the unknown percentage (x out of 100).
The proportion is set up as follows:
3/7 = x/100
To solve for x, we cross-multiply:
7x = 300
Then, divide both sides by 7:
x = 300/7 ≈ 42.86
Therefore, x is approximately 42.86, meaning 3 out of 7 is approximately 42.86%. This method reinforces the underlying relationship between fractions and percentages.
Method 3: Using a Calculator
Most calculators have a percentage function that simplifies the process significantly. Simply enter 3 ÷ 7 and then multiply the result by 100. The calculator will directly display the percentage, eliminating the need for manual decimal-to-percentage conversion. This method is particularly useful when dealing with more complex percentage calculations.
Practical Applications: Where Percentage Calculations Matter
Understanding percentage calculations isn't just about solving math problems; it's a vital skill in numerous real-world scenarios. Here are a few examples:
-
Financial Calculations: Calculating interest rates, discounts, tax amounts, profit margins, and investment returns all rely heavily on percentage calculations. For example, if a store offers a 20% discount on an item, you'll need to calculate the discounted price by subtracting 20% of the original price.
-
Statistics and Data Analysis: Percentages are fundamental in presenting and interpreting statistical data. For instance, understanding the percentage of respondents who favor a particular candidate in a survey provides valuable insights into public opinion. Conversion rates (e.g., website visitors converting into customers) are also expressed as percentages.
-
Scientific Research: In scientific studies, percentages are used extensively to express proportions, probabilities, and error margins.
-
Everyday Life: Calculating tips at restaurants, determining sale prices, understanding nutrition labels (percentage of daily values), and even grasping weather forecasts (percentage chance of rain) all involve percentage calculations.
Advanced Percentage Problems: Building Your Skills
While calculating "What percent is 3 out of 7?" is a relatively straightforward problem, let's explore some more complex scenarios to further enhance your understanding:
-
Finding the whole when given a percentage and a part: Imagine you know that 25% of a number is 10. How do you find the original number (the whole)? You can set up a proportion: 25/100 = 10/x. Solving for x reveals the original number.
-
Calculating percentage increase or decrease: Suppose a stock price increases from $50 to $60. What's the percentage increase? First, calculate the difference ($10), then divide the difference by the original price ($50) and multiply by 100. This gives you a 20% increase.
-
Compounding percentages: This involves calculating percentages on top of already-calculated percentages. For example, calculating compound interest involves applying interest rates year after year, with each year's interest being added to the principal before the next calculation.
-
Percentages with multiple parts: Imagine you have a group of 100 people, with 30% being male, 40% female, and the rest being non-binary. You can calculate the percentage of non-binary individuals by subtracting the percentages of males and females from 100%.
Tips for Mastering Percentage Calculations
-
Practice regularly: The more you practice, the more comfortable you'll become with percentage calculations. Start with simple problems and gradually work your way up to more complex scenarios.
-
Use visual aids: Diagrams, charts, and graphs can help you visualize percentage relationships and make calculations easier to understand.
-
Understand the underlying concepts: Don't just memorize formulas; understand the logic behind them. This will make it easier to adapt your approach to different problems.
-
Utilize online resources: Numerous websites and apps provide practice problems, tutorials, and calculators for percentage calculations.
-
Break down complex problems: If you encounter a complicated problem, break it down into smaller, more manageable steps.
Conclusion: Beyond the Basics of Percentage Calculations
This guide provided a thorough exploration of how to calculate what percent 3 is out of 7, utilizing different methods and highlighting the importance of understanding the underlying principles of percentage calculations. Remember that mastering percentages is not merely about solving mathematical problems; it's about developing a crucial skill applicable across various domains. By understanding these concepts and practicing regularly, you'll build a solid foundation for tackling percentage problems with confidence and applying them effectively in your daily life and professional pursuits. From managing finances to interpreting data, the ability to understand and work with percentages is invaluable.
Latest Posts
Latest Posts
-
154 Cm To Inches And Feet
Apr 13, 2025
-
How Many Inches Is 23 5 Cm
Apr 13, 2025
-
21 Is What Percent Of 30
Apr 13, 2025
-
117 Grados En Fahrenheit A Celsius
Apr 13, 2025
-
What Is 9 To The Power Of 2
Apr 13, 2025
Related Post
Thank you for visiting our website which covers about What Percent Is 3 Out Of 7 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.