What Percent Of 12 Is 48
Kalali
Apr 13, 2025 · 5 min read
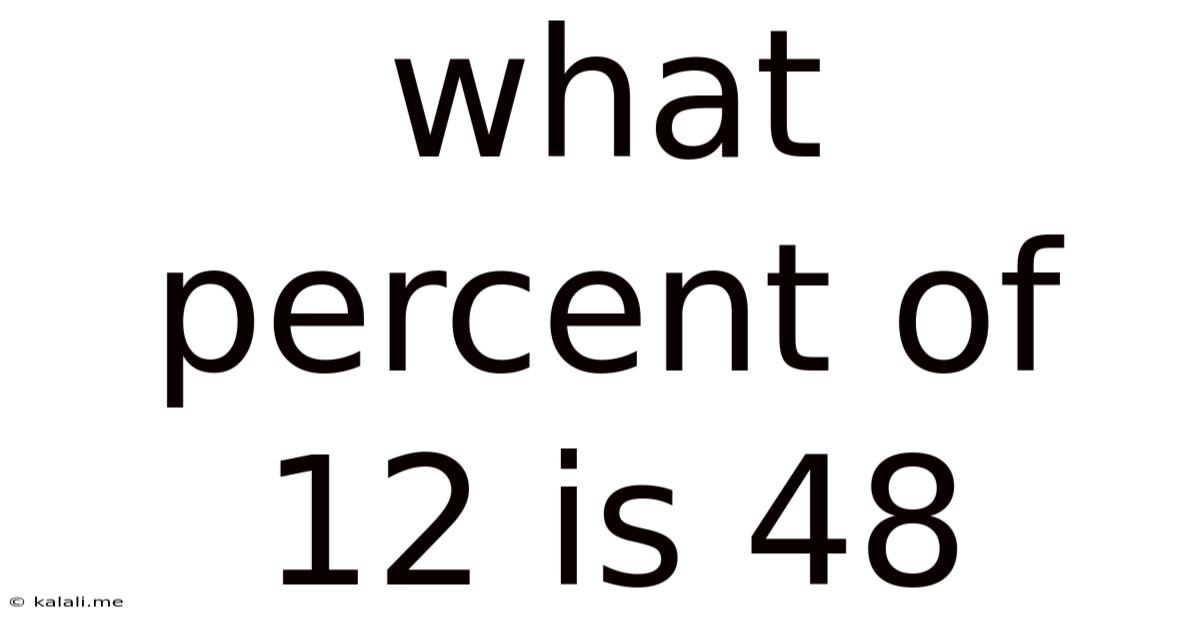
Table of Contents
What Percent of 12 is 48? Unraveling Percentages and Their Applications
This seemingly simple question, "What percent of 12 is 48?", opens a door to a fundamental mathematical concept with wide-ranging applications across various fields. Understanding percentages is crucial for everything from calculating discounts and taxes to analyzing financial data and interpreting scientific research. This article will not only answer the question directly but will also delve into the underlying principles, provide multiple methods for solving percentage problems, and explore real-world examples showcasing the importance of percentage calculations.
Meta Description: Learn how to calculate percentages with a detailed explanation of how to determine what percent of 12 is 48. Explore various methods, real-world applications, and gain a comprehensive understanding of percentage calculations.
Understanding Percentages: A Foundation
A percentage is a way of expressing a number as a fraction of 100. The word "percent" itself comes from the Latin "per centum," meaning "out of a hundred." Therefore, 50% means 50 out of 100, which is equivalent to the fraction 50/100 or the decimal 0.5. Understanding this fundamental definition is key to solving percentage problems.
Solving "What Percent of 12 is 48?"
Several methods can be used to solve this specific problem. Let's explore the most common approaches:
Method 1: Using the Formula
The basic percentage formula is:
(Part / Whole) * 100% = Percentage
In our problem:
- Part: 48 (the value we're comparing)
- Whole: 12 (the base value)
Plugging these values into the formula:
(48 / 12) * 100% = 400%
Therefore, 48 is 400% of 12.
Method 2: Setting up a Proportion
Another effective way to solve percentage problems is by setting up a proportion. We can represent the problem as:
x/100 = 48/12
To solve for x (the percentage), we cross-multiply:
12x = 4800
x = 4800 / 12
x = 400
Thus, the answer is again 400%.
Method 3: Using Decimal Conversion
We can convert the fraction 48/12 to a decimal and then multiply by 100% to find the percentage.
48 / 12 = 4
4 * 100% = 400%
This method provides a straightforward and quick solution.
Why is the Result Greater Than 100%?
It's important to note that percentages can be greater than 100%. This simply means that the "part" is larger than the "whole." In our case, 48 is more than 12, indicating a percentage exceeding 100%. This often arises in situations where growth or increase is being measured.
Real-World Applications of Percentage Calculations
Percentages are ubiquitous in everyday life. Here are a few examples:
-
Retail and Sales: Discounts, markups, and sales tax calculations all rely heavily on percentages. Understanding percentages helps consumers make informed purchasing decisions and businesses manage pricing strategies effectively. For example, a 20% discount on a $100 item means a savings of $20.
-
Finance and Investments: Interest rates, returns on investment (ROI), and financial growth are all expressed as percentages. Investors use percentage calculations to track their portfolio performance and make informed investment decisions. Understanding compound interest, which involves calculating percentages on accumulating amounts, is crucial for long-term financial planning.
-
Science and Statistics: Percentages are used extensively in scientific research to represent proportions, probabilities, and error margins. For example, researchers might report that 75% of participants in a study showed a positive response to a treatment. Statistical significance testing often relies on percentage-based calculations.
-
Data Analysis and Reporting: Percentages are a key tool in presenting data concisely and effectively. They help to simplify complex datasets, making it easier to identify trends, make comparisons, and draw meaningful conclusions. Business reports, market research analyses, and demographic studies frequently utilize percentages to present key findings.
-
Everyday Life: Calculating tips in restaurants, figuring out the percentage of a shared bill, or determining the nutritional content of food products all involve percentage calculations. These everyday applications highlight the importance of understanding and mastering this fundamental mathematical concept.
Advanced Percentage Problems and Concepts
Beyond the basic calculations, the concept of percentages extends to more complex scenarios:
-
Percentage Change: This calculates the relative change between two values. The formula is: [(New Value - Old Value) / Old Value] * 100%. For instance, if a stock price increases from $50 to $60, the percentage change is [(60 - 50) / 50] * 100% = 20%.
-
Compound Interest: This involves calculating interest on the principal amount plus accumulated interest. The formula is: A = P (1 + r/n)^(nt), where A is the future value, P is the principal amount, r is the annual interest rate, n is the number of times the interest is compounded per year, and t is the number of years.
-
Percentage Points: It's crucial to distinguish between percentage change and percentage points. A change from 10% to 20% is a 10 percentage point increase, but a 100% percentage increase.
-
Weighted Averages: When calculating averages from data with different weights, percentages play a vital role. This is common in finance, education (GPA calculation), and other fields.
Mastering Percentages: Tips and Practice
To develop proficiency in percentage calculations, consistent practice is crucial. Here are some tips:
-
Start with the Basics: Ensure you have a solid understanding of the fundamental percentage formula and different solution methods.
-
Practice Regularly: Solve a variety of percentage problems, including those with percentages greater than 100% and those involving percentage change.
-
Use Online Resources: Many websites and apps offer practice problems and tutorials on percentage calculations.
-
Real-World Application: Apply your knowledge to real-life scenarios to reinforce your understanding and improve your problem-solving skills.
Conclusion
The question, "What percent of 12 is 48?", serves as a springboard to explore the versatile world of percentages. This seemingly simple calculation underpins numerous applications across various fields. By mastering percentage calculations, individuals gain a powerful tool for navigating everyday life, making informed financial decisions, and interpreting data effectively. The more you practice, the more comfortable and proficient you will become in using this essential mathematical skill. Remember to always clearly understand the context of the problem and choose the appropriate method for solving it. From simple discounts to complex financial models, the understanding and application of percentages remain consistently relevant and valuable.
Latest Posts
Latest Posts
-
20oz Of Water Is How Many Cups
Apr 14, 2025
-
17 Out Of 25 As A Percent
Apr 14, 2025
-
Convert 36 Degrees Celcius To Farenheit
Apr 14, 2025
-
How Much Is 32 Inches In Feet
Apr 14, 2025
-
What Is The Fraction For 3 75
Apr 14, 2025
Related Post
Thank you for visiting our website which covers about What Percent Of 12 Is 48 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.