What Percent Of 140 Is 112
Kalali
Apr 17, 2025 · 4 min read
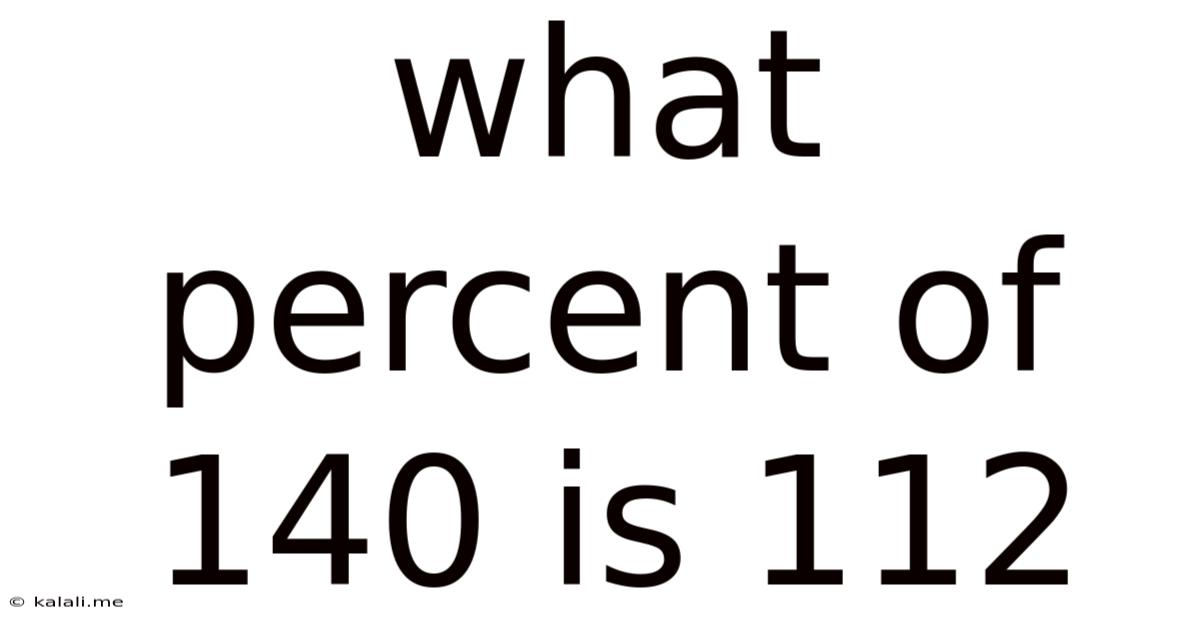
Table of Contents
What Percent of 140 is 112? A Deep Dive into Percentage Calculations
Meta Description: Learn how to calculate what percent 112 is of 140. This comprehensive guide covers the basic formula, step-by-step calculations, real-world applications, and advanced percentage techniques. Master percentage calculations and improve your math skills.
Finding out what percentage one number represents of another is a fundamental skill with widespread applications in various fields, from everyday budgeting to complex financial analysis. This article will delve into the question: "What percent of 140 is 112?" We'll explore the solution methodically, discuss the underlying principles, and explore various related percentage calculations.
Understanding the Basic Percentage Formula
Before diving into the specific problem, let's solidify the fundamental concept of percentages. A percentage is simply a fraction expressed as a number out of 100. The formula for calculating a percentage is:
(Part / Whole) * 100% = Percentage
Where:
- Part: Represents the portion you're interested in (in our case, 112).
- Whole: Represents the total amount (in our case, 140).
- Percentage: The result, expressed as a percentage.
Calculating: What Percent of 140 is 112?
Now, let's apply this formula to our specific problem:
-
Identify the Part and the Whole:
- Part = 112
- Whole = 140
-
Apply the Formula: (112 / 140) * 100% = Percentage
-
Calculate the Fraction: 112 / 140 = 0.8
-
Multiply by 100%: 0.8 * 100% = 80%
Therefore, 112 is 80% of 140.
Different Approaches to Percentage Calculation
While the above method is straightforward, there are alternative approaches that might be more intuitive or efficient depending on the context. Let's explore a few:
Method 2: Using Proportions
Percentages can also be solved using proportions. We can set up a proportion as follows:
x/100 = 112/140
To solve for x (the percentage), we cross-multiply:
140x = 11200
x = 11200 / 140
x = 80
Therefore, x = 80%, confirming our previous result. This method is particularly helpful when dealing with more complex percentage problems.
Method 3: Simplifying the Fraction
Before multiplying by 100%, we can simplify the fraction 112/140. Both numbers are divisible by 4, resulting in 28/35. Further simplification by dividing by 7 gives us 4/5. Converting 4/5 to a percentage:
(4/5) * 100% = 0.8 * 100% = 80%
Real-World Applications of Percentage Calculations
Understanding percentage calculations is crucial in numerous real-world scenarios. Here are a few examples:
- Discounts and Sales: Retail stores frequently advertise discounts as percentages. For example, a "20% off" sale means you pay 80% of the original price.
- Taxes and Fees: Calculating sales tax, income tax, or service charges often involves percentages.
- Financial Calculations: Interest rates on loans and investments, profit margins, and return on investment (ROI) are all expressed as percentages.
- Data Analysis: Percentages are frequently used to represent proportions in data sets, such as market share, survey results, or population statistics. For example, understanding that 80% of respondents prefer a certain product provides valuable market insight.
- Grade Calculations: Many academic grading systems use percentages to represent student performance.
- Tip Calculations: Calculating a gratuity (tip) at a restaurant is a common application of percentage calculation.
Advanced Percentage Problems and Techniques
While our initial problem was relatively simple, percentage calculations can become more complex. Let's explore some advanced scenarios:
- Finding the Whole when the Percentage and Part are Known: Suppose you know that 80% of a number is 112. To find the whole number, we can set up the equation:
0.80 * x = 112
Solving for x:
x = 112 / 0.80 = 140
- Finding the Part when the Percentage and Whole are Known: If you know that you need to find 25% of 140, you would calculate:
0.25 * 140 = 35
- Percentage Increase and Decrease: Calculating percentage change is common. For example, if a value increases from 100 to 120, the percentage increase is:
((120 - 100) / 100) * 100% = 20%
Similarly, if a value decreases from 100 to 80, the percentage decrease is:
((100 - 80) / 100) * 100% = 20%
- Compound Percentages: Compound percentages involve applying a percentage multiple times. For instance, if you have 10% annual growth for three years, you wouldn't simply multiply by 30%. You'd need to calculate the growth year by year, compounding the effect.
Tips for Mastering Percentage Calculations
- Practice Regularly: Consistent practice is key to mastering any mathematical concept, including percentages.
- Use Different Methods: Experiment with various calculation approaches (formula, proportions, simplification) to find the method that suits you best.
- Break Down Complex Problems: For intricate problems, break them down into smaller, manageable steps.
- Utilize Online Calculators (for verification): While it's essential to understand the underlying principles, online calculators can be helpful for checking your answers, particularly with more complex calculations. However, always focus on understanding the method.
- Focus on understanding the concepts, not just memorizing formulas: A thorough understanding of the concepts will allow you to solve a wider variety of problems.
Conclusion
The ability to calculate percentages accurately is a valuable skill applicable across many aspects of life. We've explored the fundamental formula, alternative calculation methods, real-world applications, and more advanced percentage problems. By practicing regularly and understanding the underlying concepts, you can confidently tackle a wide range of percentage calculations and significantly improve your mathematical proficiency. Remember, the key is not just getting the answer but also understanding why the answer is correct. This will make you a more effective problem-solver in any situation.
Latest Posts
Latest Posts
-
18 5 As A Mixed Number
Apr 19, 2025
-
What Is The Prefix Of In
Apr 19, 2025
-
The Ability Of A Substance To Catch Fire Or Burn
Apr 19, 2025
-
35 8 As A Mixed Number
Apr 19, 2025
-
What Is The Domain Of The Function Graphed Below
Apr 19, 2025
Related Post
Thank you for visiting our website which covers about What Percent Of 140 Is 112 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.