What Percent Of 20 Is 14
Kalali
Apr 05, 2025 · 4 min read
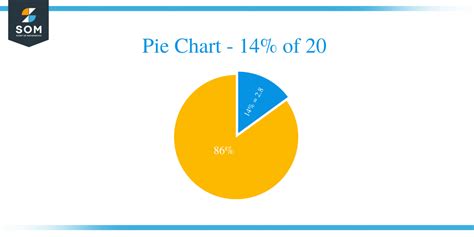
Table of Contents
What Percent of 20 is 14? A Deep Dive into Percentage Calculations
Determining what percent of 20 is 14 involves understanding the fundamental principles of percentages and applying a simple formula. While the answer itself is straightforward, exploring the underlying concepts provides a valuable foundation for tackling more complex percentage problems. This article will not only solve the problem but also delve into the various methods for calculating percentages, exploring different scenarios, and offering practical applications.
Understanding Percentages
A percentage is a fraction or ratio expressed as a portion of 100. The term "percent" literally means "out of 100," derived from the Latin "per centum." Therefore, 14% (fourteen percent) signifies 14 out of every 100. This representation allows for easy comparison and understanding of proportions across different values.
Method 1: The Basic Percentage Formula
The most common approach to solving "what percent of 20 is 14?" is using the fundamental percentage formula:
(Part / Whole) x 100 = Percentage
In this case:
- Part: 14 (the value we're interested in finding the percentage of)
- Whole: 20 (the total value)
Substituting these values into the formula:
(14 / 20) x 100 = 70%
Therefore, 14 is 70% of 20.
Method 2: Setting up a Proportion
Another effective method utilizes proportions. We can set up a proportion to solve for the unknown percentage (let's represent it as 'x'):
14/20 = x/100
To solve for 'x', we cross-multiply:
20x = 1400
x = 1400 / 20
x = 70
Again, this confirms that 14 is 70% of 20.
Method 3: Using Decimal Equivalents
Percentages can be easily converted to decimal equivalents by dividing the percentage by 100. For example, 70% is equivalent to 0.70. We can use this understanding to solve the problem:
0.70 x 20 = 14
This confirms that 70% of 20 is indeed 14.
Practical Applications and Real-World Examples
Understanding percentage calculations is crucial in numerous real-world situations. Here are some examples:
-
Discounts: A store offers a 30% discount on an item priced at $20. To calculate the discount, you'd find 30% of $20, which is ($20 x 0.30 = $6). The discounted price would be $20 - $6 = $14.
-
Sales Tax: If the sales tax in your area is 7%, and you buy an item for $20, the sales tax amount is $20 x 0.07 = $1.40. The total cost would be $20 + $1.40 = $21.40.
-
Grade Calculations: If you answered 14 out of 20 questions correctly on a test, your percentage score would be 70%.
-
Financial Calculations: Percentage calculations are fundamental in finance, including interest rates, loan repayments, investment returns, and profit margins.
-
Data Analysis: Percentages are extensively used in data analysis to represent proportions, trends, and comparisons.
Advanced Percentage Problems and Solving Techniques
While the example of finding what percent of 20 is 14 is relatively straightforward, more complex percentage problems can arise. Here are some examples and techniques for solving them:
-
Finding the Whole: If you know a percentage and the part, you can find the whole. For instance, if 70% of a number is 14, you could set up the equation: 0.7x = 14. Solving for x (by dividing both sides by 0.7) would give you the whole number, which is 20.
-
Finding the Part: If you know the percentage and the whole, you can find the part. For example, if you need to find 30% of 50, you would calculate 0.30 x 50 = 15.
-
Percentage Increase/Decrease: These calculations involve finding the percentage change between two values. The formula is: [(New Value - Old Value) / Old Value] x 100. For example, if a value increases from 20 to 26, the percentage increase is [(26 - 20) / 20] x 100 = 30%.
-
Compound Interest: Compound interest calculations involve applying interest to both the principal amount and accumulated interest. These calculations require more complex formulas.
Tips and Tricks for Efficient Percentage Calculations
-
Use a Calculator: For more complex calculations, using a calculator is highly recommended for accuracy and speed.
-
Convert to Decimals: Converting percentages to decimals simplifies many calculations.
-
Master the Basic Formula: Understanding the core formula (Part / Whole) x 100 is essential.
-
Practice Regularly: The more you practice, the more comfortable and efficient you'll become.
-
Break Down Complex Problems: Large problems can be broken into smaller, more manageable steps.
Conclusion: Mastering Percentages for Everyday Life
Understanding percentages is a vital skill applicable to numerous aspects of everyday life, from managing finances to interpreting data. While seemingly simple, mastering percentage calculations provides a solid foundation for tackling more advanced mathematical concepts and making informed decisions in various contexts. The seemingly basic question, "What percent of 20 is 14?", serves as a perfect entry point to explore the vast world of percentage calculations and their practical applications. By understanding the different methods and techniques discussed in this article, you'll be well-equipped to confidently handle various percentage-related challenges and improve your quantitative reasoning skills. Remember to practice regularly to reinforce your understanding and enhance your proficiency in this essential area of mathematics.
Latest Posts
Latest Posts
-
Anything That Has Mass And Volume
Apr 05, 2025
-
2 Is What Percent Of 15
Apr 05, 2025
-
What Is 92 Degrees Fahrenheit In Celsius
Apr 05, 2025
-
Cual Es El Valor Absoluto De 3
Apr 05, 2025
-
24 Of 60 Is What Percent
Apr 05, 2025
Related Post
Thank you for visiting our website which covers about What Percent Of 20 Is 14 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
