What Percent Of 20 Is 3
Kalali
Mar 27, 2025 · 5 min read
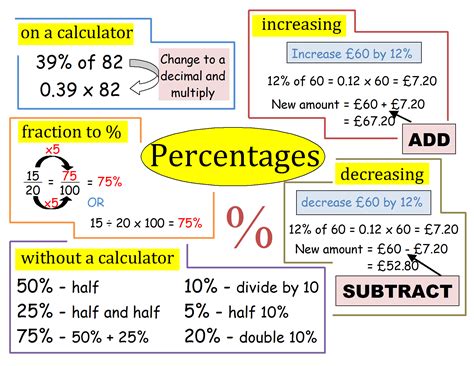
Table of Contents
What Percent of 20 is 3? A Comprehensive Guide to Percentage Calculations
This seemingly simple question, "What percent of 20 is 3?", opens the door to a broader understanding of percentages, a fundamental concept in mathematics with wide-ranging applications in everyday life and various professional fields. This article will not only answer this specific question but also delve into the methods of calculating percentages, exploring different approaches and providing practical examples to solidify your understanding. We’ll also examine the importance of percentage calculations in various contexts, from simple budgeting to complex financial analysis.
Understanding Percentages: The Foundation
Before we tackle the core question, let's solidify our understanding of percentages. A percentage is simply a fraction or ratio expressed as a number out of 100. The term "percent" literally means "out of one hundred" – "per cent" being a contraction of "per centum," Latin for "out of one hundred." Therefore, 50% signifies 50 out of 100, which is equivalent to ½ or 0.5 in decimal form.
Understanding this basic definition is key to tackling any percentage calculation problem. We can represent a percentage as a fraction, decimal, or simply as a percentage symbol (%). The ability to convert between these forms is crucial for solving percentage problems efficiently.
Calculating the Percentage: Three Methods
There are several ways to calculate what percent of 20 is 3. Let’s explore three common methods:
Method 1: The Proportion Method
This method utilizes the concept of proportions. We can set up a proportion to solve for the unknown percentage (x):
x/100 = 3/20
To solve for x, we cross-multiply:
20x = 300
Then, divide both sides by 20:
x = 15
Therefore, 3 is 15% of 20.
Method 2: The Decimal Method
This method involves converting the fraction to a decimal and then multiplying by 100 to express it as a percentage.
First, represent the problem as a fraction:
3/20
Now, convert this fraction to a decimal by dividing the numerator (3) by the denominator (20):
3 ÷ 20 = 0.15
Finally, multiply the decimal by 100 to express it as a percentage:
0.15 x 100 = 15%
Therefore, 3 is 15% of 20.
Method 3: Using the Percentage Formula
The general formula for calculating percentages is:
(Part / Whole) x 100 = Percentage
In our case:
- Part = 3
- Whole = 20
Substituting these values into the formula:
(3 / 20) x 100 = 15%
Again, we arrive at the conclusion that 3 is 15% of 20.
Practical Applications of Percentage Calculations
The ability to calculate percentages is essential in numerous real-world scenarios. Here are a few examples:
1. Financial Calculations:
- Interest Rates: Understanding interest rates, whether on loans, savings accounts, or investments, heavily relies on percentage calculations. For instance, calculating the interest earned on a savings account or the total cost of a loan with interest involves percentage calculations.
- Discounts and Sales Tax: Determining the final price of an item after a discount or adding sales tax involves calculating percentages. For example, a 20% discount on a $100 item reduces the price by $20.
- Investment Returns: Tracking the performance of investments requires calculating percentage returns or losses. For instance, if you invested $1000 and it grew to $1200, your return is a 20% increase.
- Budgeting and Expense Tracking: Managing personal finances effectively often necessitates calculating percentages. For instance, determining the percentage of your income allocated to different expense categories helps track spending habits.
2. Scientific and Statistical Analysis:
- Data Representation: Percentages are frequently used to represent data in charts, graphs, and reports. For instance, analyzing survey results or showing the proportion of different categories in a dataset often uses percentages.
- Probability and Statistics: Probability calculations heavily rely on percentages to express the likelihood of events occurring.
- Scientific Experiments: Expressing the results of scientific experiments, such as the percentage of successful trials or the error rate, uses percentages.
3. Everyday Life Scenarios:
- Tipping in Restaurants: Calculating tips in restaurants usually involves determining a percentage of the total bill.
- Grading Systems: Many educational institutions use percentage-based grading systems. For example, a score of 85 out of 100 is an 85% grade.
- Sales and Commissions: Salespeople often earn commissions based on a percentage of their sales.
- Ingredient Ratios in Recipes: Recipes may specify ingredients as percentages of the total mixture. For instance, a bread recipe might call for 70% flour and 30% water by weight.
Beyond the Basics: Advanced Percentage Calculations
While the "what percent of 20 is 3" question is straightforward, percentage calculations can become more complex. Here are some examples:
- Calculating Percentage Increase or Decrease: Determining the percentage change between two values requires understanding how to calculate the difference, divide it by the original value, and then multiply by 100.
- Calculating Percentage Points: This concept differentiates between a percentage change and a change in percentage points. For instance, a change from 10% to 20% is a 10 percentage point increase, but a 100% increase in percentage terms.
- Compound Interest Calculations: Calculating compound interest involves repeated percentage calculations over time, taking into account the accumulating interest.
- Solving for the Whole or the Part: In some problems, you might need to calculate either the whole amount or a part, given the percentage and either the part or whole.
Mastering Percentage Calculations: Tips and Tricks
Here are some strategies to improve your proficiency in percentage calculations:
- Practice Regularly: Consistent practice is key to mastering any mathematical concept. Solve various problems involving percentages to build your confidence and accuracy.
- Use Different Methods: Experiment with the proportion method, decimal method, and percentage formula to find the approach that suits you best.
- Convert to Fractions and Decimals: Being comfortable converting between percentages, fractions, and decimals helps simplify the calculations.
- Check Your Work: Always double-check your calculations to ensure accuracy. Using a calculator can help minimize errors.
- Understand the Context: Pay attention to the wording of the problem to accurately identify the part and the whole.
Conclusion: The Power of Percentages
Understanding percentages is a fundamental skill with far-reaching applications. From managing personal finances to interpreting statistical data, the ability to calculate and interpret percentages is crucial in both academic and professional settings. This article provided a thorough explanation of how to determine "what percent of 20 is 3," showcasing different calculation methods and highlighting the widespread importance of this fundamental mathematical concept. By mastering percentage calculations, you equip yourself with a powerful tool for navigating various aspects of life. Remember, consistent practice and a solid understanding of the underlying principles are the keys to proficiency.
Latest Posts
Latest Posts
-
Cuanto Es 117 Grados Fahrenheit En Centigrados
Mar 30, 2025
-
V2 Co3 3 Cation And Anion
Mar 30, 2025
-
Boiling Point Of Milk In Celsius
Mar 30, 2025
-
What Is 65 Inches In Height
Mar 30, 2025
-
How Much Is 45cm In Inches
Mar 30, 2025
Related Post
Thank you for visiting our website which covers about What Percent Of 20 Is 3 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
