What Percent Of 200 Is 120
Kalali
Apr 26, 2025 · 5 min read
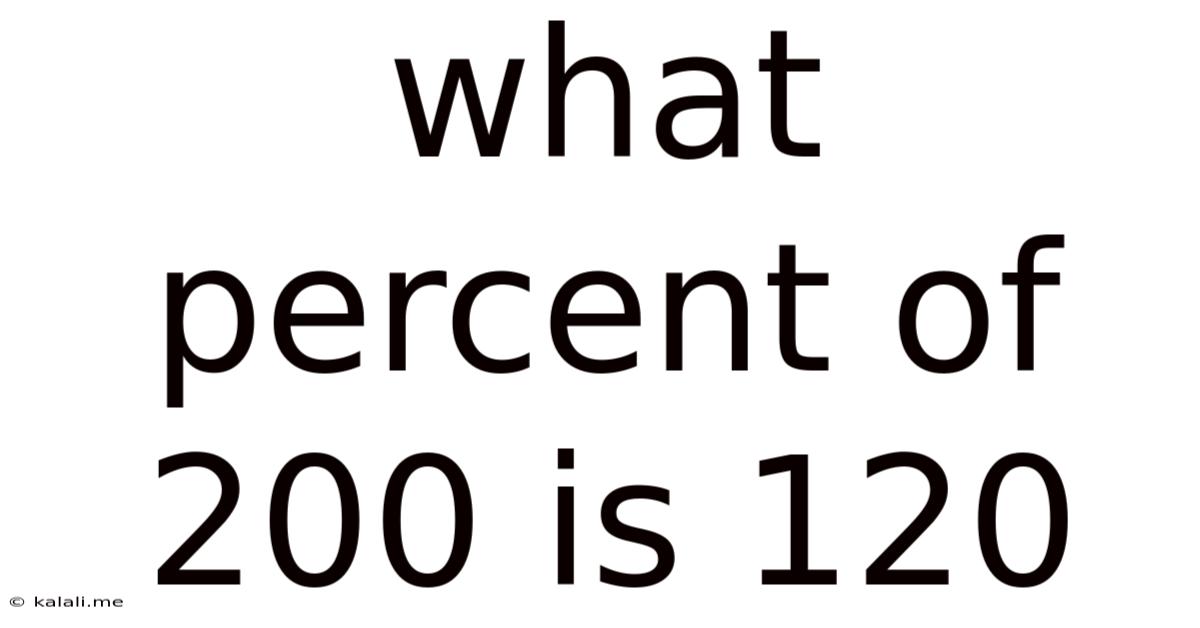
Table of Contents
What Percent of 200 is 120? A Deep Dive into Percentage Calculations
This seemingly simple question – "What percent of 200 is 120?" – opens the door to a fascinating exploration of percentage calculations, their applications in everyday life, and how to approach similar problems with confidence. This article will not only answer the question directly but will also equip you with the knowledge and tools to tackle any percentage problem you might encounter. Understanding percentages is crucial in various fields, from finance and business to science and everyday budgeting. This comprehensive guide will delve into the core concepts, providing multiple methods of solving the problem and expanding on their broader implications.
Understanding Percentages: The Foundation
A percentage is a fraction expressed as a number out of 100. The symbol "%" represents "per cent," meaning "out of one hundred." Percentages are a way of expressing proportions or ratios relative to a whole. For instance, 50% represents 50 out of 100, which is equivalent to the fraction ½ or the decimal 0.5. Understanding this fundamental relationship is key to solving percentage problems.
Method 1: Using the Formula
The most straightforward method to determine what percent of 200 is 120 involves a simple formula:
(Part / Whole) * 100% = Percentage
In this case:
- Part: 120 (the portion we're interested in)
- Whole: 200 (the total amount)
Substituting these values into the formula:
(120 / 200) * 100% = 60%
Therefore, 120 is 60% of 200.
Method 2: Setting up a Proportion
Proportions offer another effective way to solve percentage problems. A proportion is an equation stating that two ratios are equal. We can set up a proportion to represent the problem as follows:
120 / 200 = x / 100
Here, 'x' represents the unknown percentage we're trying to find. To solve for 'x', we can cross-multiply:
200x = 120 * 100 200x = 12000 x = 12000 / 200 x = 60
Again, this confirms that 120 is 60% of 200.
Method 3: Using Decimal Equivalents
Percentages can also be expressed as decimals. To convert a percentage to a decimal, divide the percentage by 100. For example, 60% is equivalent to 0.60. Conversely, to convert a decimal to a percentage, multiply the decimal by 100.
We can use this knowledge to solve our problem. We know that we want to find what percentage of 200 equals 120. We can set up the equation:
0.60 * 200 = 120
This confirms that 0.60, or 60%, of 200 is 120. This method is particularly useful when working with calculators or spreadsheets.
Real-World Applications of Percentage Calculations
Understanding percentage calculations is not just an academic exercise; it's a practical skill applicable in countless situations. Here are a few examples:
-
Finance: Calculating interest rates, discounts, taxes, profit margins, and investment returns all rely heavily on percentage calculations. For instance, understanding compound interest, crucial for investment growth, involves repeated percentage calculations over time.
-
Business: Analyzing sales figures, market share, growth rates, and cost reductions often necessitates working with percentages. Companies frequently use percentage change calculations to track performance over time.
-
Science: Percentages are widely used in scientific research to express data, such as the percentage of a population exhibiting a certain characteristic or the percentage change in a measured variable during an experiment. This is crucial in data interpretation and statistical analysis.
-
Everyday Life: Calculating tips in restaurants, understanding sale discounts at stores, determining the percentage of a budget allocated to specific categories, and interpreting statistics in news reports all involve using percentage calculations.
Advanced Percentage Problems: Expanding Your Skills
While the problem "What percent of 200 is 120?" is relatively straightforward, the underlying principles can be applied to more complex scenarios. Here are a few examples of more advanced problems:
-
Finding the Whole: If 25% of a number is 50, what is the number? This involves reversing the formula, solving for the "whole" instead of the "percentage."
-
Finding the Part: What is 35% of 800? This involves directly applying the formula, calculating the "part" given the "whole" and the "percentage."
-
Percentage Change: A product's price increased from $100 to $125. What is the percentage increase? This requires calculating the difference, then expressing it as a percentage of the original value.
-
Percentage Decrease: A shop offers a 20% discount on an item originally priced at $50. What is the discounted price? This involves calculating the discount amount and subtracting it from the original price.
Troubleshooting and Common Mistakes
Even experienced mathematicians can occasionally make mistakes when working with percentages. Here are some common pitfalls to watch out for:
-
Incorrect Formula Application: Ensure you're using the correct formula and substituting the values in the right places. Double-checking your work is always advisable.
-
Decimal Errors: Be careful when working with decimals, particularly when converting between percentages and decimals. Rounding errors can lead to inaccuracies in the final answer.
-
Mixing Up the Parts and the Whole: Clearly identify the "part" and the "whole" in the problem to avoid confusion and incorrect calculations.
-
Misunderstanding Percentage Increase/Decrease: Understand the difference between calculating a percentage increase and a percentage decrease.
Conclusion: Mastering Percentage Calculations
Mastering percentage calculations is a valuable skill with wide-ranging applications. By understanding the fundamental concepts, utilizing different solution methods, and being aware of potential pitfalls, you can confidently tackle a variety of percentage problems, from simple calculations to more complex scenarios. The seemingly basic question "What percent of 200 is 120?" serves as a springboard to exploring this fundamental mathematical concept and its pervasive role in our daily lives. Remember, practice makes perfect; the more you work with percentages, the more comfortable and proficient you'll become.
Latest Posts
Latest Posts
-
What Is 99 5 Degrees Fahrenheit In Celsius
Apr 26, 2025
-
What Percent Of 12 Is 18
Apr 26, 2025
-
How Many Liters Is 50 Milliliters
Apr 26, 2025
-
How Many Feet In 82 Inches
Apr 26, 2025
-
How Many Oz In A Pund
Apr 26, 2025
Related Post
Thank you for visiting our website which covers about What Percent Of 200 Is 120 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.