What Percent Of 25 Will Make 15
Kalali
Apr 27, 2025 · 5 min read
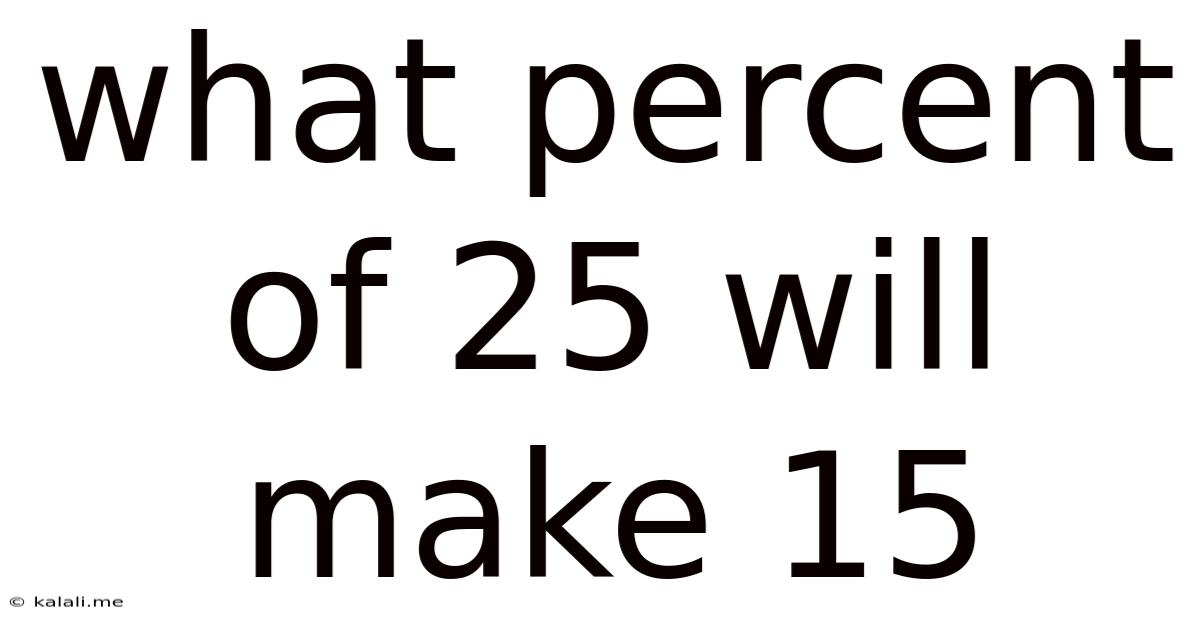
Table of Contents
What Percent of 25 Will Make 15? A Deep Dive into Percentages and Proportions
This seemingly simple question – "What percent of 25 will make 15?" – opens a door to a fascinating exploration of percentages, proportions, and their practical applications in everyday life. Understanding how to solve this type of problem is crucial for various fields, from finance and budgeting to sales and data analysis. This article will not only provide the solution but also delve into the underlying mathematical principles, offering multiple approaches and highlighting the importance of percentage calculations in the real world.
Meta Description: Discover how to calculate what percentage of 25 equals 15. This comprehensive guide explores different methods, explains the underlying mathematical principles, and provides real-world applications of percentage calculations. Learn to confidently solve percentage problems!
Understanding the Problem: Parts and Wholes
The core of the problem lies in understanding the relationship between a part (15) and a whole (25). We're looking for the percentage that represents the proportion of 15 out of 25. In essence, we want to find out what fraction of 25 is represented by 15, and then express that fraction as a percentage.
Method 1: Using the Percentage Formula
The most straightforward approach involves using the basic percentage formula:
(Part / Whole) x 100% = Percentage
In this case:
- Part = 15
- Whole = 25
Substituting these values into the formula:
(15 / 25) x 100% = 60%
Therefore, 60% of 25 is 15.
Method 2: Setting up a Proportion
Another effective method involves setting up a proportion. A proportion is an equation stating that two ratios are equal. We can set up the proportion as follows:
x / 100 = 15 / 25
Where 'x' represents the percentage we're trying to find. To solve for 'x', we can cross-multiply:
25x = 1500
Then, divide both sides by 25:
x = 60
Again, we find that 60% of 25 is 15.
Method 3: Using Decimal Equivalents
Percentages can also be expressed as decimals. To find the decimal equivalent of a percentage, simply divide the percentage by 100. For instance, 60% is equal to 0.60.
We can use this approach to solve the problem:
0.60 x 25 = 15
This confirms that 60% of 25 equals 15.
Practical Applications of Percentage Calculations
The ability to calculate percentages accurately is invaluable in numerous real-world situations. Here are just a few examples:
- Finance: Calculating interest rates, discounts, taxes, profit margins, and investment returns all rely heavily on percentage calculations. For example, understanding what percentage of your income you are saving is crucial for financial planning.
- Sales and Marketing: Determining sales growth, conversion rates, customer acquisition costs, and market share all involve percentage calculations. Analyzing these percentages can help businesses make informed decisions about their strategies.
- Data Analysis: Percentages are fundamental to interpreting data. They allow us to express proportions, compare different data sets, and identify trends. For instance, in market research, percentages are used to represent the share of the market held by different brands.
- Education: Calculating grades, assessing student performance, and understanding test results frequently involves percentage calculations. This helps teachers track student progress and tailor their instruction accordingly.
- Everyday Life: Calculating tips, discounts at stores, or even figuring out the percentage of a recipe to adjust portion sizes all require basic percentage knowledge. For example, understanding the percentage of protein, fat and carbohydrates in your diet helps you plan meals effectively.
Beyond the Basics: More Complex Percentage Problems
While the problem of finding what percent of 25 makes 15 is relatively straightforward, the principles involved can be applied to more complex scenarios. For example:
- Finding the percentage increase or decrease: Suppose a product's price increased from $25 to $30. To find the percentage increase, you would first find the difference ($5), then divide that difference by the original price ($25), and finally multiply by 100% to get the percentage increase (20%).
- Calculating compound interest: Compound interest involves calculating interest on both the principal amount and accumulated interest. This requires repeated percentage calculations over time.
- Determining percentage points: It's crucial to differentiate between a percentage change and a percentage point change. For instance, if interest rates rise from 5% to 10%, it's a 5 percentage point increase, but a 100% increase in the interest rate itself.
Mastering Percentage Calculations: Tips and Tricks
To become proficient in percentage calculations, consider these tips:
- Practice Regularly: The more you practice, the more comfortable and confident you'll become with solving percentage problems. Start with simple problems and gradually move towards more complex ones.
- Understand the Fundamentals: Ensure you have a solid grasp of the basic concepts of percentages, ratios, and proportions.
- Use Different Methods: Try different approaches to solving percentage problems, such as using the formula, setting up a proportion, or using decimal equivalents. This will help you understand the underlying principles better and choose the most efficient method for a given problem.
- Check Your Work: Always double-check your calculations to avoid errors. You can use a calculator to verify your answers, but it's important to understand the process and be able to solve the problems manually as well.
- Utilize Online Resources: Numerous online resources, including calculators, tutorials, and practice exercises, are available to help you improve your understanding of percentage calculations.
Conclusion: The Power of Percentages
The seemingly simple question "What percent of 25 will make 15?" unveils the widespread importance and versatility of percentage calculations. From managing personal finances to analyzing complex datasets, understanding percentages is a valuable skill applicable across numerous disciplines and aspects of daily life. Mastering percentage calculations empowers you to make informed decisions, solve problems effectively, and navigate the quantitative aspects of the world around you with greater confidence and accuracy. Remember the fundamental formula, explore different methods of calculation, and practice regularly to build your expertise. The ability to work comfortably with percentages is a skill that will serve you well throughout your life.
Latest Posts
Latest Posts
-
What Percent Of 16 Is 4
Apr 27, 2025
-
Las Olas Son Muy Grandes En La Playa
Apr 27, 2025
-
What Is The Overall Reaction For Cellular Respiration
Apr 27, 2025
-
What Percent Is 6 Out Of 8
Apr 27, 2025
-
How Much Is 2 Quarts In Litres
Apr 27, 2025
Related Post
Thank you for visiting our website which covers about What Percent Of 25 Will Make 15 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.