What Percent Of 65 Is 52
Kalali
May 10, 2025 · 2 min read
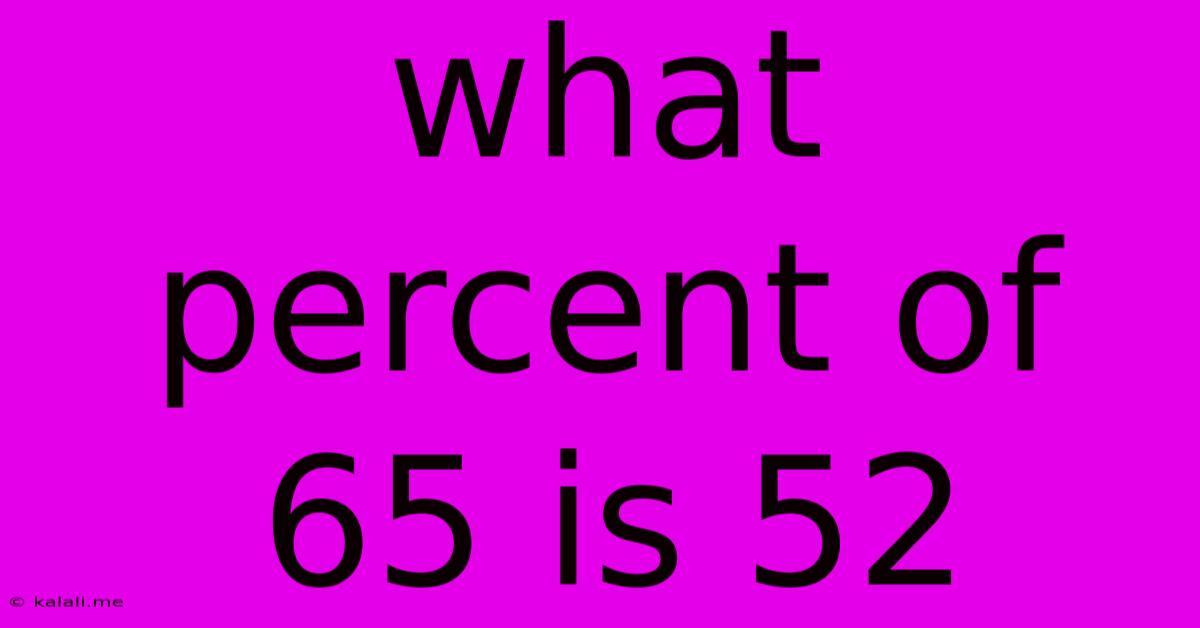
Table of Contents
What Percent of 65 is 52? A Simple Percentage Calculation
Finding what percent one number is of another is a common mathematical task. This article will walk you through how to calculate what percentage 52 represents of 65, providing a step-by-step explanation and offering alternative methods for solving similar problems. Understanding percentage calculations is crucial in various fields, from finance and budgeting to data analysis and everyday life.
Understanding the Problem:
The question "What percent of 65 is 52?" asks us to find the percentage that 52 represents when compared to the whole, which is 65. We need to determine the proportional relationship between these two numbers.
Method 1: Using Proportions
This method involves setting up a proportion to solve for the unknown percentage (let's call it 'x'). The proportion is:
52/65 = x/100
This equation states that the ratio of 52 to 65 is equal to the ratio of x (the percentage we're seeking) to 100 (representing the whole percentage).
To solve for 'x', we cross-multiply:
52 * 100 = 65 * x
5200 = 65x
Now, divide both sides by 65:
x = 5200 / 65
x = 80
Therefore, 52 is 80% of 65.
Method 2: Using Decimal Conversion
This approach involves first finding the decimal equivalent of the fraction 52/65, and then converting that decimal to a percentage.
-
Divide: Divide 52 by 65: 52 ÷ 65 = 0.8
-
Convert to Percentage: Multiply the decimal by 100: 0.8 * 100 = 80%
Again, we find that 52 is 80% of 65.
Method 3: Using the Percentage Formula
The basic percentage formula is:
(Part / Whole) * 100 = Percentage
In this case:
Part = 52 Whole = 65
Substituting these values into the formula:
(52 / 65) * 100 = 80%
This confirms our previous calculations.
Practical Applications:
Understanding percentage calculations is vital in many real-world scenarios:
- Discounts: Calculating the final price of an item after a percentage discount.
- Taxes: Determining the amount of tax payable on a purchase.
- Grade Calculations: Converting test scores to percentages.
- Financial Analysis: Analyzing financial statements and investment returns.
- Data Interpretation: Understanding and interpreting data presented as percentages in charts and graphs.
By mastering these methods, you can confidently solve various percentage problems and apply them to practical situations. Remember to always clearly identify the "part" and the "whole" in the problem to set up your calculations correctly. This understanding of percentage calculations will prove invaluable in your academic and professional pursuits.
Latest Posts
Latest Posts
-
How Many Cups Are In A Pound Of Rice
Jul 10, 2025
-
How Many Pounds Is One Ton Equal
Jul 10, 2025
-
A Warehouse Received 250 Orders In April
Jul 10, 2025
-
How Many Square Inches Are In 1 Square Foot
Jul 10, 2025
-
How Many Square Feet Is 3 4 Acre
Jul 10, 2025
Related Post
Thank you for visiting our website which covers about What Percent Of 65 Is 52 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.