What Percentage Is 4 Of 6
Kalali
Mar 28, 2025 · 5 min read
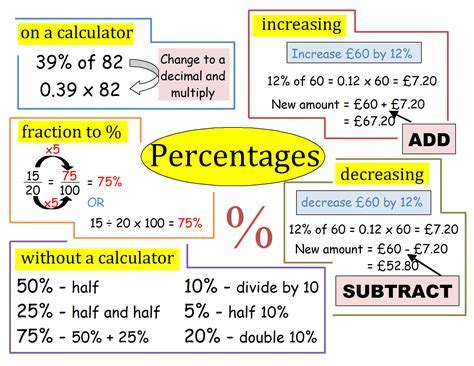
Table of Contents
What Percentage is 4 of 6? A Deep Dive into Percentages and Their Applications
Calculating percentages is a fundamental skill with widespread applications in various aspects of life, from everyday budgeting and shopping to complex financial analyses and scientific research. Understanding how to determine what percentage one number represents of another is crucial for making informed decisions and interpreting data accurately. This article explores the calculation of "What percentage is 4 of 6?" and delves into the broader context of percentage calculations, their practical uses, and potential pitfalls to avoid.
Understanding Percentages: The Basics
A percentage is a way of expressing a number as a fraction of 100. The term "percent" literally means "out of one hundred" (from the Latin per centum). For instance, 50% means 50 out of 100, which is equivalent to the fraction ½ or the decimal 0.5. Percentages provide a standardized method for comparing proportions and making relative comparisons easier to understand.
Calculating "What Percentage is 4 of 6?"
The core question, "What percentage is 4 of 6?", can be solved using a straightforward formula:
(Part / Whole) * 100% = Percentage
In this case:
- Part: 4 (the number we want to express as a percentage)
- Whole: 6 (the total number)
Substituting these values into the formula, we get:
(4 / 6) * 100% = 66.67% (approximately)
Therefore, 4 is approximately 66.67% of 6. The result is a recurring decimal (0.666...), so we typically round it to two decimal places for practical purposes.
Different Approaches to Calculating Percentages
While the above method is the most common and straightforward, there are other ways to approach this calculation:
-
Using decimals: Divide 4 by 6 to get 0.666... Then, multiply by 100 to convert the decimal to a percentage: 0.666... * 100% = 66.67%.
-
Using proportions: Set up a proportion: 4/6 = x/100. Cross-multiply to solve for x: 6x = 400, then x = 400/6 = 66.67%.
-
Using a calculator: Most calculators have a percentage function that simplifies the calculation. Simply enter 4 ÷ 6 = and then multiply by 100.
The choice of method depends on personal preference and the context of the calculation. For simple calculations, the first method (direct substitution into the formula) is often the most efficient. For more complex percentage problems, understanding the underlying principles of proportions can be more beneficial.
Real-World Applications of Percentage Calculations
Percentage calculations are ubiquitous in daily life and professional contexts. Here are a few examples:
-
Discounts and Sales: Stores frequently advertise discounts as percentages (e.g., "20% off"). Knowing how to calculate percentages allows consumers to determine the actual price reduction.
-
Taxes and Interest: Taxes are often calculated as a percentage of income or the value of goods. Similarly, interest on loans or savings accounts is expressed as a percentage rate.
-
Grades and Scores: Academic grades and test scores are frequently represented as percentages, indicating the proportion of correct answers or the level of achievement.
-
Statistics and Data Analysis: Percentages are essential in presenting and interpreting statistical data, allowing for comparisons across different groups or populations. For example, understanding the percentage change in a company's profits year-over-year is crucial for financial analysis.
-
Financial Investments: Return on investment (ROI) is commonly expressed as a percentage, providing a measure of the profitability of an investment.
-
Scientific Research: Percentages are used extensively in scientific experiments and studies to represent proportions and changes in variables. For instance, a researcher might report the percentage of participants who responded positively to a particular treatment.
-
Cooking and Baking: Recipes often use percentages to indicate the proportion of ingredients in a mixture, especially in baking, where precision is important.
Avoiding Common Mistakes in Percentage Calculations
While percentage calculations are relatively straightforward, there are some common mistakes to watch out for:
-
Incorrect identification of the part and the whole: Ensure you correctly identify the number representing the part and the number representing the whole before applying the formula.
-
Rounding errors: When rounding percentages, be mindful of the potential for accumulated errors, especially in more complex calculations. It's usually best to maintain precision until the final step.
-
Confusing percentage change with percentage points: A percentage change represents the relative change between two values, while percentage points refer to the absolute difference between two percentages. For example, an increase from 10% to 20% is a 100% change, but only a 10-percentage-point increase.
-
Misinterpreting percentages: Always carefully consider the context of the percentage. A high percentage in one context might be low in another.
Expanding on the 4 out of 6 Example: Proportions and Ratios
The problem "What percentage is 4 of 6?" fundamentally involves understanding proportions and ratios. A ratio compares two quantities, while a proportion expresses the equality of two ratios. In this case, the ratio of 4 to 6 can be expressed as 4:6 or 4/6. This ratio is equivalent to other ratios, such as 2:3 or 66.67:100 (which is the percentage).
The concept of ratios and proportions extends far beyond simple percentage calculations. They are used in many areas, including:
-
Scaling recipes: If a recipe calls for a 2:1 ratio of flour to sugar, you can scale the recipe up or down by maintaining this ratio.
-
Map scales: Maps use ratios to represent distances on the ground relative to distances on the map.
-
Mixing paints or chemicals: The proportions of different components are crucial in producing desired colors or chemical reactions.
-
Engineering and design: Ratios and proportions are fundamental to structural design, ensuring that the proportions of different components are appropriate for the intended load and stress.
Conclusion: Mastering Percentages for Everyday Life and Beyond
Understanding how to calculate percentages is a critical skill with far-reaching implications. Whether it's managing personal finances, interpreting data, or excelling in academic or professional settings, the ability to accurately calculate and interpret percentages is invaluable. By grasping the fundamental principles outlined in this article, and by practicing different calculation methods, you can confidently approach percentage problems and apply this crucial skill to a wide range of real-world scenarios. Remember to pay close attention to detail, avoid common mistakes, and always consider the context in which percentages are presented to ensure accurate interpretations and informed decision-making. The seemingly simple question, "What percentage is 4 of 6?" thus opens the door to a world of numerical understanding and practical application.
Latest Posts
Latest Posts
-
The Shaft Of The Long Bone Is Called
Mar 31, 2025
-
Cuanto Es 51 Grados Fahrenheit En Centigrados
Mar 31, 2025
-
Cuanto Equivale 32 Onzas En Litros
Mar 31, 2025
-
How Many Feet In 120 In
Mar 31, 2025
-
How Long Is 48 Inches In Cm
Mar 31, 2025
Related Post
Thank you for visiting our website which covers about What Percentage Is 4 Of 6 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
