What Percentage Of 120 Is 20
Kalali
Apr 04, 2025 · 4 min read
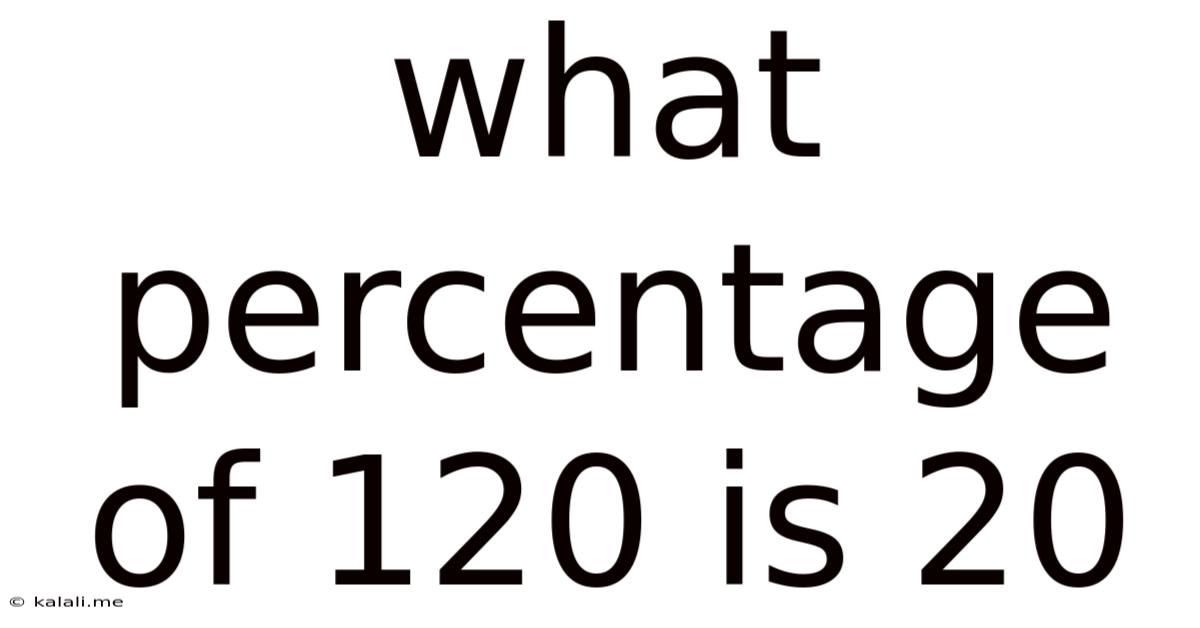
Table of Contents
What Percentage of 120 is 20? A Comprehensive Guide to Percentage Calculations
Understanding percentages is a fundamental skill applicable across numerous fields, from finance and statistics to everyday life. This article delves into the question, "What percentage of 120 is 20?", providing a detailed explanation of the calculation process and exploring various methods to solve similar percentage problems. We'll also examine the broader context of percentage calculations and their real-world applications.
Understanding Percentages: The Basics
A percentage is a way of expressing a number as a fraction of 100. The symbol "%" represents "percent," meaning "per hundred." For example, 50% means 50 out of 100, or 50/100, which simplifies to 1/2 or 0.5.
Understanding this fundamental concept is crucial for tackling percentage calculations. The key elements in any percentage problem are:
- The whole (or total): This is the base number, representing the complete quantity. In our example, the whole is 120.
- The part: This is the smaller portion of the whole that we're interested in. In our example, the part is 20.
- The percentage: This represents the proportional relationship between the part and the whole, expressed as a fraction of 100. This is what we need to calculate.
Method 1: Using the Formula
The most straightforward method for calculating percentages involves a simple formula:
(Part / Whole) * 100 = Percentage
Let's apply this formula to our problem:
(20 / 120) * 100 = Percentage
- Divide the part by the whole: 20 / 120 = 0.166666...
- Multiply the result by 100: 0.166666... * 100 = 16.6666...%
Therefore, 20 is approximately 16.67% of 120. We often round percentages to two decimal places for practicality.
Method 2: Using Proportions
Another effective approach involves setting up a proportion:
Part / Whole = Percentage / 100
Substituting the values from our problem:
20 / 120 = x / 100
To solve for 'x' (the percentage), we can cross-multiply:
20 * 100 = 120 * x
2000 = 120x
x = 2000 / 120
x ≈ 16.67
This confirms that 20 is approximately 16.67% of 120. The proportion method offers a visual representation of the relationship between the parts and the whole.
Method 3: Using Decimal Equivalents
Percentages can also be expressed as decimals. To convert a percentage to a decimal, divide by 100. Conversely, to convert a decimal to a percentage, multiply by 100.
In our problem, we can first convert the percentage to a decimal:
16.67% / 100 = 0.1667
Then, we can verify this by multiplying the decimal by the whole:
0.1667 * 120 ≈ 20
This method emphasizes the interchangeable nature of percentages and decimals, providing flexibility in calculations.
Real-World Applications of Percentage Calculations
Percentage calculations are incredibly versatile and have numerous real-world applications, including:
1. Finance:
- Interest rates: Calculating simple and compound interest requires understanding percentages.
- Discounts and sales tax: Determining the final price of an item after a discount or adding sales tax involves percentage calculations.
- Investment returns: Assessing the profitability of investments depends on calculating percentage returns.
- Loan repayments: Calculating monthly loan payments requires understanding percentages and amortization schedules.
2. Statistics:
- Data analysis: Percentages are used to represent proportions within data sets. For example, analyzing survey results often involves calculating percentages of respondents who chose different options.
- Probability: Probability is often expressed as a percentage.
- Data visualization: Charts and graphs frequently use percentages to display data clearly.
3. Everyday Life:
- Tipping: Calculating a gratuity in a restaurant often involves a percentage of the total bill.
- Sales and discounts: Understanding discounts and sales requires recognizing percentages.
- Cooking: Many recipes utilize percentages when describing ingredient proportions.
- Grading: In academic settings, grades are often expressed as percentages.
Solving Similar Percentage Problems
The methods outlined above can be easily adapted to solve a wide range of percentage problems. Consider these examples:
- What percentage of 50 is 10? Using the formula: (10/50) * 100 = 20%.
- What is 25% of 80? This requires a slight modification: (25/100) * 80 = 20.
- If 30 is 60% of a number, what is the number? This problem requires solving for the whole: 30 / (60/100) = 50.
By consistently applying the formula, proportion method, or decimal equivalents, you can confidently tackle various percentage calculations.
Advanced Percentage Concepts
For a more in-depth understanding of percentages, consider exploring these advanced concepts:
- Percentage increase and decrease: Calculating the percentage change between two values.
- Percentage points: Representing the absolute difference between two percentages.
- Compound interest: Understanding how interest accumulates over time.
- Percentage yield: Calculating the return on an investment, taking into account various factors.
Conclusion
Determining what percentage of 120 is 20 involves a fundamental understanding of percentage calculations. By mastering the formula, proportion method, and decimal equivalents, you can confidently solve this problem and a multitude of similar scenarios. The applications of percentage calculations extend far beyond mathematical exercises, proving essential in numerous personal and professional contexts. Regular practice and exploration of advanced concepts will further enhance your proficiency in this crucial area of mathematics. Remember to always double-check your work and ensure your answer aligns logically with the problem's context. This thorough understanding of percentages will empower you to confidently navigate various quantitative challenges in your daily life.
Latest Posts
Latest Posts
-
What Percent Is 10 Out Of 15
Apr 11, 2025
-
25 Is What Percent Of 70
Apr 11, 2025
-
How Many Degrees Fahrenheit Is 200 Degrees Celsius
Apr 11, 2025
-
27 Inches Is How Many Centimeters
Apr 11, 2025
-
How Do Variations In Temperature Affect The Altimeter
Apr 11, 2025
Related Post
Thank you for visiting our website which covers about What Percentage Of 120 Is 20 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.