What Percentage Of 150 Is 50
Kalali
Apr 26, 2025 · 5 min read
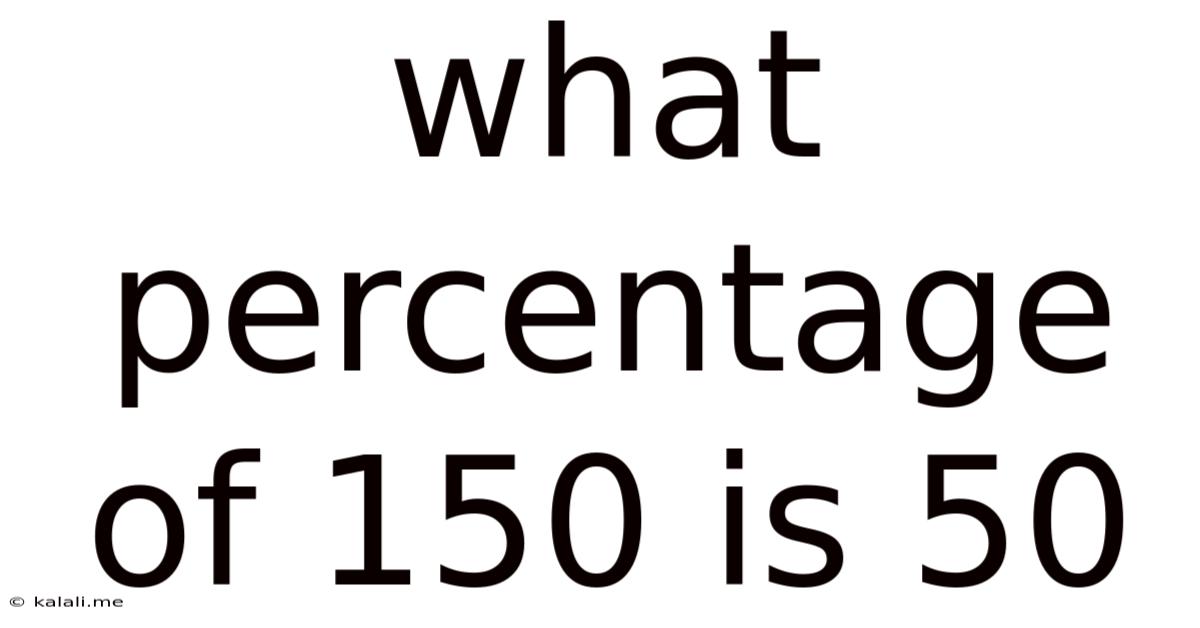
Table of Contents
What Percentage of 150 is 50? A Deep Dive into Percentage Calculations and Real-World Applications
This seemingly simple question, "What percentage of 150 is 50?", opens the door to a broader understanding of percentages, their calculations, and their ubiquitous presence in our daily lives. This article will not only answer the question directly but also explore the underlying mathematical principles, various methods for solving percentage problems, and practical applications across diverse fields. Understanding percentages is crucial for everything from calculating discounts and taxes to analyzing financial reports and understanding statistical data.
Understanding Percentages: The Foundation
A percentage is a way of expressing a number as a fraction of 100. The word "percent" literally means "out of one hundred." Therefore, 50% means 50 out of 100, which can be written as the fraction 50/100 or the decimal 0.5. This fundamental understanding is key to tackling any percentage problem.
Method 1: The Basic Formula
The most straightforward method to determine what percentage of 150 is 50 involves using the basic percentage formula:
(Part / Whole) x 100 = Percentage
In this case:
- Part: 50 (the number we're interested in)
- Whole: 150 (the total number)
Plugging these values into the formula:
(50 / 150) x 100 = 33.33%
Therefore, 50 is approximately 33.33% of 150.
Method 2: Using Proportions
Another effective method utilizes proportions. We can set up a proportion to solve for the unknown percentage (x):
50/150 = x/100
To solve for x, we cross-multiply:
50 * 100 = 150 * x
5000 = 150x
x = 5000 / 150
x = 33.33%
This method reinforces the concept of equivalent ratios and offers an alternative approach to calculating percentages.
Method 3: Decimal Conversion
This method involves converting the fraction to a decimal and then multiplying by 100. First, we express the relationship as a fraction:
50/150
Simplify the fraction by dividing both the numerator and the denominator by their greatest common divisor (50):
1/3
Now, convert the fraction to a decimal by dividing the numerator by the denominator:
1 ÷ 3 ≈ 0.3333
Finally, multiply the decimal by 100 to express it as a percentage:
0.3333 x 100 ≈ 33.33%
This method highlights the interconnectedness between fractions, decimals, and percentages.
Real-World Applications: Where Percentages Matter
The ability to calculate percentages is essential in a multitude of real-world situations. Here are some examples:
-
Retail Discounts: Stores frequently offer discounts on items. If a $150 item is discounted by $50, you can easily calculate the discount percentage using the methods described above. This knowledge helps consumers make informed purchasing decisions.
-
Tax Calculations: Sales tax is calculated as a percentage of the purchase price. Understanding percentages allows you to quickly determine the total cost of an item including tax.
-
Financial Analysis: Investors use percentages extensively to analyze financial statements. Understanding profit margins, return on investment (ROI), and growth rates expressed as percentages is crucial for making sound investment choices. Analyzing percentage changes in stock prices or analyzing year-over-year growth percentages are common practice.
-
Grade Calculations: Many academic grading systems rely on percentages. Students need to understand how their scores translate into percentage grades to assess their academic performance. Percentage scores are critical for understanding academic progress, setting goals, and identifying areas for improvement.
-
Statistical Analysis: Percentages are fundamental in statistical data representation. Opinion polls, survey results, and market research often present data in percentage terms. Understanding how to interpret this data is key to drawing meaningful conclusions. For instance, understanding the percentage of respondents who prefer a certain product helps market research analysts to understand consumer preferences.
-
Tip Calculations: Determining the appropriate tip amount in restaurants often involves calculating a percentage of the total bill. Knowing how to quickly calculate these percentages ensures fair compensation and avoids calculation errors.
Beyond the Basics: Advanced Percentage Calculations
While the above examples focus on straightforward percentage calculations, the concepts can be extended to more complex scenarios. This includes:
-
Percentage Increase/Decrease: Calculating the percentage change between two values requires understanding how to determine the difference, divide by the original value, and multiply by 100. For instance, determining the percentage increase or decrease in profits from one year to another requires comparing the differences in profit figures to determine the growth rate.
-
Compound Interest: Compound interest calculations involve repeatedly applying a percentage increase to a principal amount over time. Understanding this concept is crucial for planning long-term investments and understanding the power of compounding.
-
Percentage Points: It's important to distinguish between percentage points and percentage change. A change from 20% to 30% is a 10 percentage point increase, but a 50% percentage increase. This distinction is vital for accurate interpretation of data in many contexts, especially when reporting on change, growth, or improvement.
Conclusion: The Power of Percentages
The seemingly simple question of "What percentage of 150 is 50?" serves as a gateway to a deeper understanding of percentages and their widespread applications. Mastering percentage calculations is not just a mathematical skill; it's a practical life skill. From personal finances to professional analysis, understanding percentages empowers you to make informed decisions, interpret data effectively, and navigate the numerical world with confidence. The methods described in this article provide a solid foundation for tackling various percentage problems, allowing you to confidently apply this essential skill in countless situations. Remember to practice regularly and explore different methods to solidify your understanding and build a strong grasp of this fundamental mathematical concept.
Latest Posts
Latest Posts
-
5 Conditions Of Hardy Weinberg Equilibrium
Apr 26, 2025
-
What Is 0 83 As A Fraction
Apr 26, 2025
-
What Percent Of 50 Is 36
Apr 26, 2025
-
What Is 31 Out Of 40 As A Percentage
Apr 26, 2025
-
How Long Does It Take For Mountains To Form
Apr 26, 2025
Related Post
Thank you for visiting our website which covers about What Percentage Of 150 Is 50 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.