What Percentage Of 40 Is 5
Kalali
Apr 15, 2025 · 4 min read
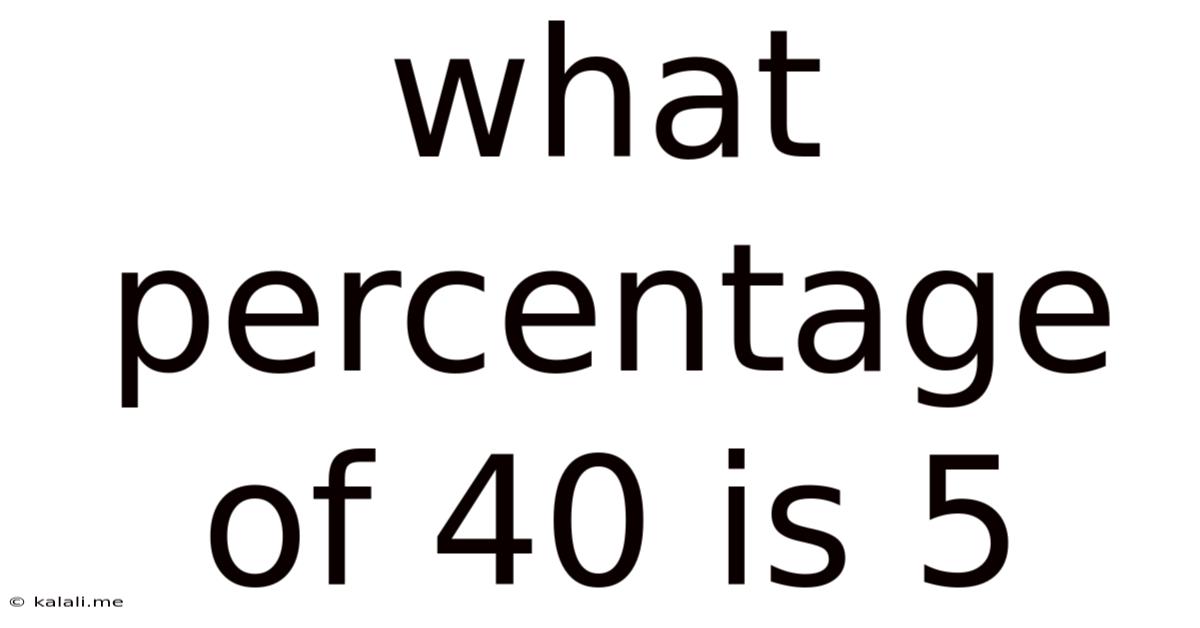
Table of Contents
What Percentage of 40 is 5? A Comprehensive Guide to Percentage Calculations
Finding what percentage 5 represents of 40 might seem like a simple arithmetic problem, but understanding the underlying concepts is crucial for a wide range of applications, from everyday budgeting and shopping to complex financial analysis and scientific research. This article will delve into multiple ways to solve this problem, explain the fundamental principles of percentage calculations, and explore its relevance in various real-world scenarios. We'll also look at variations on this problem to solidify your understanding. This comprehensive guide ensures you not only get the answer but also master the concept of percentages.
Meta Description: Learn how to calculate percentages with a step-by-step guide demonstrating how to determine what percentage 5 is of 40. Explore different methods and real-world applications of percentage calculations.
Understanding Percentages: The Basics
A percentage is a fraction or ratio expressed as a number out of 100. The symbol "%" signifies "percent" or "out of one hundred." Percentages are a convenient way to represent proportions and comparisons. They're widely used because they provide a standardized way of expressing parts of a whole, regardless of the size of the whole.
Method 1: Using the Formula
The most direct way to calculate what percentage 5 is of 40 is using the basic percentage formula:
(Part / Whole) * 100 = Percentage
In this case:
- Part: 5
- Whole: 40
Substituting these values into the formula:
(5 / 40) * 100 = 12.5%
Therefore, 5 is 12.5% of 40.
Method 2: Simplifying the Fraction
Before multiplying by 100, we can simplify the fraction (5/40):
5/40 simplifies to 1/8.
Now, convert the fraction 1/8 to a percentage:
(1/8) * 100 = 12.5%
This method demonstrates that simplifying fractions can make the calculation easier and less prone to errors, especially with larger numbers.
Method 3: Using Proportions
We can also solve this using proportions:
We set up a proportion:
5/40 = x/100
Where 'x' represents the percentage we're trying to find. To solve for x, we cross-multiply:
40x = 500
x = 500 / 40
x = 12.5
Therefore, 5 is 12.5% of 40.
Real-World Applications: Where Percentage Calculations Matter
Understanding percentage calculations is essential in numerous real-world situations. Here are a few examples:
-
Finance: Calculating interest rates, discounts, tax percentages, profit margins, and investment returns all rely heavily on percentage calculations. For example, if a bank offers a 5% interest rate on a savings account, knowing how to calculate percentages helps determine the interest earned on your deposits.
-
Retail and Sales: Discounts are expressed as percentages. If a store offers a 20% discount on an item, calculating the final price requires understanding percentages. Similarly, sales tax is calculated as a percentage of the purchase price.
-
Data Analysis and Statistics: Percentages are fundamental in presenting and interpreting data. Analyzing survey results, understanding market share, and interpreting scientific data often involves calculating and comparing percentages.
-
Everyday Life: Calculating tips in restaurants, understanding nutritional information on food labels (e.g., percentage of daily recommended values), and even figuring out the percentage of a task completed all involve percentage calculations.
Variations and Advanced Percentage Problems
Let's explore some variations of this basic percentage problem to further enhance your understanding:
1. Finding the Whole: If 15% of a number is 6, what is the number?
We can set up the equation:
0.15 * x = 6
Solving for x:
x = 6 / 0.15 = 40
Therefore, the number is 40. This demonstrates the inverse operation of finding a percentage.
2. Finding the Part: What is 25% of 80?
Using the formula:
(25/100) * 80 = 20
Therefore, 25% of 80 is 20.
3. Percentage Increase/Decrease: A product's price increased from $40 to $45. What is the percentage increase?
First, find the difference: $45 - $40 = $5
Then, divide the difference by the original price and multiply by 100:
(5 / 40) * 100 = 12.5%
The price increased by 12.5%.
Avoiding Common Mistakes in Percentage Calculations
Several common mistakes can occur when working with percentages:
-
Incorrect order of operations: Always follow the order of operations (PEMDAS/BODMAS). Parentheses, exponents, multiplication and division (from left to right), then addition and subtraction (from left to right).
-
Confusing percentages with decimals: Remember to convert percentages to decimals (divide by 100) before performing calculations. For example, 25% is equivalent to 0.25.
-
Using the wrong base: When calculating percentage increases or decreases, always use the original value as the base.
-
Rounding errors: Be mindful of rounding errors, especially in complex calculations. It's often best to carry extra decimal places during calculations and only round the final answer.
Conclusion: Mastering Percentage Calculations
Understanding how to calculate percentages is a fundamental skill applicable across various disciplines. While the problem of finding what percentage 5 is of 40 might seem simple, mastering the underlying principles and various methods opens doors to more complex percentage-related problems. By practicing different approaches and understanding common pitfalls, you can confidently tackle percentage calculations in various real-world situations and enhance your analytical abilities. Remember to practice regularly to solidify your understanding and improve your speed and accuracy. The ability to confidently and accurately calculate percentages is a valuable asset in many aspects of life.
Latest Posts
Latest Posts
-
How Much Force Is Needed To Accelerate
Apr 17, 2025
-
Least Common Multiple Of 8 And 4
Apr 17, 2025
-
How Many Liters Is 70 Oz
Apr 17, 2025
-
17 3 As A Mixed Number
Apr 17, 2025
-
Can A Right Triangle Be Equilateral
Apr 17, 2025
Related Post
Thank you for visiting our website which covers about What Percentage Of 40 Is 5 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.