2 Out Of 12 As A Percentage
Kalali
Apr 17, 2025 · 5 min read
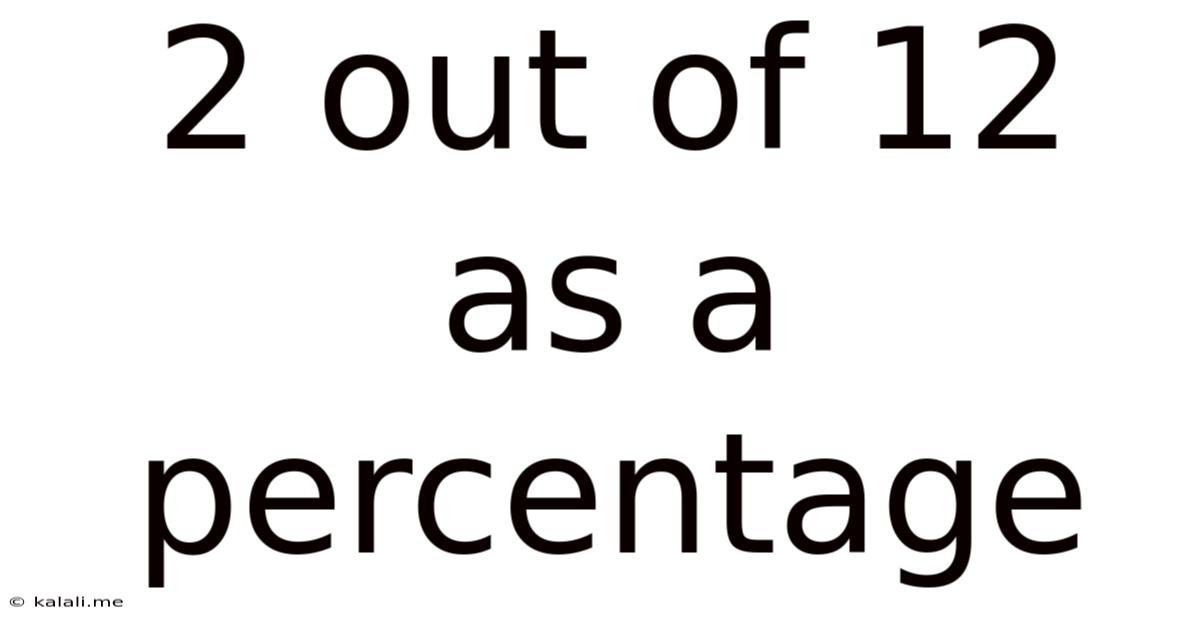
Table of Contents
2 Out of 12 as a Percentage: A Comprehensive Guide to Percentage Calculations
Calculating percentages is a fundamental skill in various aspects of life, from academic studies to professional careers. Understanding how to express fractions as percentages is crucial for interpreting data, making comparisons, and presenting information effectively. This article delves into the specifics of calculating "2 out of 12 as a percentage," providing a step-by-step guide, exploring related concepts, and offering practical applications. This detailed explanation will also cover different methods and demonstrate how to solve similar problems. By the end, you’ll not only know the answer but also grasp the underlying principles of percentage calculations.
Meta Description: Learn how to calculate 2 out of 12 as a percentage. This comprehensive guide explains the process step-by-step, covers different methods, and explores practical applications of percentage calculations.
Understanding the Basics of Percentages
Before diving into the specific calculation, let's refresh our understanding of percentages. A percentage is a fraction or ratio expressed as a number out of 100. The symbol "%" represents "percent," meaning "out of one hundred." Essentially, a percentage indicates a proportional part of a whole. For instance, 50% represents 50 parts out of 100, which is equivalent to ½ or 0.5.
Method 1: The Fraction Method
The most straightforward approach to calculating "2 out of 12 as a percentage" involves expressing the ratio as a fraction and then converting it to a percentage.
-
Express the ratio as a fraction: The phrase "2 out of 12" directly translates to the fraction 2/12.
-
Simplify the fraction (if possible): Both the numerator (2) and the denominator (12) are divisible by 2. Simplifying the fraction gives us 1/6.
-
Convert the fraction to a decimal: To convert the fraction 1/6 to a decimal, we divide the numerator (1) by the denominator (6): 1 ÷ 6 ≈ 0.1667 (we round to four decimal places for accuracy).
-
Convert the decimal to a percentage: To convert a decimal to a percentage, we multiply the decimal by 100 and add the "%" symbol: 0.1667 × 100 = 16.67%.
Therefore, 2 out of 12 is approximately 16.67%.
Method 2: The Proportion Method
This method uses proportions to solve the problem. We can set up a proportion to find the percentage:
-
We know that 2 out of 12 represents a certain percentage (x%) out of 100%. This can be written as a proportion:
2/12 = x/100
-
To solve for x, we cross-multiply:
12x = 200
-
Now, divide both sides by 12:
x = 200/12 ≈ 16.67
-
Therefore, x ≈ 16.67%, confirming our previous result.
Method 3: Using a Calculator
Most calculators have a percentage function. You can directly input the fraction 2/12 and press the percentage button (%) to obtain the result: 16.67%. This method is quick and convenient, especially for more complex calculations.
Practical Applications of Percentage Calculations
Understanding percentage calculations is invaluable in numerous real-world scenarios:
-
Finance: Calculating interest rates, discounts, tax percentages, profit margins, and investment returns all rely on percentage calculations. For instance, understanding the percentage change in stock prices or calculating the interest accrued on savings accounts directly involves percentage calculations. A 10% discount on a $100 item translates to a $10 saving.
-
Statistics and Data Analysis: Percentages are crucial for representing data in a clear and concise manner. For example, researchers often use percentages to express survey results, representing the proportion of respondents who chose a particular option. Analyzing election results, where the percentage of votes received by each candidate is key, relies heavily on percentage calculations.
-
Science: In scientific studies, percentages are frequently used to express the concentration of solutions, the success rate of experiments, or the prevalence of a particular characteristic within a population.
-
Everyday Life: We encounter percentages daily, whether it's calculating tips in restaurants (e.g., a 15% tip), understanding sale prices in stores (e.g., a 20% off sale), or interpreting nutritional information on food labels (e.g., the percentage of daily value of certain nutrients).
Understanding Percentage Change
Often, we need to calculate the percentage change between two values. This is particularly useful when comparing values over time or across different categories. The formula for percentage change is:
Percentage Change = [(New Value - Old Value) / Old Value] × 100%
For example, if your sales increased from $10,000 to $12,000, the percentage change would be:
[(12000 - 10000) / 10000] × 100% = 20%
This indicates a 20% increase in sales.
Solving Similar Problems
The methods outlined above can be applied to solve various similar problems involving percentage calculations. For instance:
- 3 out of 15: This simplifies to 1/5, which is 20%.
- 5 out of 25: This simplifies to 1/5, which is 20%.
- 8 out of 40: This simplifies to 1/5, which is 20%.
- 10 out of 50: This simplifies to 1/5, which is 20%.
- 1 out of 3: This is approximately 33.33%.
- 4 out of 5: This is 80%.
By consistently applying these methods, you can efficiently determine the percentage representation of various ratios.
Advanced Percentage Calculations
While the examples above focus on simpler calculations, percentages can be applied to more complex scenarios. These can involve:
-
Compound percentages: This deals with applying a percentage to a value, and then applying a further percentage to the resulting value. This is commonly encountered in compound interest calculations.
-
Percentage increase/decrease over multiple periods: Calculating the overall percentage change when there are multiple periods of increase or decrease.
-
Weighted averages: This involves calculating the average of values, where each value has a different weight or importance, often expressed as a percentage.
Conclusion
Calculating "2 out of 12 as a percentage" is a straightforward process, but understanding the underlying principles is crucial for applying these calculations in various contexts. Whether using the fraction method, the proportion method, or a calculator, the answer remains consistent: approximately 16.67%. Mastering percentage calculations enhances your ability to interpret data, solve problems, and make informed decisions in various aspects of your life, from personal finance to professional endeavors. The ability to accurately and confidently perform these calculations is a valuable asset in today's data-driven world. Remember to practice these methods to build confidence and proficiency in percentage calculations. The more you practice, the easier it becomes to tackle more complex percentage problems.
Latest Posts
Latest Posts
-
What Percent Is 14 Out Of 20
Apr 19, 2025
-
What Is 3 Percent Of 10000
Apr 19, 2025
-
4 Liters Is How Many Milliliters
Apr 19, 2025
-
Cuanto Es 30 Grados Centigrados En Fahrenheit
Apr 19, 2025
-
22 15 As A Mixed Number
Apr 19, 2025
Related Post
Thank you for visiting our website which covers about 2 Out Of 12 As A Percentage . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.