2 Out Of 7 Is What Percent
Kalali
Apr 22, 2025 · 5 min read
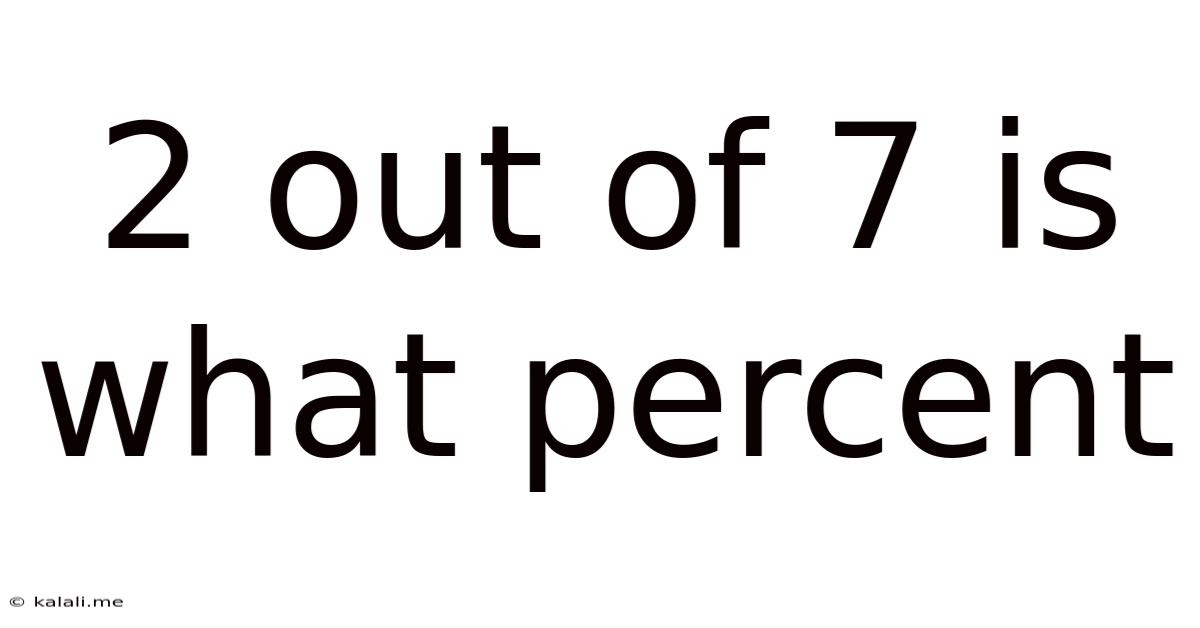
Table of Contents
2 Out of 7 is What Percent? A Comprehensive Guide to Percentage Calculations
Calculating percentages is a fundamental skill in many aspects of life, from understanding sales discounts to analyzing statistical data. This comprehensive guide will not only answer the question "2 out of 7 is what percent?" but will also equip you with the knowledge and tools to tackle similar percentage problems with confidence. We'll explore various methods for calculating percentages, discuss their applications, and delve into some common pitfalls to avoid. This article will serve as your definitive resource for mastering percentage calculations.
Understanding Percentages:
Before diving into the specific calculation, let's establish a solid foundation in understanding percentages. A percentage is simply a fraction where the denominator is 100. It represents a portion of a whole, expressed as a number out of 100. The symbol "%" is used to denote a percentage. For instance, 50% means 50 out of 100, or 50/100, which simplifies to 1/2.
Methods for Calculating Percentages:
There are several methods to calculate percentages, each offering a slightly different approach. Let's explore three common methods:
1. The Fraction Method:
This is arguably the most intuitive method, especially for simple calculations like "2 out of 7 is what percent?". We express the problem as a fraction: 2/7. To convert this fraction to a percentage, we need to find an equivalent fraction with a denominator of 100.
We can set up a proportion:
2/7 = x/100
To solve for x, we cross-multiply:
7x = 200
x = 200/7
x ≈ 28.57
Therefore, 2 out of 7 is approximately 28.57%.
2. The Decimal Method:
This method involves converting the fraction to a decimal and then multiplying by 100. Following the same example:
2/7 ≈ 0.2857
0.2857 * 100 = 28.57%
This method is efficient and readily adaptable to calculations using a calculator.
3. Using a Calculator:
Most calculators have a percentage function. Simply divide the part (2) by the whole (7) and then multiply by 100. This eliminates the need for manual calculations, making it the fastest method for larger or more complex problems. The result, as before, is approximately 28.57%.
Rounding and Precision:
When dealing with percentages, you'll often encounter decimal values. It's crucial to understand the concept of rounding. In our example, we rounded the result to two decimal places (28.57%). The level of precision depends on the context of the problem. For some applications, rounding to the nearest whole number (29%) might be sufficient. For others, greater precision might be required.
Practical Applications of Percentage Calculations:
Percentage calculations are ubiquitous in various fields:
-
Finance: Calculating interest rates, discounts, profit margins, tax rates, and investment returns. Understanding percentages is critical for making informed financial decisions. For example, a 10% discount on a $100 item means a saving of $10.
-
Statistics: Analyzing data sets, representing proportions, and understanding probabilities. Percentages are used to present data clearly and concisely, making it easier to interpret trends and patterns. For example, if a survey shows that 60% of respondents prefer a particular product, it demonstrates a strong preference.
-
Science: Expressing concentrations, measuring changes, and representing experimental results. For example, a solution with a 5% concentration of salt means 5 grams of salt per 100ml of solution.
-
Everyday Life: Calculating tips, understanding sales, determining nutritional values, and comparing prices. Percentages are frequently encountered in daily life, making it essential to understand their meaning and calculation. For instance, a 15% tip on a $50 meal would be $7.50.
-
Business: Calculating market share, analyzing sales data, evaluating performance metrics, and forecasting future trends. Business decisions heavily rely on accurate percentage calculations. Understanding profit margins, cost-of-goods sold and revenue as percentages is fundamental for business success.
Common Mistakes to Avoid:
-
Incorrect Order of Operations: When using the fraction method, ensure you correctly set up the proportion and solve for the unknown variable.
-
Rounding Errors: Be mindful of rounding errors, especially in multi-step calculations. Rounding prematurely can lead to significant inaccuracies in the final result.
-
Misinterpreting the Question: Carefully read and understand the problem statement. Identify the part and the whole accurately before attempting the calculation.
-
Using the Wrong Formula: Ensure you use the correct formula for the type of percentage calculation you are performing.
Expanding on Percentage Calculations:
Let's explore some more complex scenarios involving percentage calculations:
-
Finding the Percentage Increase or Decrease: This involves calculating the percentage change between two values. The formula is: [(New Value - Old Value) / Old Value] * 100. For example, if a product's price increases from $50 to $60, the percentage increase is [(60 - 50) / 50] * 100 = 20%.
-
Calculating the Original Value: If you know the percentage change and the new value, you can work backward to find the original value. For example, if a product is discounted by 20% and costs $80 after the discount, the original price was $100.
-
Calculating Percentage Points: Percentage points represent the arithmetic difference between two percentages. For example, if interest rates increase from 2% to 5%, the increase is 3 percentage points, not 3%.
-
Compound Interest: This involves earning interest on both the principal amount and accumulated interest. Compound interest calculations require a more complex formula but are essential for understanding investment growth.
-
Percentage of a Percentage: Calculating a percentage of a percentage involves multiplying the percentages together. For instance, finding 10% of 20% means 0.10 * 0.20 = 0.02 or 2%.
Conclusion:
Calculating percentages is a versatile skill applicable across numerous fields. While seemingly simple, understanding the various methods and potential pitfalls ensures accurate and confident calculations. This comprehensive guide has provided you with the tools and knowledge to tackle percentage problems effectively, whether it’s determining what percentage 2 out of 7 represents or engaging with more complex percentage-related tasks. Mastering percentages is key to successful problem-solving in both academic and professional settings. Remember to practice regularly and always double-check your calculations to ensure accuracy.
Latest Posts
Latest Posts
-
What Is 8 12 As A Percentage
Apr 22, 2025
-
2 Out Of 3 As A Percentage
Apr 22, 2025
-
How Many Liters In 64 Ounces
Apr 22, 2025
-
Cuanto Son 70 Grados Fahrenheit En Centigrados
Apr 22, 2025
-
Common Multiples Of 5 And 15
Apr 22, 2025
Related Post
Thank you for visiting our website which covers about 2 Out Of 7 Is What Percent . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.