31 Is What Percent Of 40
Kalali
Apr 18, 2025 · 5 min read
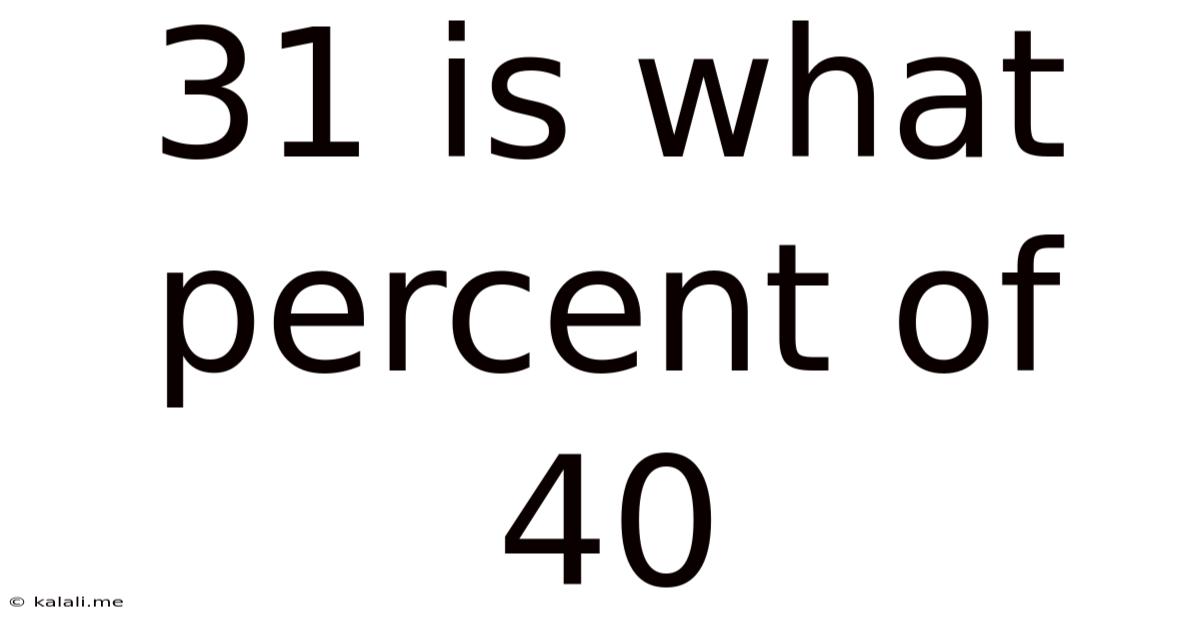
Table of Contents
31 is What Percent of 40: A Comprehensive Guide to Percentage Calculations
This article delves into the seemingly simple question: "31 is what percent of 40?" While the answer itself is straightforward, the underlying principles extend far beyond this specific problem. We'll explore multiple methods for solving this type of percentage calculation, discuss the importance of percentages in various fields, and offer practical applications to solidify your understanding. This comprehensive guide will empower you to confidently tackle any percentage problem you encounter.
Meta Description: Learn how to calculate percentages with a detailed explanation of how to determine what percent 31 is of 40. This guide covers multiple methods and real-world applications of percentage calculations.
Understanding Percentages: The Foundation
Before diving into the calculation, let's establish a firm understanding of percentages. A percentage is a fraction or ratio expressed as a number out of 100. The symbol "%" represents "per hundred" or "out of 100." Therefore, 50% means 50 out of 100, which is equivalent to ½ or 0.5. Understanding this fundamental concept is crucial for mastering percentage calculations. Many everyday scenarios involve percentages, including discounts, tax rates, interest rates, and statistical data.
Method 1: Using Proportions
This method leverages the concept of ratios and proportions to solve the percentage problem. We can set up a proportion to represent the relationship between the parts and the whole:
- Part/Whole = Percentage/100
In our case:
- 31/40 = x/100
To solve for 'x' (the percentage), we cross-multiply:
- 31 * 100 = 40 * x
- 3100 = 40x
Now, divide both sides by 40:
- x = 3100 / 40
- x = 77.5
Therefore, 31 is 77.5% of 40.
Method 2: Using Decimal Conversion
This method involves converting the fraction to a decimal and then multiplying by 100 to express it as a percentage. We start with the fraction representing the problem:
- 31/40
Now, we divide 31 by 40:
- 31 ÷ 40 = 0.775
Finally, multiply the decimal by 100 to convert it to a percentage:
- 0.775 * 100 = 77.5%
This confirms that 31 is 77.5% of 40. This method is often quicker for simple calculations.
Method 3: Using a Calculator
Most calculators have a percentage function that simplifies the process significantly. Simply enter "31 ÷ 40" and then multiply the result by 100. The calculator will directly provide the answer: 77.5%. This is the most efficient method for quick calculations, especially when dealing with larger or more complex numbers.
Real-World Applications of Percentage Calculations
The ability to calculate percentages is essential in numerous real-world scenarios. Let's explore some examples:
-
Retail Discounts: Imagine a store offering a 20% discount on a $50 item. To calculate the discount, you'd find 20% of $50 (0.20 * $50 = $10), and then subtract the discount from the original price ($50 - $10 = $40). Understanding percentage discounts is crucial for savvy shopping.
-
Tax Calculations: Sales tax is often expressed as a percentage of the purchase price. For example, a 6% sales tax on a $100 item would be calculated as 0.06 * $100 = $6. Knowing how to calculate sales tax is vital for budgeting and financial planning.
-
Interest Rates: Interest on loans and savings accounts is usually quoted as an annual percentage rate (APR). Calculating the interest earned or owed requires understanding percentage calculations. This knowledge is essential for making informed financial decisions.
-
Grade Calculation: Many educational systems use percentages to represent grades. For example, a student scoring 25 out of 30 on a test could calculate their percentage score using the methods described above, revealing their grade.
-
Statistical Analysis: Percentages are frequently used in data analysis to represent proportions and trends. For instance, understanding the percentage of people who prefer a certain product can inform marketing strategies.
-
Financial Investments: Investment returns are typically expressed as percentages. Calculating the percentage increase or decrease in the value of an investment is crucial for monitoring performance and making investment decisions.
-
Data Representation in Charts and Graphs: Percentages are a common way to represent data in pie charts, bar graphs, and other visual aids, making them easier to understand and interpret.
Beyond the Basics: More Complex Percentage Problems
While the example "31 is what percent of 40" is relatively straightforward, percentage problems can become more complex. Here are some variations:
-
Finding the Whole: If you know a percentage and the part, you can calculate the whole. For example, if 25% of a number is 10, you can set up the equation: 0.25 * x = 10, and solve for x.
-
Finding the Part: If you know a percentage and the whole, you can calculate the part. For example, to find 15% of 80, you would calculate 0.15 * 80.
-
Percentage Increase/Decrease: Calculating percentage changes involves finding the difference between two values, dividing by the original value, and multiplying by 100. This is common in finance and economics.
-
Compound Interest: This involves calculating interest on both the principal amount and accumulated interest from previous periods. It's a more complex application of percentage calculations.
Mastering Percentage Calculations: Tips and Tricks
Here are some tips to improve your proficiency with percentage calculations:
-
Practice Regularly: The best way to master any skill is through consistent practice. Solve various percentage problems to build your confidence and speed.
-
Understand the Concepts: Don't just memorize formulas; understand the underlying principles of percentages and ratios. This will allow you to approach problems from different angles.
-
Use Different Methods: Experiment with different methods (proportions, decimal conversion, calculator) to find the approach that works best for you.
-
Check Your Work: Always verify your answers using a different method or by estimating the result. This helps prevent errors and build accuracy.
-
Utilize Online Resources: Numerous websites and apps offer practice problems and tutorials on percentage calculations. These can be valuable tools for learning and improvement.
In conclusion, understanding how to calculate percentages is a fundamental skill with widespread applications in various aspects of life. While the question "31 is what percent of 40?" serves as a starting point, this article provides a thorough exploration of the underlying principles, diverse calculation methods, and practical applications of percentages. By mastering these concepts, you'll be well-equipped to tackle any percentage problem with confidence and efficiency. Remember to practice regularly and utilize the resources available to solidify your understanding and become proficient in this essential skill.
Latest Posts
Latest Posts
-
How To Turn 2 5 8 Into A Decimal
Apr 22, 2025
-
What Is 17 12 As A Mixed Number
Apr 22, 2025
-
2 Out Of 7 Is What Percent
Apr 22, 2025
-
What Is 30 100 As A Percent
Apr 22, 2025
-
How To Find The Range In A Dot Plot
Apr 22, 2025
Related Post
Thank you for visiting our website which covers about 31 Is What Percent Of 40 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.