7 Is What Percent Of 9
Kalali
Apr 01, 2025 · 5 min read
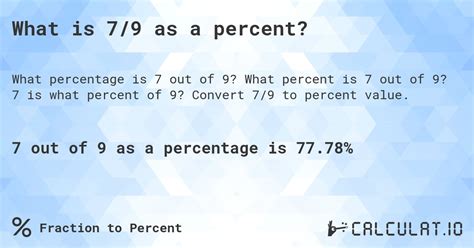
Table of Contents
7 is What Percent of 9? A Comprehensive Guide to Percentage Calculations
Understanding percentages is a fundamental skill in various aspects of life, from calculating discounts and taxes to comprehending statistical data and financial reports. This article will delve into the seemingly simple question, "7 is what percent of 9?", providing a detailed explanation of the calculation process, exploring different methods, and illustrating the broader applications of percentage calculations.
Understanding Percentages
A percentage is a fraction or ratio expressed as a number out of 100. The symbol "%" represents "per cent," which literally means "out of one hundred." Therefore, 50% means 50 out of 100, which can be simplified to 1/2 or 0.5.
Calculating "7 is What Percent of 9?"
To determine what percentage 7 represents of 9, we can employ several methods. The most straightforward approach involves using a simple formula:
(Part / Whole) x 100% = Percentage
In this case:
- Part: 7
- Whole: 9
Substituting these values into the formula, we get:
(7 / 9) x 100% = 77.78% (approximately)
Therefore, 7 is approximately 77.78% of 9.
Alternative Methods for Percentage Calculation
While the above method is the most common and arguably the easiest, there are other ways to approach this problem:
Method 2: Using Proportions
We can set up a proportion to solve this problem:
7/9 = x/100
Here, 'x' represents the percentage we're trying to find. To solve for 'x', we cross-multiply:
9x = 700
x = 700/9
x ≈ 77.78%
This method demonstrates the relationship between fractions and percentages, highlighting the fundamental principles behind percentage calculations.
Method 3: Decimal Conversion
First, convert the fraction 7/9 into a decimal by dividing 7 by 9:
7 ÷ 9 ≈ 0.7778
Then, multiply the decimal by 100% to express it as a percentage:
0.7778 x 100% ≈ 77.78%
Practical Applications of Percentage Calculations
The ability to calculate percentages accurately has numerous real-world applications. Here are just a few examples:
1. Financial Calculations:
-
Interest Rates: Banks and financial institutions use percentages to calculate interest on loans and savings accounts. Understanding percentages is crucial for managing personal finances and making informed decisions about borrowing and investing. For instance, if you invest $1000 at an annual interest rate of 5%, you'll earn $50 in interest after one year.
-
Discounts and Sales Tax: Retailers utilize percentages to advertise discounts and apply sales taxes. Knowing how to calculate percentages allows consumers to determine the final price of an item after a discount or the total cost including sales tax. For example, a 20% discount on a $50 item means you save $10, paying only $40.
-
Profit Margins: Businesses use percentage calculations to determine their profit margins, which indicates the profitability of their products or services. A high profit margin suggests healthy financial performance, while a low margin might warrant a review of pricing strategies.
2. Statistical Analysis:
-
Data Interpretation: Percentages are frequently used to present data in a clear and concise manner. In surveys, for example, results are often expressed as percentages to demonstrate the proportion of respondents who answered in a particular way.
-
Growth Rates: Percentages are essential for tracking growth rates in various fields, from population growth to economic indicators. For instance, a 3% increase in GDP indicates that the economy grew by 3% compared to the previous period.
-
Probability and Statistics: Probability is often expressed as a percentage, reflecting the likelihood of an event occurring. For example, a 70% chance of rain indicates a relatively high probability of precipitation.
3. Everyday Applications:
-
Tip Calculations: When dining out, calculating a tip (usually expressed as a percentage of the bill) is a common application of percentages. A 15% tip on a $50 meal would be $7.50.
-
Recipe Scaling: Scaling recipes up or down involves adjusting ingredient quantities proportionally, frequently using percentage calculations. If a recipe calls for 100g of flour and you want to increase it by 25%, you would need 125g of flour.
-
Grading Systems: Many educational systems use percentages to represent grades, reflecting the proportion of correct answers or the overall performance in a course. A grade of 85% indicates a strong understanding of the subject matter.
Beyond the Basics: Handling More Complex Percentage Problems
While the "7 is what percent of 9?" problem is relatively straightforward, understanding percentage calculations expands to encompass more complex scenarios. These might involve:
-
Finding the whole when given the part and percentage: For example, if 20% of a number is 10, what is the number?
-
Finding the part when given the whole and percentage: For example, what is 30% of 50?
-
Calculating percentage increase or decrease: This involves determining the percentage change between two values. For example, if a price increased from $10 to $12, the percentage increase is 20%.
-
Working with multiple percentages: This often involves calculating compound percentages, such as applying successive discounts or interest rates.
Conclusion: Mastering Percentage Calculations
Understanding how to calculate percentages is a valuable skill with wide-ranging applications. While the simple calculation of "7 is what percent of 9?" provides a basic understanding, the principles involved extend far beyond this particular example. By mastering percentage calculations, you gain the ability to navigate various financial, statistical, and everyday situations more effectively. Remember to practice regularly to solidify your understanding and improve your speed and accuracy in solving percentage-related problems. The more you practice, the more comfortable and confident you'll become in tackling various percentage calculations, making them an integral part of your problem-solving toolkit.
Latest Posts
Latest Posts
-
Cuanto Es 4 Metros En Pies
Apr 02, 2025
-
How Many Milliliters In 8 Cups
Apr 02, 2025
-
Do Nonpolar Molecules Attract Each Other
Apr 02, 2025
-
How Many Liters Is 750 Milliliters
Apr 02, 2025
-
What Is The Least Common Multiple Of 10 And 15
Apr 02, 2025
Related Post
Thank you for visiting our website which covers about 7 Is What Percent Of 9 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
