Integral Of 1 X 2 3
Kalali
May 24, 2025 · 2 min read
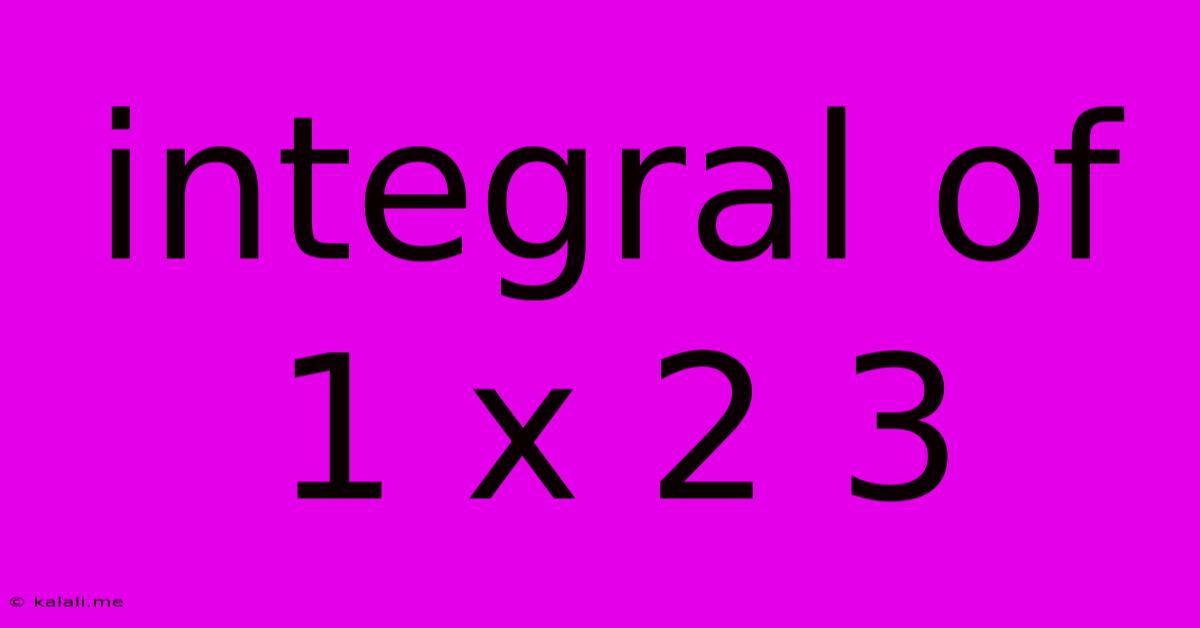
Table of Contents
Understanding and Solving the Integral of 1, x, x², and x³
This article will guide you through the process of solving the indefinite integrals of 1, x, x², and x³. Understanding these fundamental integrals is crucial for mastering calculus and tackling more complex integration problems. We'll explore the power rule of integration, a key concept in solving these types of problems. This will be helpful for students learning calculus, as well as anyone needing a refresher on basic integration techniques.
What is an Indefinite Integral?
Before diving into the specifics, let's clarify what an indefinite integral is. It's the reverse process of differentiation. While differentiation finds the rate of change of a function, integration finds the function whose derivative is the given function. The indefinite integral is represented by the symbol ∫ and always includes a constant of integration, "+C," because the derivative of a constant is zero.
The Power Rule of Integration
The power rule is your go-to method for integrating polynomial terms. The rule states:
∫xⁿ dx = (xⁿ⁺¹)/(n+1) + C
where 'n' is any real number except -1, and 'C' represents the constant of integration.
Solving the Integrals
Let's apply the power rule to each of our target integrals:
1. The Integral of 1 (∫1 dx)
Here, we can rewrite 1 as x⁰. Applying the power rule:
∫x⁰ dx = (x⁰⁺¹)/(0+1) + C = x + C
Therefore, the integral of 1 is simply x + C.
2. The Integral of x (∫x dx)
In this case, n = 1. Applying the power rule:
∫x¹ dx = (x¹⁺¹)/(1+1) + C = (x²)/2 + C
The integral of x is (x²)/2 + C.
3. The Integral of x² (∫x² dx)
Here, n = 2. Applying the power rule:
∫x² dx = (x²⁺¹)/(2+1) + C = (x³)/3 + C
The integral of x² is (x³)/3 + C.
4. The Integral of x³ (∫x³ dx)
Finally, with n = 3:
∫x³ dx = (x³⁺¹)/(3+1) + C = (x⁴)/4 + C
The integral of x³ is (x⁴)/4 + C.
Key Takeaways
- The power rule is fundamental to integrating polynomial functions.
- Remember the constant of integration "+C" in every indefinite integral.
- Practice is key to mastering integration. Work through various examples to solidify your understanding.
This article provides a clear and concise explanation of integrating simple polynomial terms. Understanding these basic principles lays the foundation for tackling more complex integration problems encountered in higher-level calculus. Remember to always double-check your work and practice regularly to build your calculus skills.
Latest Posts
Latest Posts
-
Error Stat Of Oracle Sqlnet Log Failed Permission Denied
May 24, 2025
-
Map Of Middle Earth Frodos Journey
May 24, 2025
-
Bright Colors Losing Detail Gimp Coloraize
May 24, 2025
-
Is Momentum Conserved In An Inelastic Collision
May 24, 2025
-
How To Change Recessed Light Bulb
May 24, 2025
Related Post
Thank you for visiting our website which covers about Integral Of 1 X 2 3 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.