What Is A Polynomial In Standard Form
Kalali
Apr 03, 2025 · 5 min read
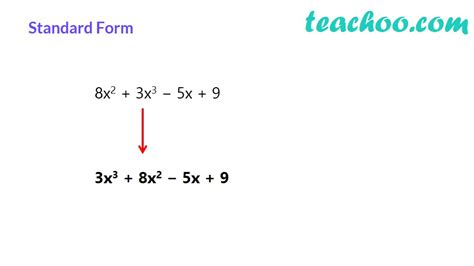
Table of Contents
What is a Polynomial in Standard Form? A Comprehensive Guide
Polynomials are fundamental building blocks in algebra and beyond, forming the basis for many mathematical concepts and applications. Understanding what a polynomial is, particularly in its standard form, is crucial for success in various mathematical fields, from solving equations to advanced calculus. This comprehensive guide will delve into the definition of a polynomial, explain what standard form entails, illustrate how to convert polynomials to standard form, and explore the significance of this form in mathematical operations.
Defining a Polynomial
A polynomial is an expression consisting of variables (often represented by x, y, etc.) and coefficients, that involves only the operations of addition, subtraction, multiplication, and non-negative integer exponents of variables. It's a finite sum of terms, each term being a product of a constant and a non-negative integer power of a variable.
Here's a breakdown:
- Variables: These are symbols representing unknown values (e.g., x, y, z).
- Coefficients: These are the numerical constants multiplying the variables (e.g., 2, -5, 1/2).
- Exponents: These are the non-negative integers indicating the power to which the variable is raised (e.g., 2 in x², 0 in x⁰ = 1).
- Terms: A term is a single product of coefficients and variables raised to powers (e.g., 3x², -2xy, 5).
- Finite Sum: A polynomial is a finite sum of such terms, meaning it doesn't have infinitely many terms.
Examples of Polynomials:
- 3x² + 2x - 5
- 7y⁴ - 2y² + y + 1
- x³ + 5x²y - 2xy² + y³
- 4 (This is a constant polynomial, considered a polynomial of degree 0)
- -2x (This is a linear polynomial)
Examples of Expressions that are NOT Polynomials:
- 1/x (The variable is in the denominator, violating the non-negative integer exponent rule)
- √x (The exponent is 1/2, which is not an integer)
- 2ˣ (The variable is in the exponent)
- x⁻² (Negative exponent)
- 1/x² + 3x - 5 (Has a term with a negative exponent)
Understanding Standard Form of a Polynomial
The standard form of a polynomial is a specific way of writing the polynomial where the terms are arranged in descending order of their degree. The degree of a term is the sum of the exponents of its variables. The degree of a polynomial is the highest degree among its terms.
Let's illustrate this with examples:
Example 1: Consider the polynomial 2x³ - 5x + 3x² + 7.
- The term 2x³ has a degree of 3.
- The term 3x² has a degree of 2.
- The term -5x has a degree of 1.
- The term 7 has a degree of 0 (constant term).
In standard form, this polynomial would be written as: 2x³ + 3x² - 5x + 7
Example 2: Consider the polynomial 5xy² + 3x²y - 2x³ + 4. The degree of each term is calculated as follows:
- 5xy²: degree 3 (1 + 2)
- 3x²y: degree 3 (2 + 1)
- -2x³: degree 3 (3)
- 4: degree 0
In standard form, there might be some flexibility when multiple terms have the same highest degree. However, a common approach would be:
-2x³ + 3x²y + 5xy² + 4 or any other arrangement where the highest-degree terms are first.
Converting Polynomials to Standard Form
To write a polynomial in standard form, follow these steps:
- Identify the terms: Break down the polynomial into its individual terms.
- Calculate the degree of each term: Determine the sum of the exponents of the variables in each term.
- Arrange the terms: Write the terms in descending order of their degrees, starting with the highest degree and ending with the constant term (degree 0).
- Combine like terms: If there are terms with the same variables raised to the same powers, combine their coefficients.
Example: Convert the polynomial 5x - 2x² + 7 + 3x³ to standard form.
- Terms: 5x, -2x², 7, 3x³
- Degrees: 5x (degree 1), -2x² (degree 2), 7 (degree 0), 3x³ (degree 3)
- Arrangement: 3x³ - 2x² + 5x + 7
Significance of Standard Form
The standard form is more than just a neat arrangement; it plays a crucial role in various algebraic operations and applications:
- Easier identification of the degree: The degree of the polynomial is immediately apparent as it's the exponent of the first term. The degree is essential in classifying polynomials (e.g., linear, quadratic, cubic) and understanding their properties.
- Simplified addition and subtraction: Adding and subtracting polynomials in standard form is straightforward, as you can directly combine like terms with the same degree.
- Efficient multiplication: While not directly simplifying multiplication, the standard form can help organize the terms after multiplication, facilitating the collection of like terms.
- Finding roots (solutions) of polynomial equations: Many methods for solving polynomial equations, such as factoring or the quadratic formula (for quadratic polynomials), work more effectively when the polynomial is in standard form.
- Graphing polynomials: The standard form offers insights into the end behavior of the polynomial graph (i.e., the behavior of the graph as x approaches positive or negative infinity). The leading term (the term with the highest degree) dictates the end behavior.
- Polynomial division (long division or synthetic division): Performing polynomial division is significantly easier when both the dividend and divisor are in standard form.
Advanced Concepts and Applications
The importance of standard form extends to more advanced topics:
- Partial Fraction Decomposition: When decomposing rational functions (fractions with polynomials in the numerator and denominator), expressing the polynomials in standard form simplifies the process.
- Numerical Analysis: Many numerical methods used to approximate the roots of polynomials or evaluate polynomial functions rely on the polynomial being in standard form or a readily transformable form.
- Linear Algebra: Polynomials play a role in topics like matrix representations and characteristic equations, where the standard form is implicitly or explicitly used.
- Calculus: Finding derivatives and integrals of polynomials benefits from having them in standard form, facilitating the application of power rules.
Conclusion
The standard form of a polynomial is not merely a stylistic choice; it's a fundamental representation that significantly simplifies mathematical operations, reveals crucial properties, and facilitates the application of polynomials in diverse mathematical contexts. Mastering the concept of standard form and the ability to convert polynomials into this form is essential for anyone pursuing a path involving mathematics or related fields. From basic algebraic manipulations to advanced analytical techniques, the standard form provides a crucial organizational and operational framework for working with polynomials. A firm understanding of this concept is a cornerstone of mathematical proficiency.
Latest Posts
Latest Posts
-
What Are The Least Common Multiples Of 3 And 4
Apr 04, 2025
-
What Percentage Of 30 Is 40
Apr 04, 2025
-
14 Ounces Equals How Many Cups
Apr 04, 2025
-
31 Out Of 35 As A Percentage
Apr 04, 2025
-
How Many Inches Is 120 Centimeters
Apr 04, 2025
Related Post
Thank you for visiting our website which covers about What Is A Polynomial In Standard Form . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
