What Percent Is 20 Out Of 30
Kalali
Apr 04, 2025 · 5 min read
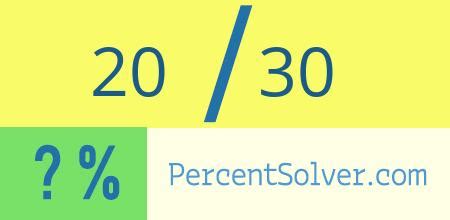
Table of Contents
What Percent is 20 out of 30? A Deep Dive into Percentage Calculations and Applications
Determining what percent 20 is out of 30 might seem like a simple calculation, but understanding the underlying principles can unlock a world of applications in various fields. This comprehensive guide will not only answer this specific question but also delve into the broader context of percentage calculations, exploring different methods, practical examples, and real-world applications.
Understanding Percentages: The Foundation
Percentages are a fundamental concept in mathematics representing a fraction of 100. They're expressed using the "%" symbol, and essentially show a proportion relative to a whole. The whole is always considered 100%. For example, 50% represents 50 out of 100, or one-half.
Calculating "What Percent is 20 out of 30?"
There are several ways to calculate what percentage 20 represents of 30. Let's explore the most common methods:
Method 1: The Fraction Method
This is perhaps the most intuitive approach. We express the problem as a fraction:
20/30
Then, we convert this fraction into a percentage by multiplying it by 100%:
(20/30) * 100% = 66.67% (approximately)
Therefore, 20 is approximately 66.67% of 30.
Method 2: The Proportion Method
This method utilizes the concept of proportions. We set up a proportion:
20/30 = x/100
Where 'x' represents the percentage we're trying to find. Solving for 'x':
x = (20 * 100) / 30 x = 66.67 (approximately)
Again, we find that 20 is approximately 66.67% of 30.
Method 3: Using a Calculator
Most calculators have a percentage function. Simply enter 20 ÷ 30 and then multiply by 100 to obtain the percentage directly. This provides the quickest and most efficient solution for this specific problem.
Beyond the Basics: Understanding the Context
While the calculation itself is straightforward, understanding the context of the problem is crucial for real-world applications. For instance, if we're looking at exam scores, 20 out of 30 might represent a student's performance. In business, it could represent sales figures, production output, or market share. The interpretation changes based on the scenario.
Real-World Applications of Percentage Calculations:
Percentages are ubiquitous in our daily lives and essential across various fields:
-
Finance: Calculating interest rates, loan repayments, discounts, tax rates, profit margins, and investment returns all rely heavily on percentage calculations. Understanding percentage changes is key to analyzing financial performance and making informed decisions. For example, calculating the percentage increase or decrease in stock prices, or understanding compound interest calculations are crucial for investors.
-
Retail and Sales: Discounts, sales tax, markups, and profit margins are all expressed as percentages. Understanding these percentages is crucial for consumers to make informed purchasing decisions and for businesses to set prices strategically. For example, a "20% off" sale means a 20% reduction in the original price.
-
Science and Statistics: Percentages are frequently used to represent data, such as the percentage of a population with a particular characteristic, the success rate of a medical treatment, or the probability of an event occurring. Statistical analysis frequently involves percentage calculations to draw meaningful conclusions from data.
-
Education: Grade calculations, test scores, and performance assessments often use percentages to express student achievement. Understanding these percentages is crucial for students to track their progress and for educators to assess student learning.
-
Everyday Life: We use percentages constantly in everyday situations, from figuring out tips at restaurants to understanding nutritional information on food labels. Understanding percentages empowers us to make informed decisions and manage our finances effectively.
Advanced Percentage Calculations:
Beyond basic percentage calculations, several advanced concepts are frequently used:
-
Percentage Change: This measures the change in a quantity expressed as a percentage of the original value. The formula is: [(New Value - Old Value) / Old Value] * 100%. This is useful for tracking growth or decline over time in various metrics.
-
Percentage Point Difference: This refers to the arithmetic difference between two percentages. For instance, an increase from 20% to 30% is a 10 percentage point increase, not a 50% increase. This distinction is critical in interpreting changes accurately, especially in areas like economic statistics or polling data.
-
Compounding Percentages: This refers to situations where a percentage change is applied repeatedly over time, as often seen in interest calculations. The effect of compounding can be significant, leading to exponential growth or decline. Understanding compound interest is crucial for long-term financial planning and investment strategies.
Solving More Complex Percentage Problems:
Let's look at some more advanced percentage problems to further illustrate the principles discussed:
Problem 1: A shirt is originally priced at $50. It's on sale for 30% off. What's the sale price?
Solution: The discount is 30% of $50, which is (30/100) * $50 = $15. The sale price is $50 - $15 = $35.
Problem 2: A student scores 80% on a test with 40 questions. How many questions did they answer correctly?
Solution: The number of correct answers is 80% of 40, which is (80/100) * 40 = 32 questions.
Problem 3: A company's revenue increased from $100,000 to $120,000. What is the percentage increase?
Solution: The percentage increase is [(120,000 - 100,000) / 100,000] * 100% = 20%.
Conclusion:
Understanding percentages is a fundamental skill with widespread applications. While calculating "what percent is 20 out of 30" may seem trivial, the underlying principles extend far beyond this single calculation. Mastering percentage calculations empowers you to analyze data, make informed decisions, and navigate various aspects of your personal and professional life more effectively. From financial planning to everyday purchases, the ability to confidently work with percentages is invaluable. By understanding the various methods of calculation, the importance of context, and the application of advanced concepts, you can become proficient in this essential mathematical skill.
Latest Posts
Latest Posts
-
What Is 58 Degrees Celsius In Fahrenheit
Apr 05, 2025
-
What Is 6 Percent Of 20
Apr 05, 2025
-
What Is 33 Inches In Feet
Apr 05, 2025
-
How To Know If An Quadratic Equation Is Linear
Apr 05, 2025
-
How Much Is 78 Inches In Feet
Apr 05, 2025
Related Post
Thank you for visiting our website which covers about What Percent Is 20 Out Of 30 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
