What Percentage Is 5 Out Of 20
Kalali
Apr 17, 2025 · 6 min read
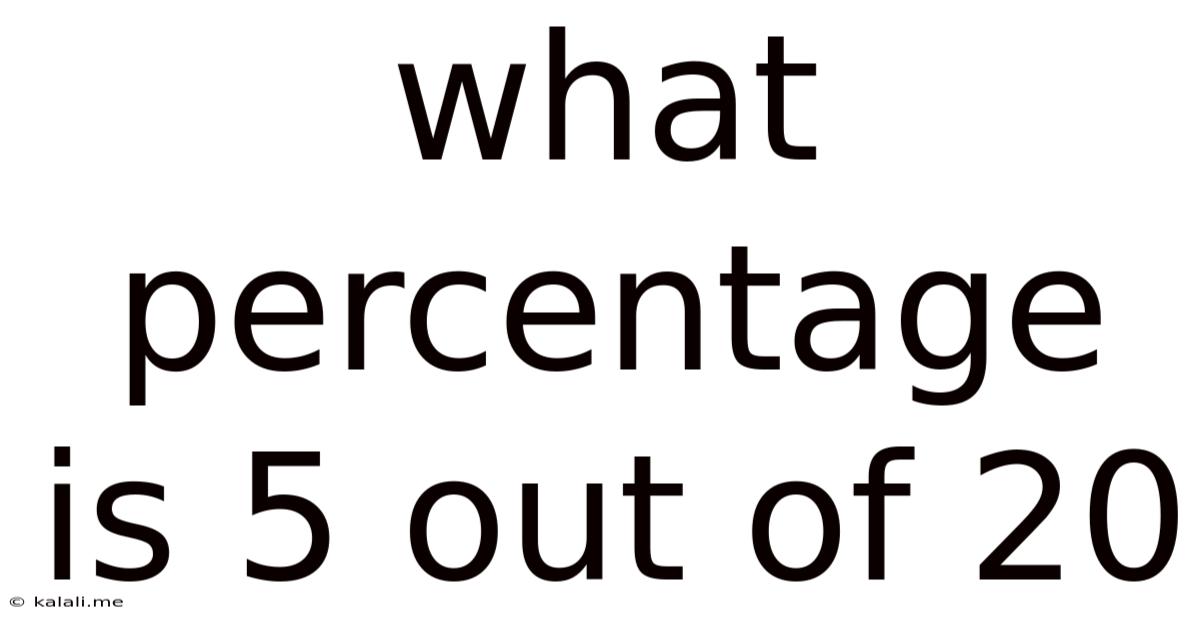
Table of Contents
What Percentage is 5 out of 20? A Deep Dive into Percentages and Their Applications
Meta Description: Learn how to calculate percentages, understand the meaning of "5 out of 20" as a percentage, and explore various real-world applications of percentage calculations. This comprehensive guide covers basic percentage concepts and advanced applications.
Calculating percentages is a fundamental skill with widespread applications across various fields, from everyday budgeting to complex statistical analysis. Understanding how to determine what percentage 5 out of 20 represents is not just about finding a single answer; it's about grasping the underlying principles of percentage calculations and their practical uses. This article will delve into this seemingly simple question, exploring the process, its variations, and its significance in different contexts.
Understanding the Basics of Percentages
A percentage is a fraction or ratio expressed as a number out of 100. The symbol used to represent percentage is "%". It essentially shows the proportion of a part relative to a whole. For instance, 50% means 50 out of 100, or half. This concept is crucial for understanding how to calculate any percentage, including what percentage 5 out of 20 represents.
The fundamental formula for calculating percentages is:
(Part / Whole) x 100% = Percentage
This formula can be applied to any scenario where you need to determine the percentage one value represents of another. In our case, the "part" is 5, and the "whole" is 20.
Calculating the Percentage: 5 out of 20
Let's apply the formula to answer the question, "What percentage is 5 out of 20?"
-
Identify the part and the whole: The part is 5, and the whole is 20.
-
Substitute into the formula: (5 / 20) x 100% = Percentage
-
Calculate the fraction: 5 divided by 20 equals 0.25.
-
Multiply by 100: 0.25 x 100% = 25%
Therefore, 5 out of 20 is 25%.
Different Ways to Calculate Percentages
While the above method is straightforward, there are alternative approaches to calculating percentages, especially helpful when dealing with more complex scenarios or mental calculations.
-
Using Proportions: You can set up a proportion to solve for the percentage. For example:
5/20 = x/100
Cross-multiply: 20x = 500
Solve for x: x = 500/20 = 25
Therefore, x = 25%, confirming our previous result.
-
Simplifying the Fraction: Before multiplying by 100%, simplify the fraction if possible. In this case, 5/20 simplifies to 1/4. This makes the calculation easier: (1/4) x 100% = 25%.
-
Using a Calculator: Calculators provide the most efficient way to calculate percentages, especially with more complicated numbers. Simply divide the part by the whole and multiply by 100.
Real-World Applications of Percentage Calculations
Understanding percentages is essential in numerous real-world situations. Here are some examples:
-
Finance: Calculating interest rates, discounts, tax rates, profit margins, and investment returns all involve percentage calculations. For instance, understanding that a 25% discount on a $100 item means a $25 reduction is crucial for making informed financial decisions.
-
Statistics: Percentages are frequently used to represent data in graphs, charts, and reports. For instance, analyzing survey results, election polls, or economic indicators often involves interpreting percentages. Understanding that 25% of respondents favored a particular candidate offers valuable insight.
-
Academics: Grading systems, test scores, and performance evaluations often use percentages to represent academic achievements. A student scoring 25 out of 100 on a test has achieved a 25% score.
-
Science: In scientific research, percentages are used to express concentrations, error margins, and other quantitative data. For example, a 25% solution of salt in water indicates that 25 grams of salt are dissolved in 100ml of water.
Beyond the Basics: More Complex Percentage Problems
While calculating "5 out of 20" is relatively simple, the principles extend to more complex percentage problems. Here are some examples:
-
Finding the Whole: If you know the percentage and the part, you can calculate the whole. For example, if 25% of a number is 5, what is the whole number? The calculation would be: 5 / 0.25 = 20.
-
Finding the Part: If you know the percentage and the whole, you can calculate the part. For example, what is 25% of 20? The calculation would be: 0.25 x 20 = 5.
-
Percentage Increase/Decrease: This involves calculating the percentage change between two values. For instance, if a price increases from $20 to $25, the percentage increase is calculated as follows: [(25-20)/20] x 100% = 25%. Conversely, a decrease from $25 to $20 represents a 20% decrease.
-
Compound Interest: Compound interest calculations involve applying percentage growth repeatedly over time. Understanding these concepts is crucial for planning long-term investments and savings.
Practical Applications: Examples in Different Fields
Let's explore how the understanding of percentages, as exemplified by calculating 5 out of 20, applies across various fields:
1. Marketing and Sales:
- Conversion Rates: If a website receives 20 visitors and 5 make a purchase, the conversion rate is 25%. This metric is crucial for optimizing marketing campaigns and improving sales strategies.
- Customer Satisfaction: If 5 out of 20 customers express dissatisfaction, a 25% dissatisfaction rate signals a need for improvement in customer service or product quality.
2. Healthcare:
- Success Rates: If a surgical procedure is performed on 20 patients and 5 experience complications, the complication rate is 25%. This data informs improvements in surgical techniques and patient care.
- Vaccination Rates: If 5 out of 20 individuals in a community are unvaccinated, the vaccination rate is 75%, indicating potential vulnerabilities within the population.
3. Education:
- Test Scores: As mentioned earlier, a score of 5 out of 20 represents a 25% grade. This metric helps assess student understanding and the effectiveness of teaching methods.
- Attendance Rates: If 5 out of 20 students are absent from a class, the attendance rate is 75%, potentially signaling issues requiring attention.
Mastering Percentages: Tips and Practice
Becoming proficient in percentage calculations requires consistent practice. Here are some tips:
- Master the Formula: Understand the formula (Part / Whole) x 100% and how to apply it to different situations.
- Practice Regularly: Solve various percentage problems to build your understanding and speed.
- Use Mental Math: Practice mental calculation techniques to improve your speed and accuracy.
- Utilize Online Resources: Several online tools and resources offer practice problems and interactive exercises.
In conclusion, while answering "What percentage is 5 out of 20?" with a simple "25%" is straightforward, the underlying concept of percentages extends far beyond this basic calculation. Understanding percentage calculations is a fundamental skill applicable to various aspects of life, making it a valuable asset in both personal and professional settings. By grasping the core principles and practicing regularly, you can confidently navigate numerous scenarios involving percentages and make informed decisions based on quantitative data.
Latest Posts
Latest Posts
-
What Percent Is 14 Out Of 20
Apr 19, 2025
-
What Is 3 Percent Of 10000
Apr 19, 2025
-
4 Liters Is How Many Milliliters
Apr 19, 2025
-
Cuanto Es 30 Grados Centigrados En Fahrenheit
Apr 19, 2025
-
22 15 As A Mixed Number
Apr 19, 2025
Related Post
Thank you for visiting our website which covers about What Percentage Is 5 Out Of 20 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.