16 Over 5 As A Mixed Number
Kalali
Apr 20, 2025 · 5 min read
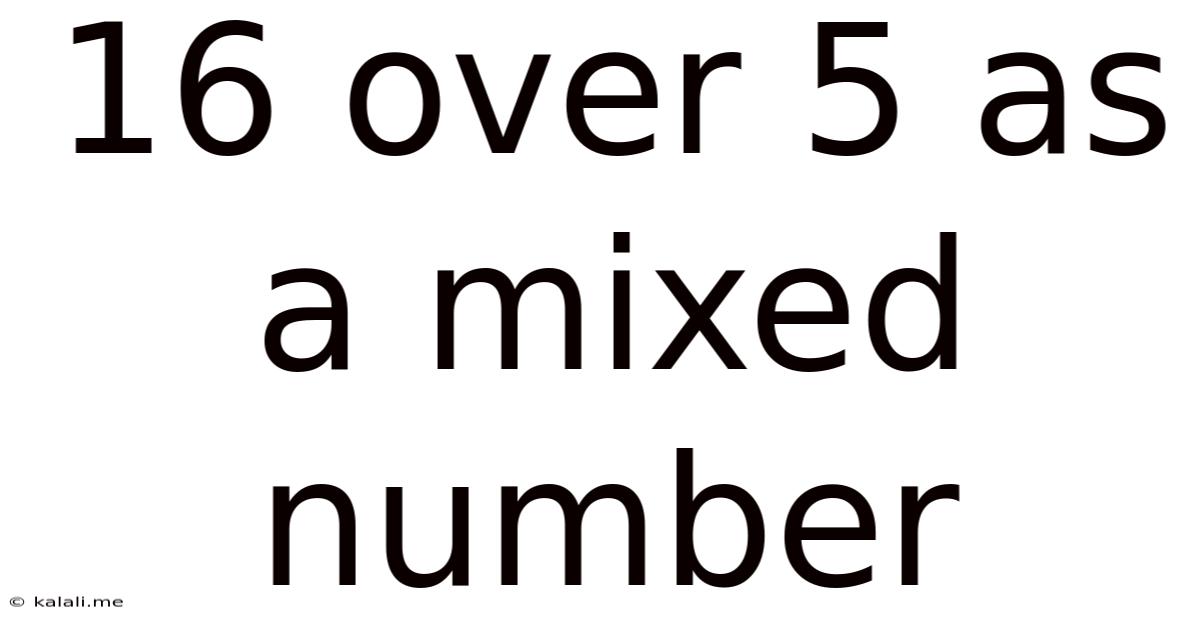
Table of Contents
16 Over 5 as a Mixed Number: A Comprehensive Guide
Understanding fractions and how to convert them into mixed numbers is a fundamental skill in mathematics. This comprehensive guide will delve into the process of converting the improper fraction 16/5 into a mixed number, exploring the underlying concepts and offering practical examples to solidify your understanding. We'll cover not just the mechanics of the conversion but also the broader context of fractions and their applications.
Meta Description: Learn how to convert the improper fraction 16/5 into a mixed number. This detailed guide explains the process, explores related concepts, and provides practical examples for a thorough understanding.
Understanding Fractions and Mixed Numbers
Before diving into the conversion of 16/5, let's refresh our understanding of fractions and mixed numbers. A fraction represents a part of a whole. It consists of two numbers: the numerator (the top number) and the denominator (the bottom number). The denominator indicates how many equal parts the whole is divided into, while the numerator indicates how many of those parts are being considered.
An improper fraction is a fraction where the numerator is greater than or equal to the denominator (e.g., 16/5, 7/4, 9/9). This indicates that the fraction represents a value greater than or equal to one.
A mixed number combines a whole number and a proper fraction (e.g., 3 ¼, 2 ⅔, 1 ⅛). It represents a value greater than one, expressing the whole number part separately from the fractional part. Mixed numbers are often preferred for representing quantities in real-world situations because they provide a more intuitive understanding of the magnitude.
Converting 16/5 to a Mixed Number: The Process
The conversion of an improper fraction like 16/5 to a mixed number involves dividing the numerator by the denominator. Here's a step-by-step breakdown:
-
Divide the numerator by the denominator: Divide 16 by 5.
16 ÷ 5 = 3 with a remainder of 1.
-
The quotient becomes the whole number part: The quotient of the division (3) becomes the whole number part of the mixed number.
-
The remainder becomes the numerator of the fractional part: The remainder of the division (1) becomes the numerator of the fractional part.
-
The denominator remains the same: The denominator of the original improper fraction (5) remains the same in the fractional part of the mixed number.
Therefore, 16/5 as a mixed number is 3 1/5.
Visual Representation
Imagine you have 16 slices of pizza, and each pizza has 5 slices. You can make 3 whole pizzas (3 x 5 = 15 slices) and have 1 slice left over. This visually represents the mixed number 3 1/5. This visual approach can be helpful, especially when explaining the concept to younger learners.
Practical Applications
Converting improper fractions to mixed numbers is crucial in numerous real-world applications:
-
Measurement: Imagine you're measuring ingredients for a recipe. If a recipe calls for 16/5 cups of flour, converting it to 3 1/5 cups makes it much easier to measure using standard measuring cups.
-
Construction: In construction projects, measurements are often expressed in fractions and mixed numbers. Converting between these forms ensures accurate calculations and avoids errors.
-
Data Analysis: When dealing with data analysis, representing data as mixed numbers can provide a clearer picture and easier interpretation than using only improper fractions.
-
Everyday Calculations: Many everyday scenarios involve fractions, from splitting bills to calculating distances. Understanding how to convert improper fractions to mixed numbers is essential for accurate and efficient calculations.
Working with Mixed Numbers: Addition and Subtraction
Once you've converted an improper fraction to a mixed number, you can perform various arithmetic operations. Let's look at addition and subtraction as examples:
Addition: To add mixed numbers, you add the whole number parts separately and then the fractional parts separately. If the sum of the fractional parts is an improper fraction, convert it to a mixed number and add it to the whole number sum.
Example: Add 3 1/5 + 2 2/5
- Add the whole numbers: 3 + 2 = 5
- Add the fractions: 1/5 + 2/5 = 3/5
- Combine: 5 + 3/5 = 5 3/5
Subtraction: Similar to addition, subtract the whole number parts and then the fractional parts separately. If the fractional part of the subtrahend is larger than the minuend, you'll need to borrow from the whole number part.
Example: Subtract 3 1/5 - 1 3/5
- Since 1/5 < 3/5, we borrow 1 from the whole number part of 3 1/5. This gives us 2 6/5 (because 1 = 5/5, and 5/5 + 1/5 = 6/5).
- Subtract the whole numbers: 2 - 1 = 1
- Subtract the fractions: 6/5 - 3/5 = 3/5
- Combine: 1 + 3/5 = 1 3/5
Equivalent Fractions
Remember that many fractions can represent the same value. For instance, 2/4, 3/6, and 4/8 are all equivalent to ½. This concept is vital when performing operations with fractions. When adding or subtracting fractions, ensure they have a common denominator.
Beyond 16/5: Generalizing the Conversion Process
The method for converting 16/5 to a mixed number applies to any improper fraction. The core process remains the same: divide the numerator by the denominator, use the quotient as the whole number, and the remainder as the numerator of the fractional part. The denominator remains unchanged.
Using a Calculator
While the manual process enhances understanding, calculators can quickly convert improper fractions to mixed numbers. Most calculators have a function to handle fractions directly. However, understanding the manual process is crucial for problem-solving and building a strong mathematical foundation.
Conclusion
Converting 16/5 to the mixed number 3 1/5 is a fundamental skill in arithmetic. This guide has explored the process in detail, providing a comprehensive understanding not only of the conversion itself but also its practical applications and the broader context of fractions and mixed numbers. Mastering this skill is essential for success in mathematics and various real-world applications, from cooking to construction and beyond. Remember to practice regularly to build proficiency and confidence in handling fractions.
Latest Posts
Latest Posts
-
What Percentage Is 15 Out Of 18
Apr 22, 2025
-
What Percentage Of 30 Is 6
Apr 22, 2025
-
How Tall Is 60 Cm In Feet
Apr 22, 2025
-
Cuanto Es 1 7 Oz En Ml
Apr 22, 2025
-
What Percent Of 30 Is 75
Apr 22, 2025
Related Post
Thank you for visiting our website which covers about 16 Over 5 As A Mixed Number . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.