What Is 4/7 As A Percentage
Kalali
Apr 26, 2025 · 5 min read
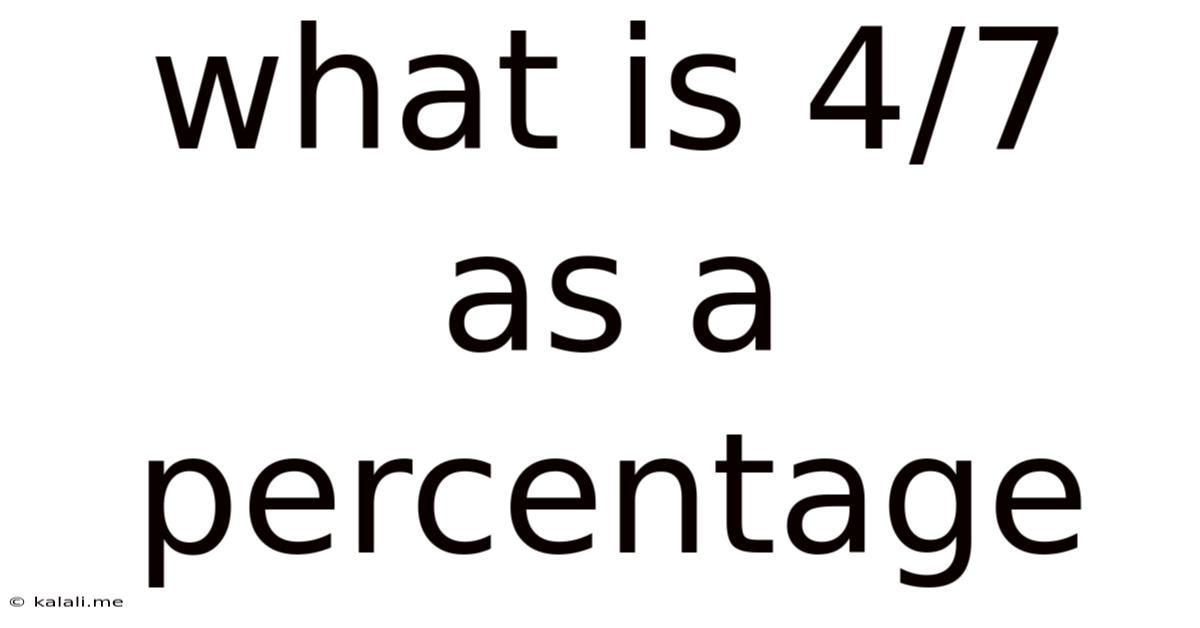
Table of Contents
What is 4/7 as a Percentage? A Comprehensive Guide to Fraction-to-Percentage Conversion
This article will delve deep into the conversion of the fraction 4/7 into a percentage, providing a step-by-step explanation accessible to all levels of mathematical understanding. We'll explore the underlying principles, various calculation methods, and practical applications of this conversion. Understanding fraction-to-percentage conversion is crucial in various fields, from everyday budgeting and cooking to advanced statistical analysis and financial modeling. This comprehensive guide will equip you with the knowledge and skills to tackle similar conversions with confidence.
Understanding Fractions and Percentages
Before diving into the conversion of 4/7, let's refresh our understanding of fractions and percentages. A fraction represents a part of a whole, expressed as a ratio of two numbers: the numerator (top number) and the denominator (bottom number). For example, in the fraction 4/7, 4 is the numerator and 7 is the denominator. This means we have 4 parts out of a total of 7 parts.
A percentage, on the other hand, is a way of expressing a number as a fraction of 100. It represents a proportion per hundred. The symbol "%" is used to denote percentage. For instance, 50% means 50 out of 100, or 50/100, which simplifies to 1/2.
Method 1: Direct Conversion using Division
The most straightforward method to convert a fraction to a percentage involves dividing the numerator by the denominator and then multiplying the result by 100. Let's apply this to 4/7:
-
Divide the numerator by the denominator: 4 ÷ 7 ≈ 0.5714
-
Multiply the result by 100: 0.5714 × 100 ≈ 57.14
Therefore, 4/7 is approximately 57.14%.
Understanding Decimal Precision
The result, 57.14%, is an approximation. The decimal representation of 4/7 (0.57142857...) is a recurring decimal, meaning the digits repeat infinitely. We rounded the decimal to four decimal places (0.5714) before multiplying by 100. The level of precision required depends on the context. For many applications, two decimal places (57.14%) are sufficient. For others, you might need more decimal places for greater accuracy.
Method 2: Conversion using Equivalent Fractions
An alternative method involves finding an equivalent fraction with a denominator of 100. While this isn't always practical, it provides a deeper understanding of the underlying concept. Unfortunately, there's no easy way to directly convert 7 to 100 through simple multiplication. This method is more suitable for fractions with denominators that are factors of 100 (like 2, 4, 5, 10, 20, 25, 50).
Let's explore a similar fraction where this approach is simpler: converting 2/5 to a percentage. We can multiply both the numerator and the denominator by 20 to get an equivalent fraction with a denominator of 100:
(2 × 20) / (5 × 20) = 40/100 = 40%
This method clearly illustrates the relationship between fractions and percentages.
Method 3: Using a Calculator
Most calculators have a percentage function that simplifies the conversion process. Simply divide the numerator by the denominator and then use the percentage function (often represented by a "%" button) to obtain the percentage directly. This method is quick and efficient, especially for more complex fractions.
Practical Applications of Fraction-to-Percentage Conversions
The ability to convert fractions to percentages is valuable across numerous disciplines:
-
Finance: Calculating interest rates, discounts, profit margins, and investment returns often requires converting fractions to percentages. For example, understanding a 4/7 increase in investment value is much clearer when expressed as a percentage increase of approximately 57.14%.
-
Statistics: Representing data in percentage form makes comparisons and interpretations easier. Converting fractions representing proportions within a sample to percentages allows for clear visualization and analysis.
-
Everyday Life: From calculating sales tax to determining the proportion of ingredients in a recipe, understanding fractions and percentages is essential for daily life calculations. Suppose a recipe calls for 4/7 of a cup of flour; knowing this translates to approximately 57.14% of a cup helps with accurate measuring.
-
Science and Engineering: Expressing experimental results and data using percentages simplifies the communication of findings and facilitates comparisons.
-
Education: Converting fractions to percentages is a fundamental skill taught in elementary and secondary education, forming a basis for more advanced mathematical concepts.
Addressing Common Mistakes and Challenges
-
Rounding Errors: As demonstrated with 4/7, rounding decimals can introduce minor inaccuracies. The degree of accuracy required depends heavily on the context of the calculation.
-
Incorrect Order of Operations: When using the direct division method, ensure you divide the numerator by the denominator before multiplying by 100.
-
Misunderstanding of Percentages: Remember that percentages are always relative to 100. Avoid common mistakes like adding percentages directly without considering their underlying values.
Beyond 4/7: Expanding your Skills
The techniques discussed above can be applied to converting any fraction to a percentage. Regardless of the complexity of the fraction, the fundamental principles remain consistent: divide the numerator by the denominator and multiply by 100. Practice with various fractions, gradually increasing their difficulty, will enhance your proficiency in this essential mathematical skill. Consider practicing with fractions like 3/8, 5/12, or even more complex fractions with larger numerators and denominators. This continuous practice will reinforce your understanding and improve your calculation speed and accuracy.
Conclusion:
Converting 4/7 to a percentage, approximately 57.14%, is a simple yet essential mathematical operation. This article has explored the underlying concepts, detailed several calculation methods, and highlighted the practical applications of fraction-to-percentage conversions. By mastering this fundamental skill, you'll enhance your mathematical abilities and improve your efficiency in various fields. Remember to choose the method most comfortable and efficient for your needs, keeping in mind the level of precision required for your specific application. Practice regularly to build confidence and accuracy in your calculations.
Latest Posts
Latest Posts
-
10 Out Of 17 As A Percentage
Apr 27, 2025
-
100 Is What Percent Of 500
Apr 27, 2025
-
Does 4 Cups Equal A Quart
Apr 27, 2025
-
3 3 Cm Is How Many Inches
Apr 27, 2025
-
What Percent Of 12 Is 5
Apr 27, 2025
Related Post
Thank you for visiting our website which covers about What Is 4/7 As A Percentage . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.